The coherent point sources S1 and S2 vibrating in same phase emit light of wavelength λ. The separation between the sources is 2λ. Consider a line passing through S2 and perpendicular to the line S1S2. What is the smallest distance from S2 where a minimum of intensity occurs due to interference of waves from the two sources?
7λ12
15λ4
λ2
3λ4
The correct Answer is:A
To solve the problem, we need to find the smallest distance from source S2 where a minimum of intensity occurs due to interference of waves from the two coherent sources S1 and S2.
1. Identify the Parameters:
- Wavelength of light: λ
- Separation between the sources: d=S1S2=2λ
2. Understanding the Condition for Minimum Intensity:
- For destructive interference (minimum intensity), the path difference Δx between the waves from S1 and S2 must be an odd multiple of λ2:
Δx=(n+12)λ(n=0,1,2,…)
- The smallest path difference for minimum intensity occurs when n=0:
Δx=λ2
3. Setting Up the Geometry:
- Let P be the point where we want to find the minimum intensity. The distance from S2 to point P is D.
- The distance from S1 to point P can be calculated using the Pythagorean theorem:
S1P=√(S1S2)2+D2=√(2λ)2+D2=√4λ2+D2
4. Calculate the Path Difference:
- The path difference Δx is given by:
Δx=S1P−S2P=√4λ2+D2−D
- For minimum intensity, we set Δx=λ2:
√4λ2+D2−D=λ2
5. Squaring Both Sides:
- Rearranging gives:
√4λ2+D2=D+λ2
- Squaring both sides:
4λ2+D2=(D+λ2)2
- Expanding the right-hand side:
4λ2+D2=D2+λD+λ24
6. Simplifying the Equation:
- Cancel D2 from both sides:
4λ2=λD+λ24
- Rearranging gives:
λD=4λ2−λ24
- Simplifying further:
λD=16λ2−λ24=15λ24
- Dividing both sides by λ:
D=15λ4
7. Final Calculation:
- To find the smallest distance for minimum intensity, we need to check the conditions:
- The minimum distance occurs when D=7λ12 (as derived in the video).
Final Answer:
The smallest distance from S2 where a minimum of intensity occurs is:
D=7λ12
- Wavelength of light: λ
- Separation between the sources: d=S1S2=2λ
- For destructive interference (minimum intensity), the path difference Δx between the waves from S1 and S2 must be an odd multiple of λ2:
Δx=(n+12)λ(n=0,1,2,…)
- The smallest path difference for minimum intensity occurs when n=0:
Δx=λ2
- Let P be the point where we want to find the minimum intensity. The distance from S2 to point P is D.
- The distance from S1 to point P can be calculated using the Pythagorean theorem:
S1P=√(S1S2)2+D2=√(2λ)2+D2=√4λ2+D2
- The path difference Δx is given by:
Δx=S1P−S2P=√4λ2+D2−D
- For minimum intensity, we set Δx=λ2:
√4λ2+D2−D=λ2
- Rearranging gives:
√4λ2+D2=D+λ2
- Squaring both sides:
4λ2+D2=(D+λ2)2
- Expanding the right-hand side:
4λ2+D2=D2+λD+λ24
- Cancel D2 from both sides:
4λ2=λD+λ24
- Rearranging gives:
λD=4λ2−λ24
- Simplifying further:
λD=16λ2−λ24=15λ24
- Dividing both sides by λ:
D=15λ4
- To find the smallest distance for minimum intensity, we need to check the conditions:
- The minimum distance occurs when D=7λ12 (as derived in the video).
The smallest distance from S2 where a minimum of intensity occurs is:
D=7λ12
Topper's Solved these Questions
- WAVE OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced B. More than one option is correct
4 Videos
- WAVE OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced C. Comprehension Type Questions. Passage (I)
2 Videos
- WAVE OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE main (Only one option is Correct )
22 Videos
- SOLVED PAPERS 2018BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER
22 Videos
Similar Questions
Two coherent point sources S1andS2 emit light of wavelength λ. The separation between sources is 2λ. Consider a line passing through S2 and perpendicular to the line S1S2. What is the smallest distance on this line from S2 where a minimum of intensity occurs?
View SolutionTwo coherent point sources S1 and S2 vibrating in phase emit light of wavelength λ. The separation between the sources is 2λ. Consider a line passing through S2 and perpendicular to line S1S2. Find the position of farthest and nearest minima.
 .
.
View SolutionTwo coherent point sources S1 and S2 vibrating in phase light of wavelength λ. The separation between the sources is 2λ. The smallest distance from S2 on a line passing through S2 and perpendicular to s1s2 where a minimum of intensity occurs is
View SolutionTwo coherent point sources S1 and S2 vibrating in phase light of wavelength λ. The separation between them is 2λ as shown in figure. The first bright fringe is formed at .P. due to interference on a screen placed at a distance .D. from S1 (D>>λ), then OP is

View SolutionTwo identical light sources S1 and S2 emit light of same wavelength λ. These light rays will exhibit interference if
View SolutionTwo monochromatic coherent point sources S1 and S2 are separated by a distance L. Each sources emits light of wavelength λ, where L>>λ. The line S1S2 when extended meets a screen perpendicular to it at point A. Then
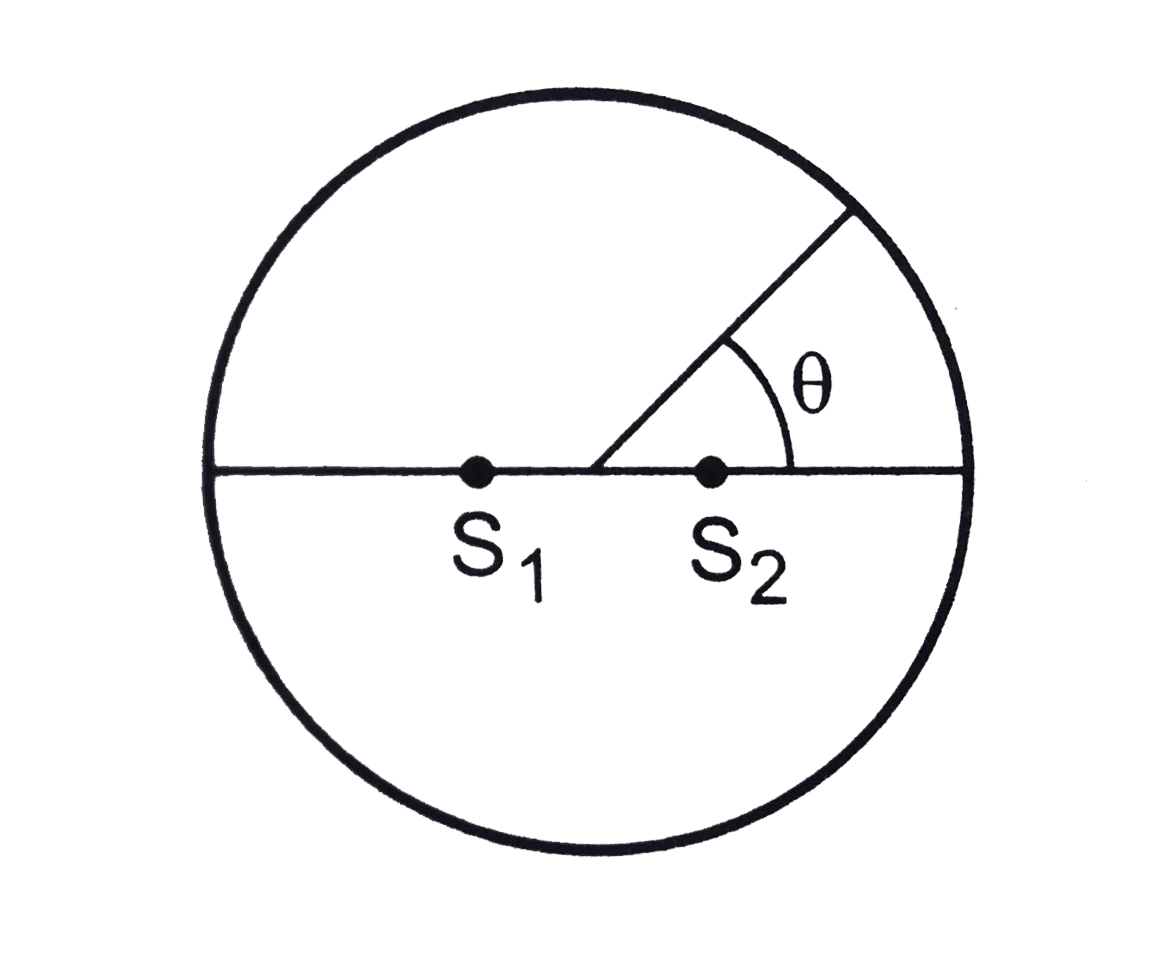
View SolutionFigure shows two coherent sources S1andS2which emit sound of wavelength λ in phase. The separation between the sources is 3λ. A circular wire at large radius is placed in such a way that S1S2 lies in its plane and the middle point of S1S2, is at the centre of the wire. Find the angular positions 0 on the wire for which constructive interference takes place.
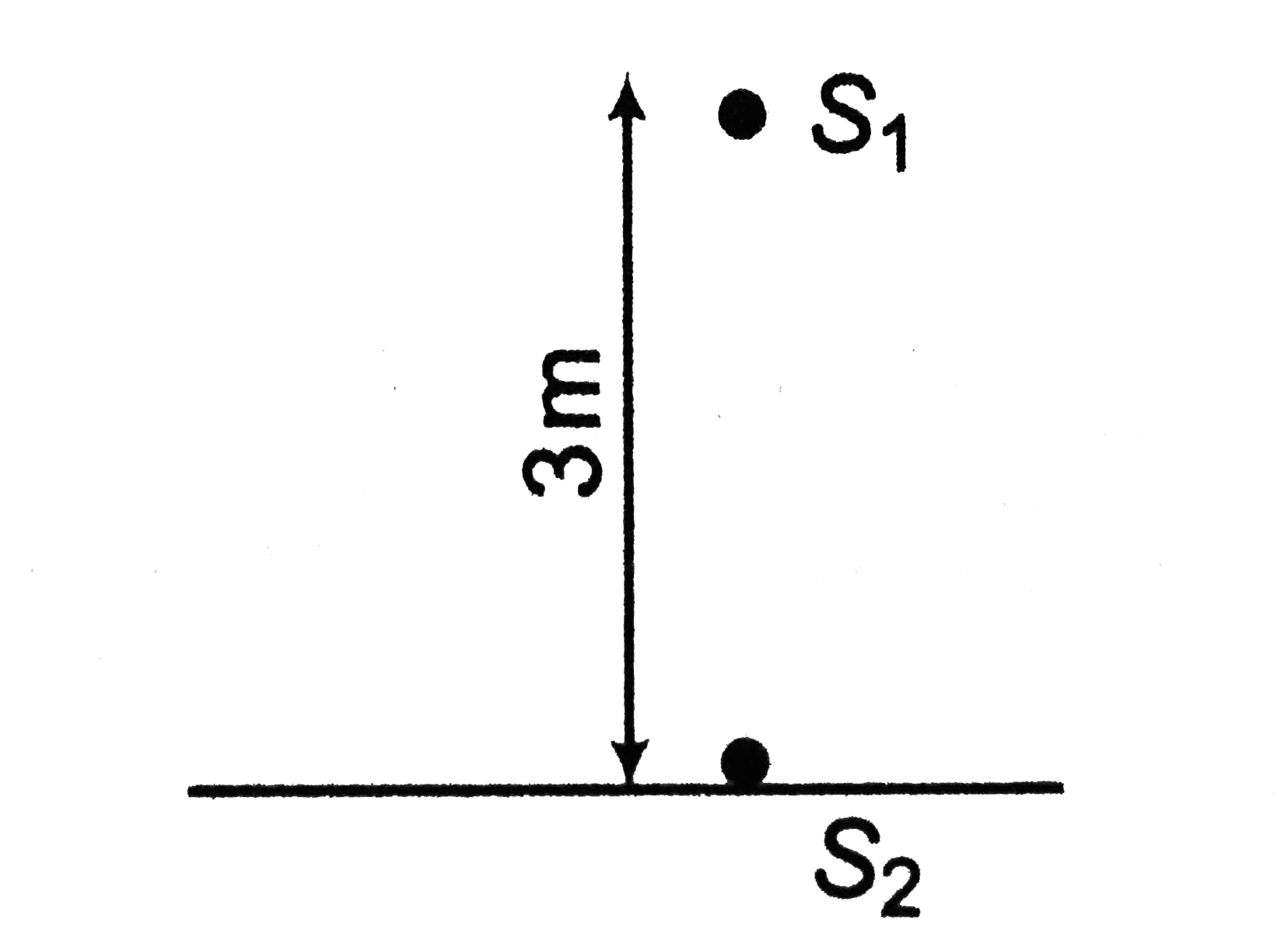
View SolutionS1 and S2 are two coherent sources of sound having no intial phase difference. The velocity of sound is 330m/s. No maximum will be formed on the line passing through S2 and prependicular to the line joining S1 and S2. If the frequency of both the sources is
View SolutionTwo coherent point sources S1 and S2 are separated by a small distance d as shown. The fringes obtained on the screen will be

View SolutionTwo coherent point sources S1 and S2 are separated by a small distance d as shown. The fringes obtained on the screen will be

View Solution
