The electric potential decreases unifromly from 120 V to 80 V as one moves on the x-axis from x=-1 cm to x=+1 cm. The electric field at the origin
must be equal to 20 Vcm^(−1)
may be equal to 20 Vcm^(−1)
may be greater than 20 Vcm(−1)
may be less than 20Vcm(−1)
To find the electric field at the origin given the uniform decrease in electric potential, we can follow these steps:
1. Identify the change in electric potential (ΔV):
The potential decreases from 120 V to 80 V. Therefore, the change in potential is:
ΔV=Vfinal−Vinitial=80V−120V=−40V
2. Identify the change in position (ΔR):
The change in position is from x=−1cm to x=+1cm. The distance moved is:
ΔR=xfinal−xinitial=1cm−(−1cm)=2cm=0.02m
3. Calculate the electric field (E):
The electric field is defined as the negative gradient of the electric potential, which can be calculated using the formula:
E=−ΔVΔR
Substituting the values we have:
E=−−40V0.02m=40V0.02m=2000V/m
4. Convert the electric field to Newtons per Coulomb:
Since 1V/m=1N/C, we can say:
E=2000N/C
5. Direction of the electric field:
The electric field points in the direction of decreasing potential. Since the potential decreases from 120 V to 80 V as we move from -1 cm to +1 cm, the electric field at the origin (0 cm) will point from the higher potential (120 V) towards the lower potential (80 V), which is in the positive x-direction.
Final Answer:
The electric field at the origin is:
E=2000N/Cin the positive x-direction.
The potential decreases from 120 V to 80 V. Therefore, the change in potential is:
ΔV=Vfinal−Vinitial=80V−120V=−40V
The change in position is from x=−1cm to x=+1cm. The distance moved is:
ΔR=xfinal−xinitial=1cm−(−1cm)=2cm=0.02m
The electric field is defined as the negative gradient of the electric potential, which can be calculated using the formula:
E=−ΔVΔR
Substituting the values we have:
E=−−40V0.02m=40V0.02m=2000V/m
Since 1V/m=1N/C, we can say:
E=2000N/C
The electric field points in the direction of decreasing potential. Since the potential decreases from 120 V to 80 V as we move from -1 cm to +1 cm, the electric field at the origin (0 cm) will point from the higher potential (120 V) towards the lower potential (80 V), which is in the positive x-direction.
The electric field at the origin is:
E=2000N/Cin the positive x-direction.
Topper's Solved these Questions
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 1
9 Videos
- ELECTRIC CURRENT THROUGH GASESBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Short answer
6 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Questions for short answer
9 Videos
Similar Questions
The electric potential decreases uniformly from 180V to 20V as one moves on the X-axis from x=−2cm to x=+2cm. The electric field at the origin:
View SolutionThe electric potential decreases uniformly from 100V to 50V as one moves along the x-axis from x=0 to x=5m. The electric field at x=2m must be equal to 10V/m. Is this statement true or false.
View SolutionIf on the x - axis electric potential decreases uniform from 60 V to 20 V between x = -2 m to x = +2 m then the magnitude of electric field at the origin
View SolutionThe electric potential decreases uniformly from V to -V along X-axis in a coordinate system as we moves from a point (−x0,0) to (x0,0), then the electric field at the origin:
View SolutionThe electric potential V at any point (x,y,z), all in meters in space is given by V=4x2 volt. The electric field at the point (1,0,2) in volt//meter is
View SolutionThe electric potential V at any point x, y, z (all in meters) in space is given by V=4x2 volts. The electric field at the point (1m, 0, 2m) is……………..V/m.
View SolutionThe electric potential V at any point x,y,z (all in metre) in space is given by V=4x2 volt. The electric field at the point (1m,0,2m) is ……………Vm.
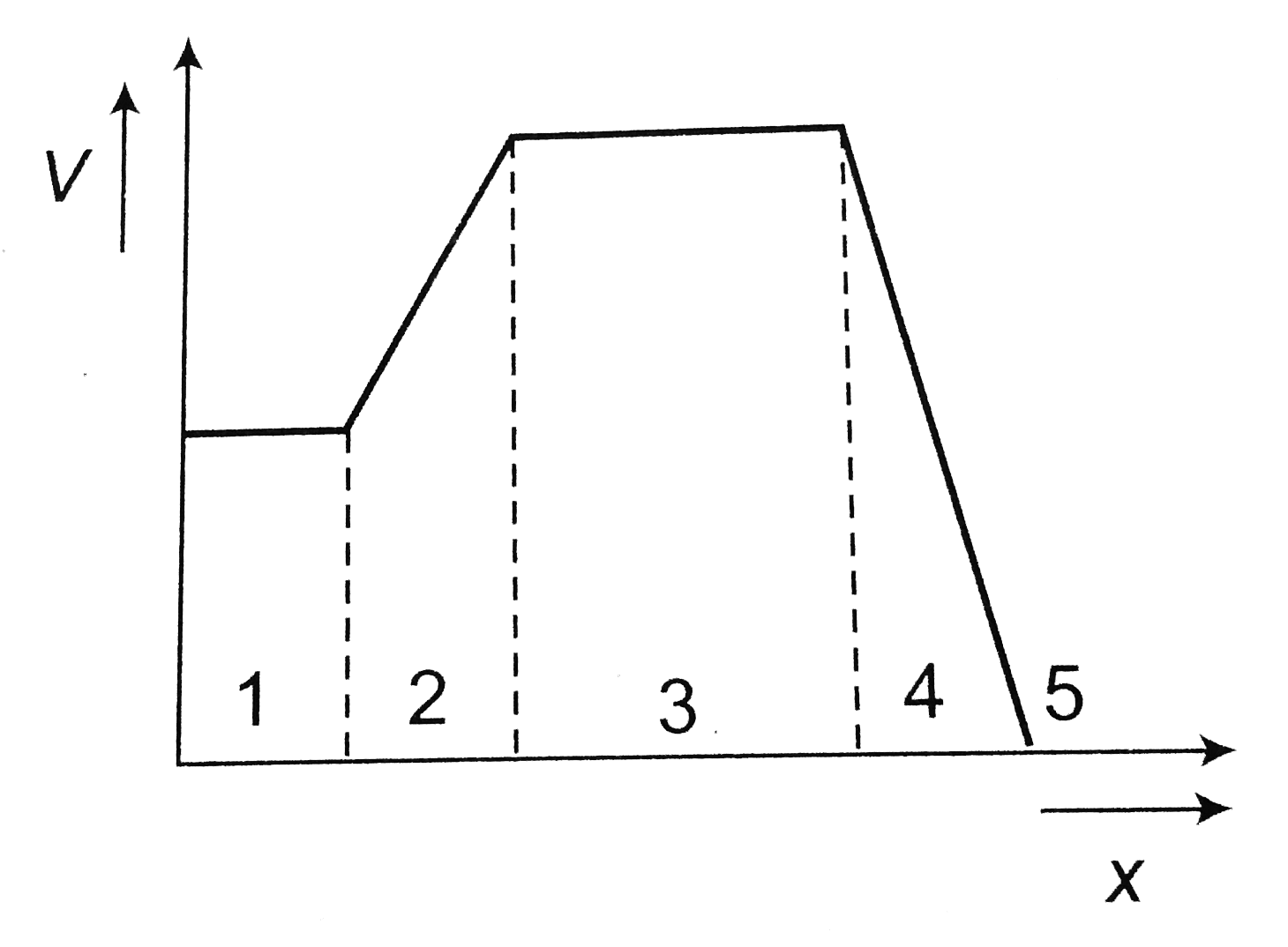
View SolutionThe figure gives the electric potential V as a function of distance through five regions on x-axis. Which of the following is true for the electric E in these regions ?
View SolutionThe electric potential V is givne as a function of distance x (metre) by V=(5x2+10x−9) volt. Value of electric field at x=1is
View SolutionThe electric potential V is givne as a function of distance x (metre) by V=(5x2+10x−9) volt. Value of electric field at x=1is
View Solution
