The luminous intensity of a small plane source of light along the forward normal is 160 candela. Assume the source to be perfectly diffused, find the luminous flux emitted into a cone of solid angle 0.02 sr around a line making an angle of 60∘ with the forward normal.

The situation is shown in ure. By Lambert's cosine law, intensity in the direction SB is
I=I0cos60∘
Where eI0=160 candela is the intensity along the forward normal
ThusI=(160candela)(12)
=80 candela.
The luminous flux emitted in the cone shown in the ure is
△f=I△ω
=(80candela)(0.02sr)
=1.6 lumen.
Topper's Solved these Questions
- PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYBOOK - HC VERMA ENGLISHCHAPTER - PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYEXERCISE - question for short answer
13 Videos
- SEMICONDUCTOR AND SEMICONDUCTOR DEVICESBOOK - HC VERMA ENGLISHCHAPTER - SEMICONDUCTOR AND SEMICONDUCTOR DEVICESEXERCISE - Question for short answer
11 Videos
Similar Questions
In the given figure rasy incident on an interface would converge 10cm below the interface if they continues to move in straight lines without bending. But due to refraction, the rays will bend and meet some where else. Find the distance of meeting point of refracted rasy below the interface, assuming the rays to be making small angles with the normal to the interface.
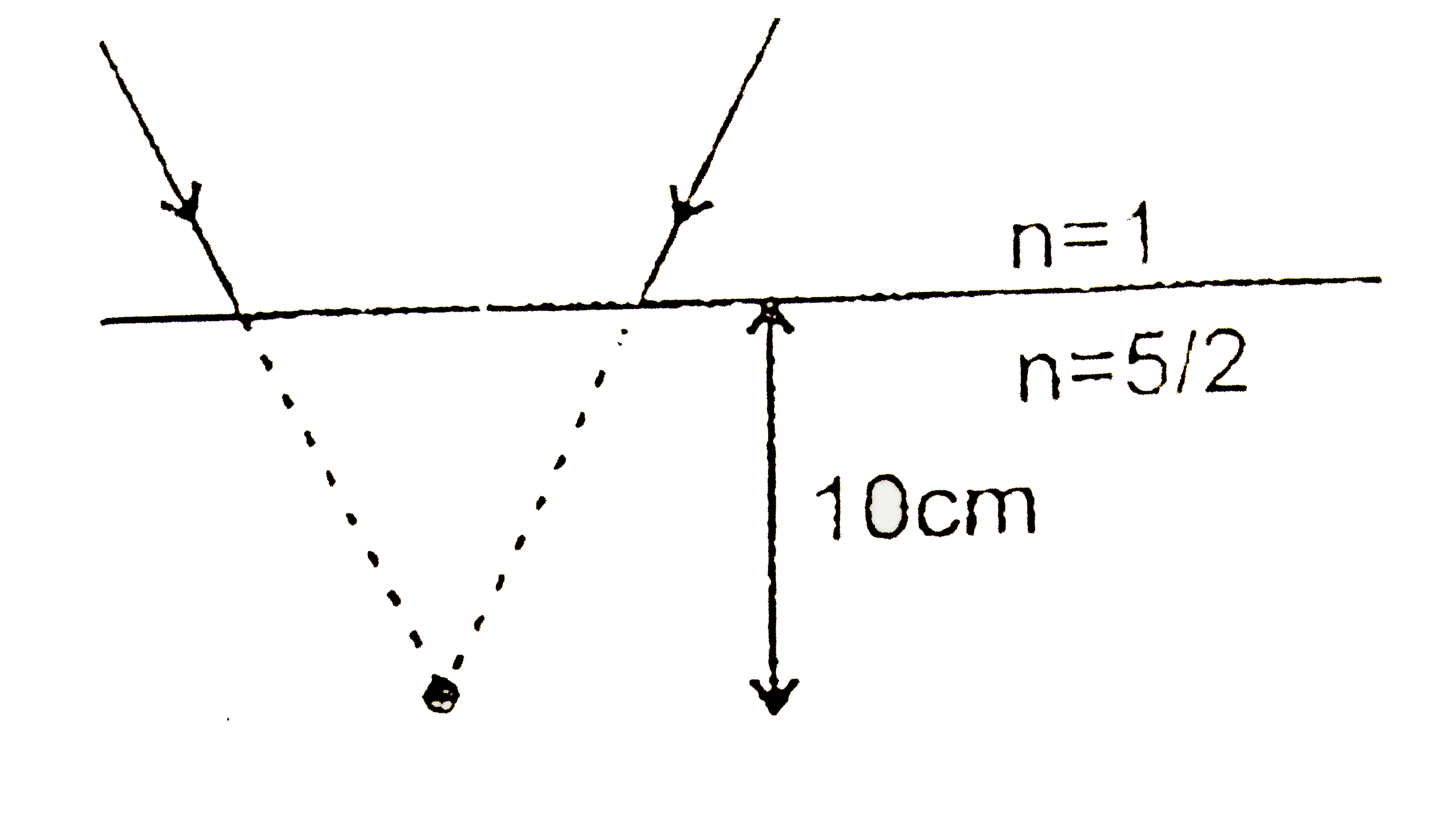
View SolutionA circular area of radius 1.0 cm is placed at a distance of 2.0 m from a poit source.Teh source emits light uniformluy in al directions. The line joining the sorce to the dcentre of the area is normal to the area. It ils found that 2.0×1−3 lumen of luminous flux is incident on the area. Calculate the total luminous flux emitted by the soure and teh luminous intensity of the source along the axis of the area.
View Solution
Knowledge Check
In the question number 66, the flux through the same square if the line normal to its plane makes a 60∘ angle with the x-axis is
A30NC−1m2B10NC−1m2C20NC−1m2D25NC−1m2
A large plane sheet of charge having surface charge density 5×10−16cm−2 lies in XY plane. Find electric flux through a circular area of radius 1cm Given normal to the circular area makes an angle of 60∘ with Z-axis.
View SolutionThe overall luminous efficient of a 100 W electric lamp is 25 lumen W−1. Assume that light is emitted by the lamp only in the forward half, and is uniformly distributed in al diredctionsin this half. Calculate the luminous flux falling on a plane object of area 1cm2 placed at a distance of 50 cm from the lamp and perpendicular to the line joining the lamp and the object.
View SolutionA large plane charge sheet having surface xharge density σ=2.0×10−6Cm−2 lies in the x-y plane. Find the flux of the electric field through a circular area of radius 1 cm lying completely in the region where x, y, z are all positive and with its normal making an angle of 600 with the z-axis.
View SolutionA beam of light from a source L is incident normally on a plane mirror fixed at a certain distance x from the source. The beam is reflected back as a spot on a scale placed just above the source L. When the mirror is rotated through a small angle θ the spot of the light is found to move through a distance y on the scale. The angle θ is given by
View SolutionA narrow beam of monochromatic light of wavelength λ emitted from a source of power P is propogating in the positive x-direction. After being reflected from a perfectly reflecting plane mirror of area vector →A=A(−ˆi−ˆj), the beam falls on a metal plate of surface area A[→A is along outward normal at mirror]. the force exerted by light beam on the mirror is →F and Δ→p is change in momentum of each photon then [cis speed of light in vacuum]
View SolutionOn a frictionless horizontal surface , assumed to be the x−y plane , a small trolley A is moving along a straight line parallel to the y−axis ( see figure) with a constant velocity of (√3−1)m/s . At a particular instant , when the line OA makes an angle of 45(∘) with the x− axis , a ball is thrown along the surface from the origin O. Its velocity makes an angle ϕ with the x− axis and it hits the trolley .
(a) The motion of the ball is observed from the frame of the trolley . Calculate the angle θ made by the velocity vector of the ball with the x−axis in this frame .
(b) Find the speed of the ball with respect to the surface , if ϕ=(4θ)/(3).

View SolutionOn a frictionless horizontal surface , assumed to be the x−y plane , a small trolley A is moving along a straight line parallel to the y-axis( see figure) with a constant velocity of (√3−1)m/s . At a particular instant , when the line OA makes an angle of 45(∘) with the x - axis , a ball is thrown along the surface from the origin O. Its velocity makes an angle ϕ with the x -axis and it hits the trolley .
(a) The motion of the ball is observed from the frame of the trolley . Calculate the angle θ made by the velocity vector of the ball with the x-axis in this frame .
(b) Find the speed of the ball with respect to the surface , if ϕ=(4θ)/(3).

View Solution
