The magnetic field at O is
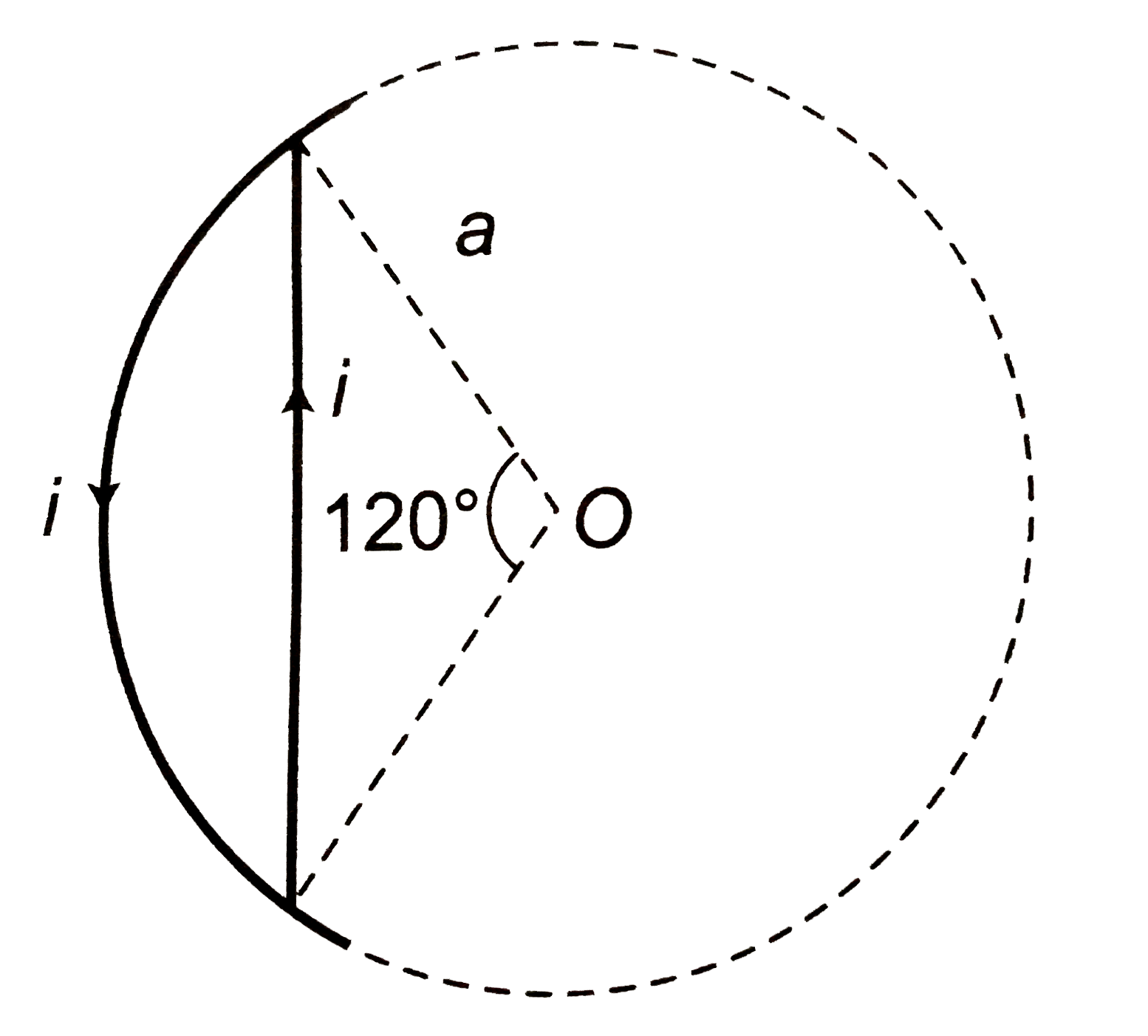
μ0i2π(√3π−13)
μ0i2a(√2π−12)
μ0ia(√3π−13)
μ0i4a(√3π−13)
The correct Answer is:A
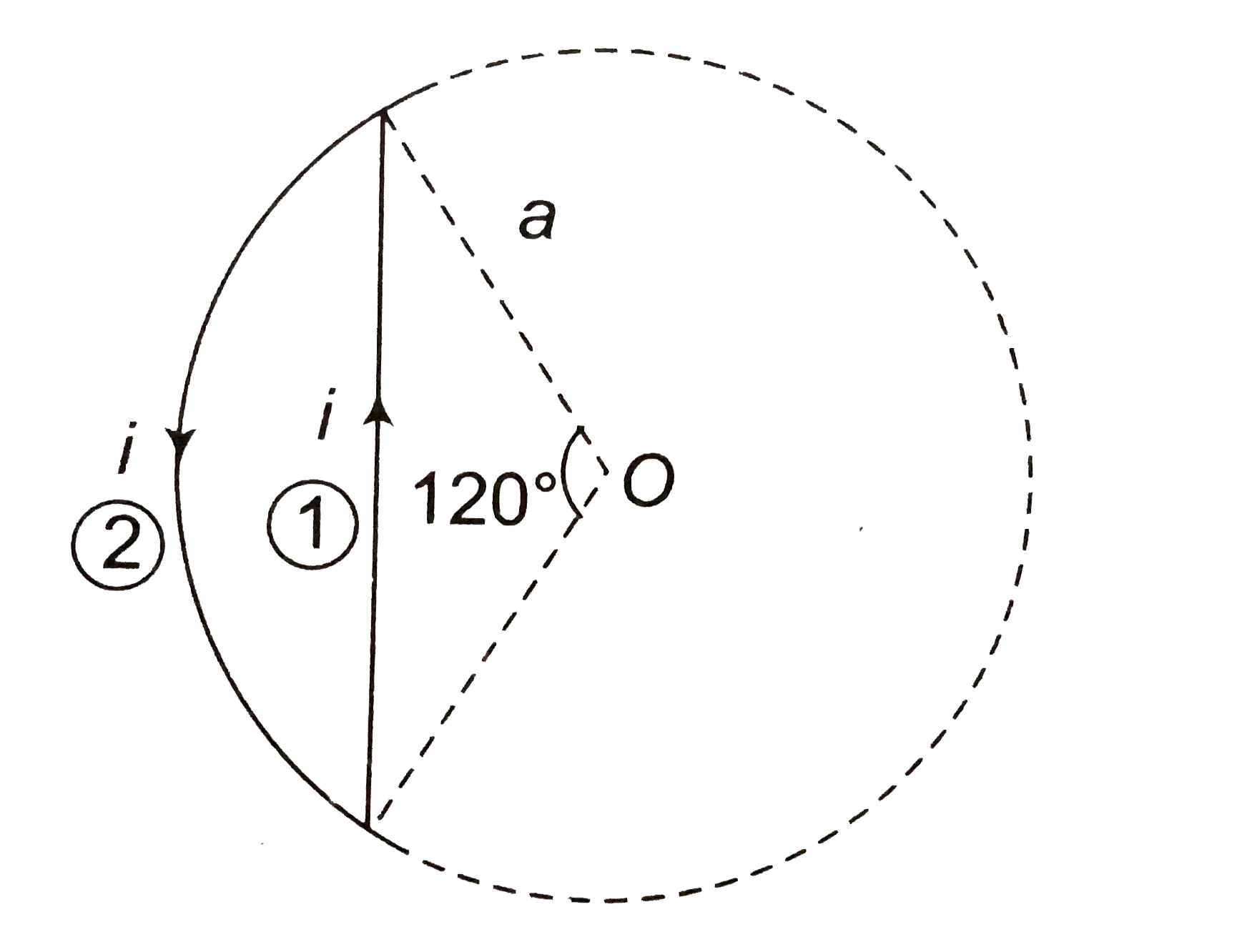
B1=μ0i4πacos60∘(cos30∘+cos30∘)
=μ0i2πa.√3,⊗
B2=μ0i2a.120∘360∘=μ0i6a,⊙
BO=B1−B2=μ0i2a(√3π−13),⊗
Topper's Solved these Questions
Similar Questions
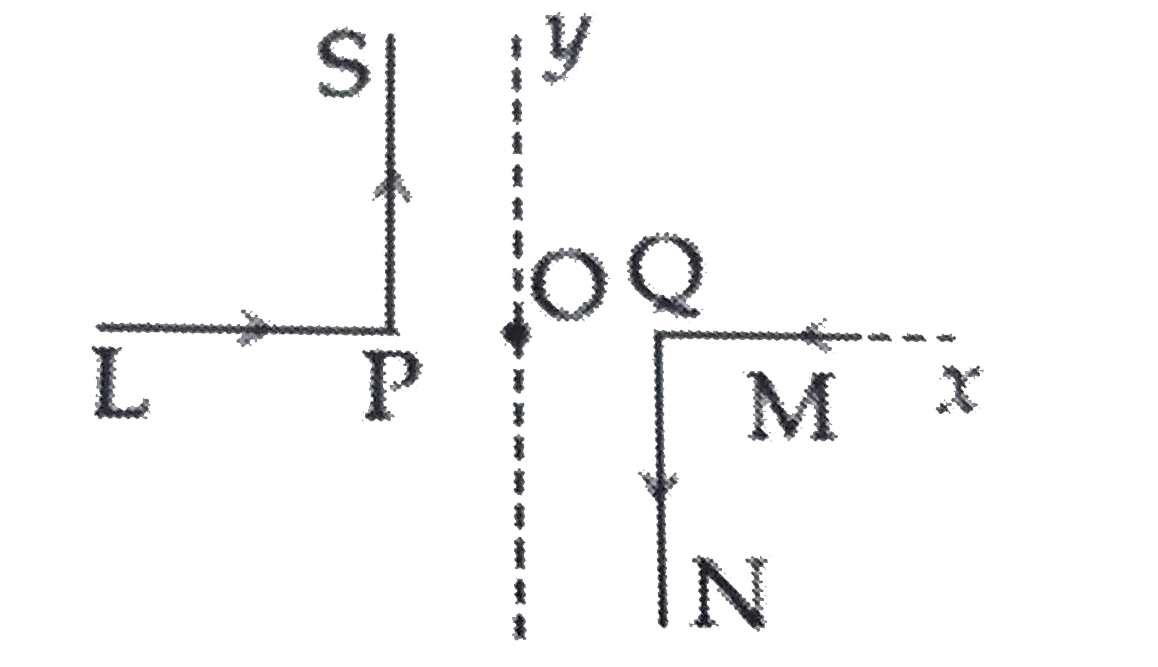
As shown in the figure two infinitely long, identical wires are bent by 90∘ and placed in such a way that the segments LP and QM are along the x-axis , while segments PS and QN are parallel to the Y-axis If OP=OQ=4 cm ,and the magnitude of the magnetic field at O is 10−4 T, and the magnitude of the current in each wire and the direction of the magnetic field at O will be
(μo=4π×10−7NA−2):
View SolutionA loop ABCD has current I = 10 A , as shown in the figure . AD and BC are circular arcs with centre at O, for both . The magnetic field at point O is

View Solution
Knowledge Check
the unit vector ˆi,ˆj and ˆk are as shown below. What will be the magnetic field at O in the following figure ?
 Aμ04πia(2−π2)ˆjBμ04πia(2+π2)ˆjCμ04πia(2+π2)ˆiDμ04πia(2+π2)ˆk
Aμ04πia(2−π2)ˆjBμ04πia(2+π2)ˆjCμ04πia(2+π2)ˆiDμ04πia(2+π2)ˆkThe unit vectors ˆi,ˆj and ˆk are as shown below. What will be the magnetic field at O in the following figure
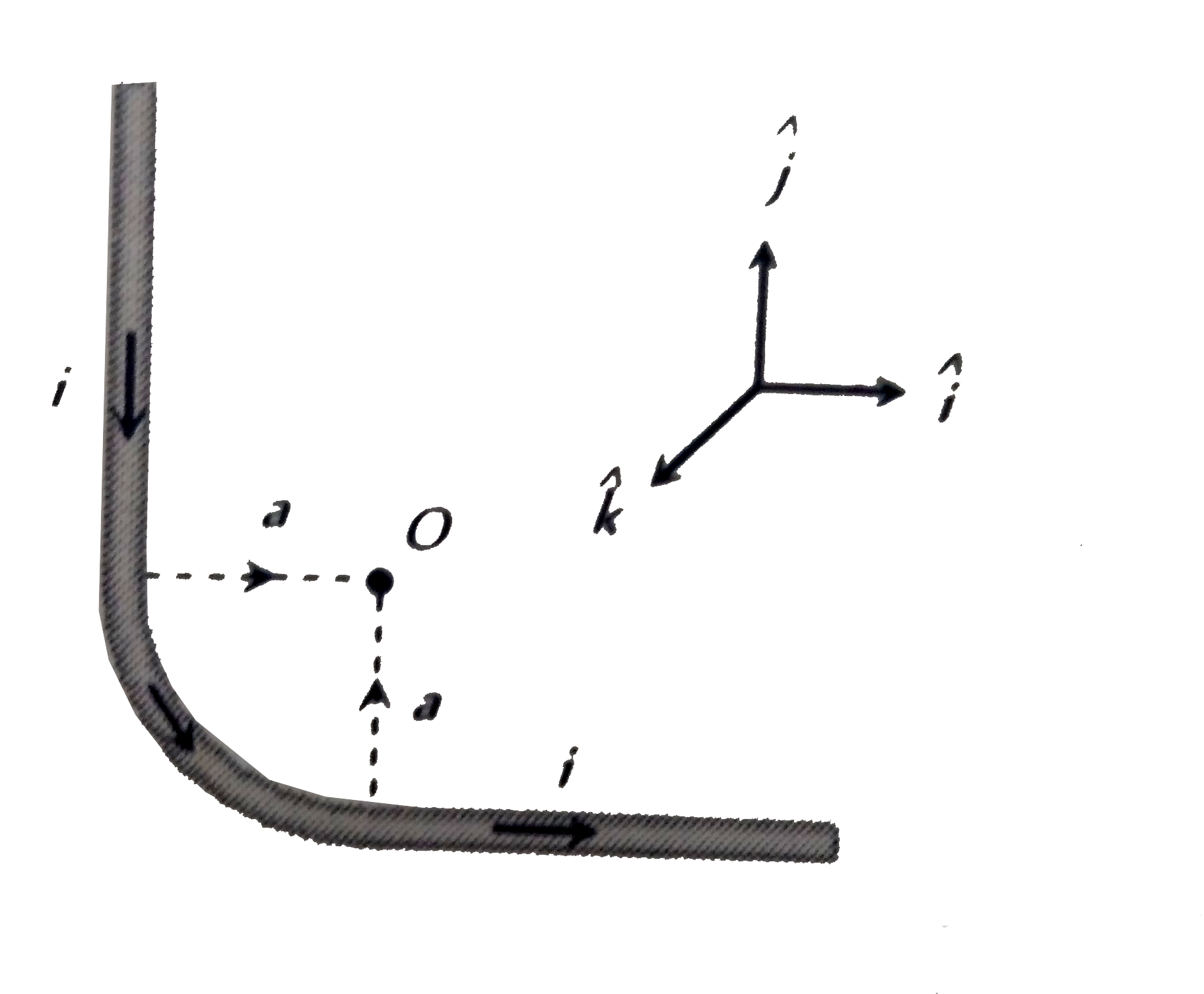 Aμ04πia(2−π2)ˆjBμ04πia(2+π2)ˆjCμ04πia(2+π2)ˆiDμ04πia(2+π2)ˆk
Aμ04πia(2−π2)ˆjBμ04πia(2+π2)ˆjCμ04πia(2+π2)ˆiDμ04πia(2+π2)ˆkThe magnetic field at O due to current in the infinite wire forming a loop as a shown in Fig.
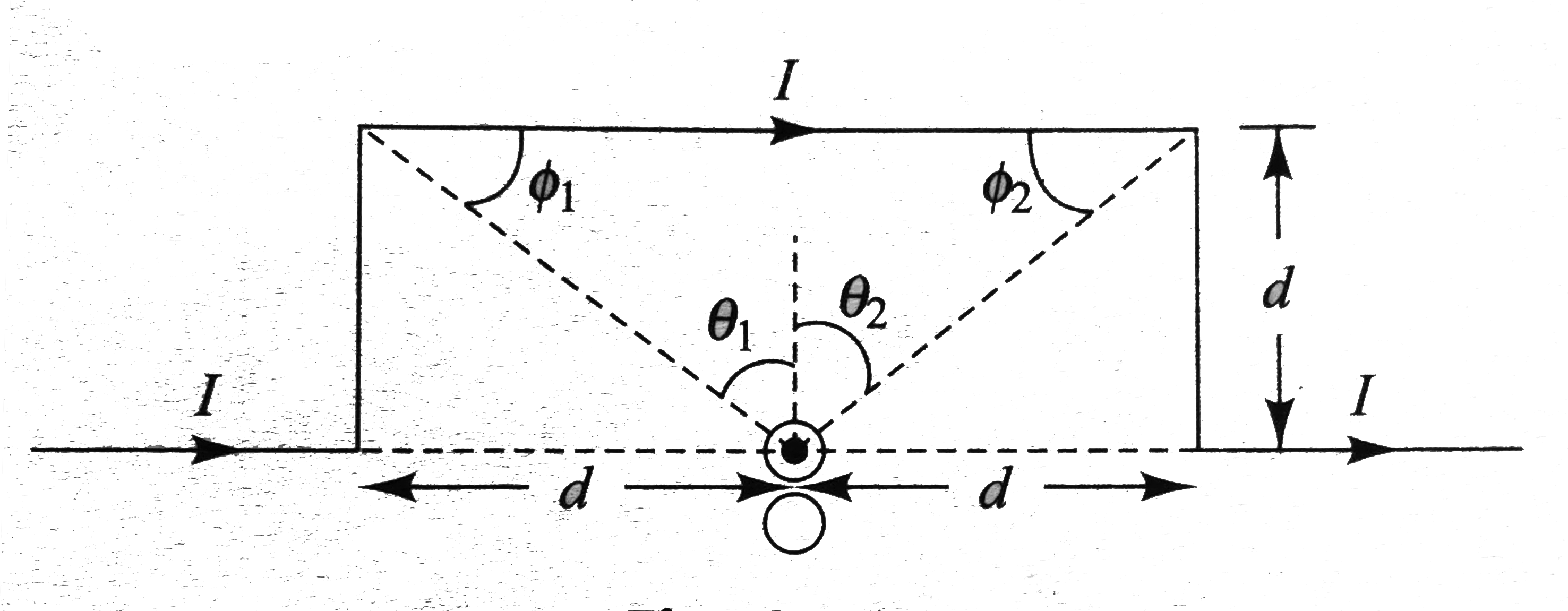 Aμ0I2πd(cosϕ1+cosϕ2)Bμ04π2Id(tanθ1+tanθ2)Cμ04πId(sinϕ1+sinϕ2)Dμ04πId(cosθ1+cosθ2)
Aμ0I2πd(cosϕ1+cosϕ2)Bμ04π2Id(tanθ1+tanθ2)Cμ04πId(sinϕ1+sinϕ2)Dμ04πId(cosθ1+cosθ2)
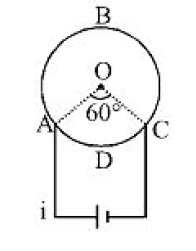
A cell is connected between the points A and C of a circular conductor ABCD of centre 'O'.∠AOC=60∘. IfB1andB2 are the magnitudes of the magnetic fields at O due to the currents in ABC and ADC respectively, the ratio B1B2, is
View SolutionIn the given figure the magnitude of magnetic field at O is ( all three wires are quarter circular ares )

View SolutionTwo small magnets of each of magnetic moment M0 is placed parallel to each other (show in figure). The magnetic field at point O is

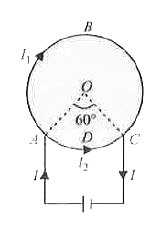
View SolutionA cell is connected between the points A and Cofa circular conductor ABCDA of centre O. ∠AOC=60∘. If B1, and B2, are the magnitudes of the magnetic fields at O due to currents in ABC and ADC respectively, the ratio B1/B2, is
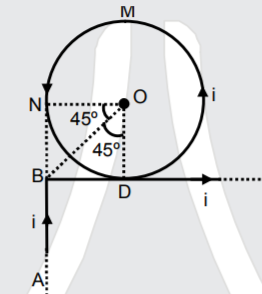
View SolutionFind magnetic field at O.
View Solution