The moment of inertia of a rod about its perpendicular bisector is I . When the temperature of the rod is increased by ΔT , the increase in the moment of inertia of the rod about the same axis is (Here , α is the coefficient of linear expansion of the rod )
αIΔT
2αIΔT
αIΔT2
2IΔTα
The correct Answer is:B
To solve the problem, we need to determine the increase in the moment of inertia of a rod when its temperature is increased by ΔT. The moment of inertia of a rod about its perpendicular bisector is given as I.
1. Understand the Moment of Inertia:
The moment of inertia I of a rod of mass m and length L about its perpendicular bisector is given by the formula:
I=112mL2
2. Determine the Change in Length:
When the temperature of the rod is increased by ΔT, the length of the rod changes due to thermal expansion. The change in length ΔL can be expressed as:
ΔL=LαΔT
where α is the coefficient of linear expansion of the material of the rod.
3. Calculate the New Length:
The new length L′ of the rod after the temperature increase will be:
L′=L+ΔL=L+LαΔT=L(1+αΔT)
4. Calculate the New Moment of Inertia:
The new moment of inertia I′ about the same axis (perpendicular bisector) can be calculated using the new length L′:
I′=112m(L′)2=112m(L(1+αΔT))2
Expanding this:
I′=112m(L2(1+2αΔT+(αΔT)2))
5. Neglect Higher Order Terms:
Since ΔT is small, we can neglect the term (αΔT)2:
I′≈112mL2(1+2αΔT)=I(1+2αΔT)
6. Find the Increase in Moment of Inertia:
The increase in moment of inertia ΔI is given by:
ΔI=I′−I=I(1+2αΔT)−I=2αIΔT
Final Answer:
The increase in the moment of inertia of the rod about the same axis when the temperature is increased by ΔT is:
ΔI=2αIΔT
The moment of inertia I of a rod of mass m and length L about its perpendicular bisector is given by the formula:
I=112mL2
When the temperature of the rod is increased by ΔT, the length of the rod changes due to thermal expansion. The change in length ΔL can be expressed as:
ΔL=LαΔT
where α is the coefficient of linear expansion of the material of the rod.
The new length L′ of the rod after the temperature increase will be:
L′=L+ΔL=L+LαΔT=L(1+αΔT)
The new moment of inertia I′ about the same axis (perpendicular bisector) can be calculated using the new length L′:
I′=112m(L′)2=112m(L(1+αΔT))2
Expanding this:
I′=112m(L2(1+2αΔT+(αΔT)2))
Since ΔT is small, we can neglect the term (αΔT)2:
I′≈112mL2(1+2αΔT)=I(1+2αΔT)
The increase in moment of inertia ΔI is given by:
ΔI=I′−I=I(1+2αΔT)−I=2αIΔT
The increase in the moment of inertia of the rod about the same axis when the temperature is increased by ΔT is:
ΔI=2αIΔT
Topper's Solved these Questions
- THERMAL PROPERTIES OF MATTERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Specific Heat
5 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Calorimetery
4 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Measurement of Temperature
2 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - NCERT Exemplar
8 Videos
- THERMODYNAMICSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
The moment of inertia of a rod about its perpendicular bisector is l, when temperature of rod is increased by ΔT, the increase in the moment of inertia of the rod about same axis (γ = temperature coefficient of volume expansion)
View SolutionCalculate the moment of inertia of the given rod about an axis CD
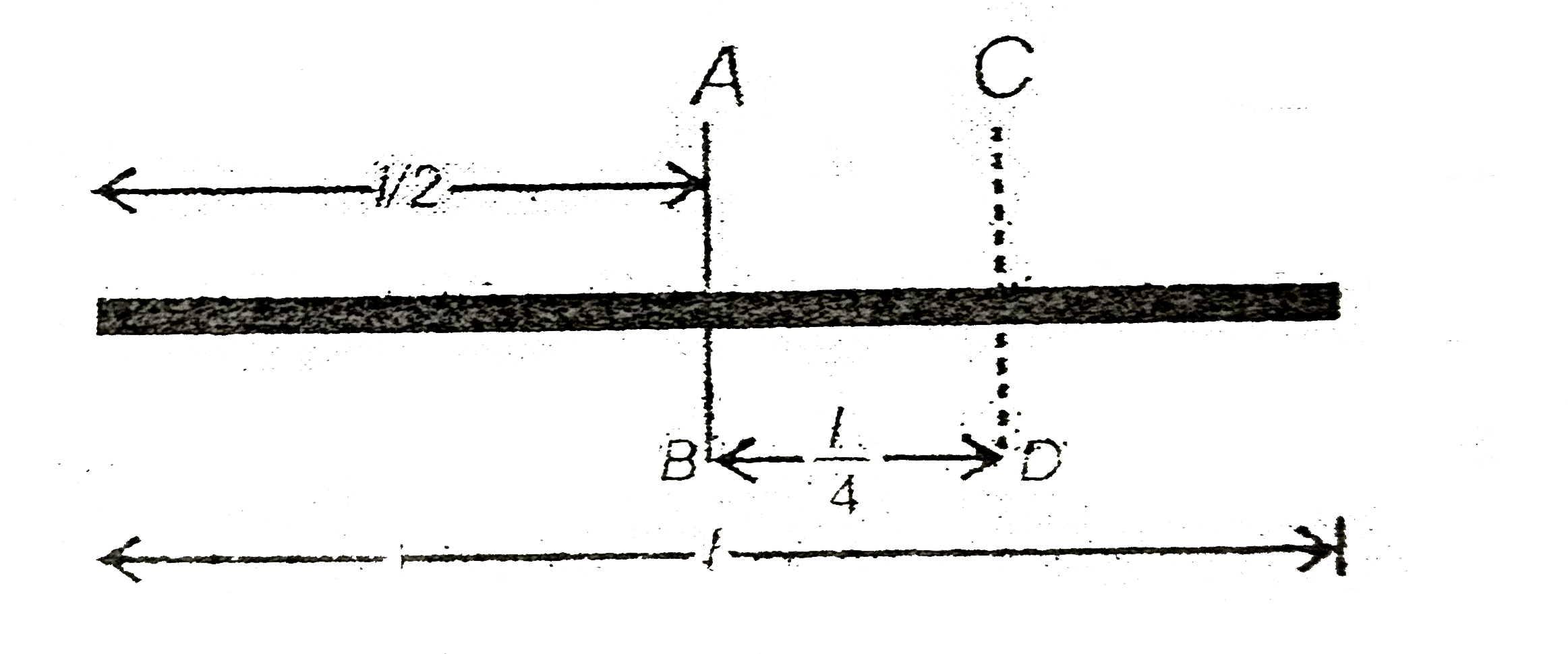
View SolutionCalculate the moment of inertia of the given rod about an axis CD.
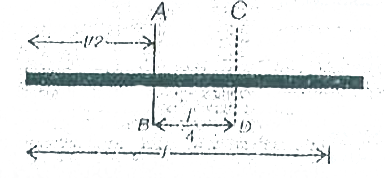
View SolutionMoment of inertia of a inclined rod.
View SolutionThe moment of inertia of a ring about its geometrical axis is I, then its moment of inertia about its diameter will be
View SolutionThe moment of inertia of a uniform cylinder of length landradiusR about its perpendicular bisector is I. What is the ratio l/R such that the moment of inertia is minimum ?
View SolutionIf the temperature of a uniform rod is slighely increased by Δt its moment of inertia I about perpendicular bisector increases by
View SolutionIf the temperature of a uniform rod is slightly increased by Δt, its moment of inertia I about a line parallel to itself will increased by
View SolutionWhen the temperature of a rod increases from t to r+Δt, its moment of inertia increases from I to I+ΔI. If α is the value of ΔI/I is
View SolutionIf I1 is the moment of inertia of a thin rod about an axis perpendicular to its length and passing through its centre of mass and I2 is the moment of inertia of the ring about an axis perpendicular to plane of ring and passing through its centre formed by bending the rod, then
View Solution
