The negative plate of a parallel plate capacitor is given a charge of $- 20 X 10^{- 8} C$ . Find the charges appearing on the four surface of the capacitor plates.

Video Solution

Text Solution

Verified by Experts

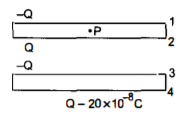
Let the charge appearing on the inner surface of the nagative plate be -Q. The charge on its outer surface will be $$ Q - 20 X 10 ^(-8) C $$ . The charge on the inner surface of the positive plate will be + Q from Gauss's law and that on the outer surface will be -Q as the positive plate is electrically neutral. The distribution is shown in figure. To obtain the value of Q, consider the electric field at a point P inside the upper plate. Field due to suface (1) $$ = (Q)/(2 epsilon_0 A) $$ upward, due to surface (2) $$ =(Q)/(2epsilon_0 A) $$ upward, due ot surface (3) $$ = (Q)/(2 epsilon_0 A) $$ downward and due to surface (4) $$ = (Q - 20 X10^(-C)/(2epsilon_0 A) $$ upward. As P is a point inside the conductor, the field here must be zero. Thus, $$ Q = - Q +20 X10^(-8) $$ C. or Q = 10 X 10^(-8) $$ C. The charges on the four surfaces may be written immediately from figure.

Updated on:21/07/2023

The capacity of a parallel plate air capacitor is $10 μ F$ and it is given a charge of $40 μ C$ . The electrical energy stored in the capacitor is
A400 erg
B600 erg
C800 erg
D900 erg
A parallel plate capacitor is charged. If the plates are pulled apart
Athe potential difference increases
Bthe capacitance increases
Cthe total charge increases
Dthe charge and the potential difference remain the same
The plates of small size of a parallel plate capacitor are charged as shown. The force on the charged particle of 'q' at a distance 'l' from the capacitor is : (Assume that the distance between the plates is $d < < l$ )

Azero
B $\frac{Q q d}{2 π ε_{0} l^{3}}$
C $\frac{Q q d}{π ε_{0} l^{3}}$
D $\frac{Q q d}{4 π ε_{0} l^{3}}$