The period of a particle in SHM is 8s. At t=0 it is in its equilibrium position.
(a) Compare the distance travelled in the first 4s and the second 4s.
(b) Compare the distance travelled in the first 2s and the second 2s.
The correct Answer is:A, B
To solve the problem, we will analyze the motion of a particle in Simple Harmonic Motion (SHM) based on the given period and the distances traveled in specified time intervals.
Given:
- Period T=8s
- At t=0, the particle is at its equilibrium position.
Part (a): Compare the distance traveled in the first 4 seconds and the second 4 seconds.
1. Understanding the Motion:
- The period T=8s means the particle completes one full cycle in 8 seconds.
- Half of the period T2=4s is the time taken to move from the equilibrium position to one extreme and back to the equilibrium position.
2. Distance Traveled in the First 4 Seconds:
- From t=0 to t=4s:
- The particle moves from the equilibrium position (O) to one extreme position (P) and back to the equilibrium position (O).
- Distance traveled d1=OP+PO=A+A=2A.
3. Distance Traveled in the Second 4 Seconds:
- From t=4s to t=8s:
- The particle moves from the equilibrium position (O) to the opposite extreme position (Q) and back to the equilibrium position (O).
- Distance traveled d2=OQ+QO=A+A=2A.
4. Comparison:
- Since d1=2A and d2=2A, we find that:
d1=d2
- Therefore, the distance traveled in the first 4 seconds is equal to the distance traveled in the second 4 seconds.
Part (b): Compare the distance traveled in the first 2 seconds and the second 2 seconds.
1. Understanding the Motion:
- The time interval of 2 seconds corresponds to T4=2s, which is one-fourth of the total period.
2. Distance Traveled in the First 2 Seconds:
- From t=0 to t=2s:
- The particle moves from the equilibrium position (O) to the maximum amplitude position (P).
- Distance traveled d1=OP=A.
3. Distance Traveled in the Second 2 Seconds:
- From t=2s to t=4s:
- The particle moves from the maximum amplitude position (P) back to the equilibrium position (O).
- Distance traveled d2=PO=A.
4. Comparison:
- Since d1=A and d2=A, we find that:
d1=d2
- Therefore, the distance traveled in the first 2 seconds is equal to the distance traveled in the second 2 seconds.
Summary of Results:
- (a) The distance traveled in the first 4 seconds is equal to the distance traveled in the second 4 seconds.
- (b) The distance traveled in the first 2 seconds is equal to the distance traveled in the second 2 seconds.
- Period T=8s
- At t=0, the particle is at its equilibrium position.
- The period T=8s means the particle completes one full cycle in 8 seconds.
- Half of the period T2=4s is the time taken to move from the equilibrium position to one extreme and back to the equilibrium position.
- From t=0 to t=4s:
- The particle moves from the equilibrium position (O) to one extreme position (P) and back to the equilibrium position (O).
- Distance traveled d1=OP+PO=A+A=2A.
- From t=4s to t=8s:
- The particle moves from the equilibrium position (O) to the opposite extreme position (Q) and back to the equilibrium position (O).
- Distance traveled d2=OQ+QO=A+A=2A.
- Since d1=2A and d2=2A, we find that:
d1=d2
- Therefore, the distance traveled in the first 4 seconds is equal to the distance traveled in the second 4 seconds.
- The time interval of 2 seconds corresponds to T4=2s, which is one-fourth of the total period.
- From t=0 to t=2s:
- The particle moves from the equilibrium position (O) to the maximum amplitude position (P).
- Distance traveled d1=OP=A.
- From t=2s to t=4s:
- The particle moves from the maximum amplitude position (P) back to the equilibrium position (O).
- Distance traveled d2=PO=A.
- Since d1=A and d2=A, we find that:
d1=d2
- Therefore, the distance traveled in the first 2 seconds is equal to the distance traveled in the second 2 seconds.
- (a) The distance traveled in the first 4 seconds is equal to the distance traveled in the second 4 seconds.
- (b) The distance traveled in the first 2 seconds is equal to the distance traveled in the second 2 seconds.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Single Correct
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 More Than One Correct
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Single Correct
24 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
x−t equation of a particle in SHM is
x=(4cm)cos(π2t)
Here ,t is in seconds. Find the distance travelled by the particle in first three seconds.
View SolutionThe speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
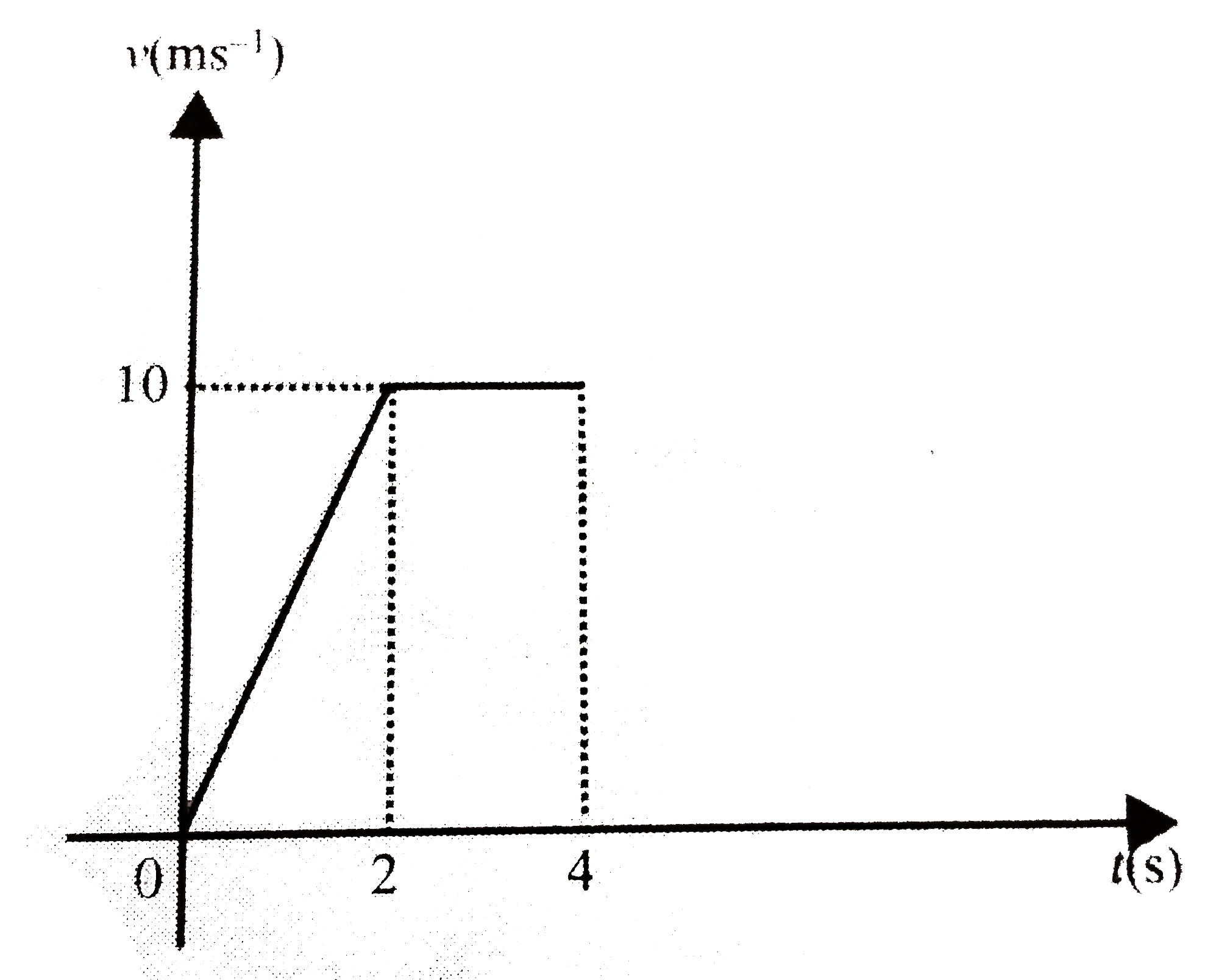
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View SolutionIf the distance travelled by a freely falling body in the last second of its journey is equal to the distance travelled in the first 2s, the time of descent of the body is
View SolutionA particle initially at rest, moves with an acceleration 5 m s−2 for 5 s. Find the distance travelled in (a) 4 s, (b) 5 s and (c) 5th second.
View SolutionA body starting from rest has an acceleration of 5m/s2. Calculate the distance travelled by it in 4th second.
View SolutionA body starts from rest and accelerates with 4m/s2 for 5 seconds. Find the distance travelled with 5 seconds.
View SolutionFig. 2.44 shows the velocity-time graph of a particle moving in a straight line.
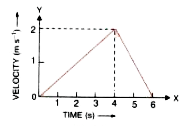
(i) State the nature of motion of particle.
(ii) Find the displacement of particle at t = 6 s.
(iii) Does the particle change its direction of motion ?
(iv) Compare the distance travelled by the particle from 0 to 4 s and from 4 s to 6 s.
(v) Find the acceleration from 0 to 4 s and retardation from 4 s to 6 s.
View SolutionThe position of a particle along x-axis at time t is given by x=1+t−t2. The distance travelled by the particle in first 2 seconds is
View SolutionThe variation of velocity of a particle moving along a straight line is shown in the figure. The distance travelled by the particle in 4s is
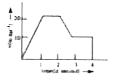
View SolutionThe motion of a particle is described by the equation at u=at.The distance travelled by the particle in the first 4 seconds
View Solution
