The position of a particle along x-axis at time t is given by x=1+t−t2. The distance travelled by the particle in first 2 seconds is
1 m
2 m
2.5 m
3 m
The correct Answer is:C
To find the distance traveled by the particle in the first 2 seconds, we will follow these steps:
Step 1: Define the position function
The position of the particle along the x-axis is given by the equation:
x(t)=1+t−t2
Step 2: Find the velocity function
To determine if the particle changes direction, we need to find the velocity, which is the derivative of the position function with respect to time t:
v(t)=dxdt=ddt(1+t−t2)
Calculating the derivative:
v(t)=0+1−2t=1−2t
Step 3: Determine when the velocity is zero
Set the velocity function equal to zero to find when the particle changes direction:
1−2t=0
Solving for t:
2t=1
t=12 seconds
Step 4: Calculate the position at key time points
Now we will calculate the position of the particle at t=0, t=12, and t=2 seconds.
- At t=0:
x(0)=1+0−02=1
- At t=12:
x(12)=1+12−(12)2=1+12−14=1+24−14=1+14=54
- At t=2:
x(2)=1+2−22=1+2−4=−1
Step 5: Calculate the distance traveled
Now we can calculate the distance traveled in two segments:
1. From t=0 to t=12:
- The distance is |x(12)−x(0)|=∣∣∣54−1∣∣∣=∣∣∣54−44∣∣∣=14
2. From t=12 to t=2:
- The distance is |x(2)−x(12)|=|−1−54|=|−1−1.25|=|−2.25|=2.25
Step 6: Total distance traveled
Now, add the distances from both segments:
Total distance=14+2.25=14+94=104=2.5
Final Answer
The total distance traveled by the particle in the first 2 seconds is:
2.5
---
The position of the particle along the x-axis is given by the equation:
x(t)=1+t−t2
To determine if the particle changes direction, we need to find the velocity, which is the derivative of the position function with respect to time t:
v(t)=dxdt=ddt(1+t−t2)
Calculating the derivative:
v(t)=0+1−2t=1−2t
Set the velocity function equal to zero to find when the particle changes direction:
1−2t=0
Solving for t:
2t=1
t=12 seconds
Now we will calculate the position of the particle at t=0, t=12, and t=2 seconds.
x(0)=1+0−02=1
x(12)=1+12−(12)2=1+12−14=1+24−14=1+14=54
x(2)=1+2−22=1+2−4=−1
Now we can calculate the distance traveled in two segments:
1. From t=0 to t=12:
- The distance is |x(12)−x(0)|=∣∣∣54−1∣∣∣=∣∣∣54−44∣∣∣=14
- The distance is |x(2)−x(12)|=|−1−54|=|−1−1.25|=|−2.25|=2.25
Now, add the distances from both segments:
Total distance=14+2.25=14+94=104=2.5
The total distance traveled by the particle in the first 2 seconds is:
2.5
Topper's Solved these Questions
Similar Questions
Position of a particle moving along x-axis is given by x=2+8t−4t2. The distance travelled by the particle from t=0 to t=2 is:-
View SolutionThe position of a particle along X-axis at time t is given by x=2+t−3t2. The displacement and the distance travelled in the interval, t = 0 to t = 1 are respectively
View SolutionA particle moves along a straight line on y-axis. The distance of the particle from O varies with time and is given by : y=20t−t2. The distance travelled by the particle before it momentarily comes to rest is
View SolutionA particle moves along x-axis such that its position veries with time as x=50t−5t2. Select the correct alternative (s).
View SolutionVelocity of a particle moving along a straight line at any time 't' is give by V=cos(π3t) . Then the distance travelled by the particle in the first two seconds is :
View SolutionThe position x of a particle moving along x - axis at time (t) is given by the equation t=√x+2, where x is in metres and t in seconds. Find the work done by the force in first four seconds
View SolutionThe position of a particle along x-axis at certain time is given below
Determine the following
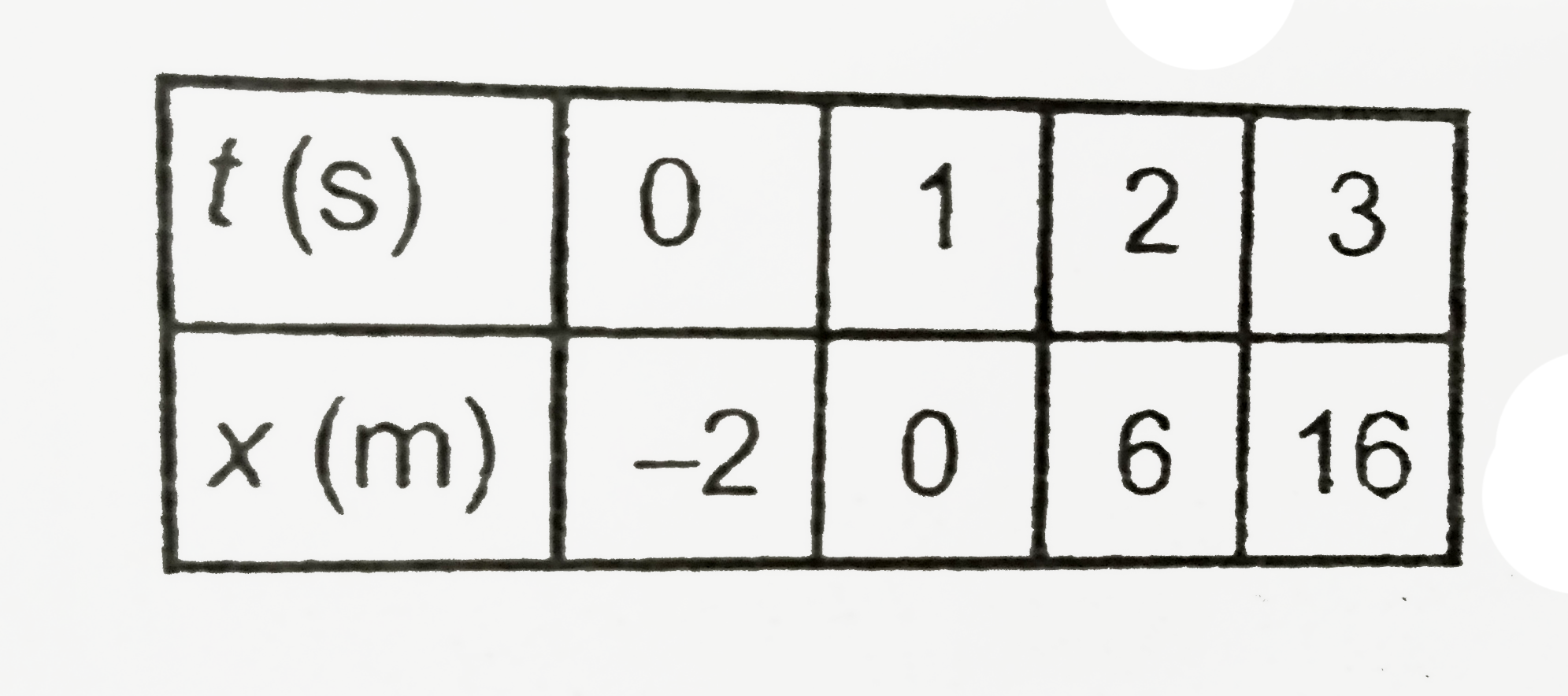
(a) Average velocity of particle in first 3 seconds.
(b) Position of particle at the end of 4th second if particle continue the trend.
(c) Acceleration of particle at time t = 3 s
(d) Type of motion
View SolutionThe displacement x of a particle along the x-axis at time t is given by x=a12t+a23t2. Find the acceleration of the particle.
View SolutionThe displacement x of a particle moving along x-axis at time t is given by x2=2t2+6t. The velocity at any time t is
View SolutionA particle is moving along the x-axis whose acceleration is given by a=3x−4, where x is the location of the particle. At t = 0, the particle is at rest at x=4/3m. The distance travelled by the particles in 5 s is
View Solution
