The position of center of mass of a system of particles does not depend upon the
mass of particles
symmetry of the body
position of the particles
nature of particles.
To determine what the position of the center of mass of a system of particles does not depend upon, we can analyze the factors involved in calculating the center of mass.
1. Understanding the Center of Mass Formula:
The position of the center of mass (COM) for a system of particles is given by the formula:
RCOM=∑miri∑mi
where mi is the mass of the i-th particle and ri is its position vector.
2. Analyzing Each Option:
- Mass of the Particles: The formula includes mi, indicating that the center of mass does depend on the mass of the particles.
- Symmetry of the Body: The symmetry affects the distribution of mass and thus influences the center of mass position. For example, a uniform ring has its center of mass at the center, while an asymmetric shape will have a different center of mass.
- Position of the Particles: The position vectors ri are directly used in the formula, meaning the center of mass is dependent on where the particles are located.
- Nature of the Particles: This refers to the intrinsic properties of the particles (e.g., whether they are protons, neutrons, etc.). The formula does not include any characteristics that define the nature of the particles, only their masses and positions.
3. Conclusion:
Based on the analysis, the position of the center of mass does not depend on the nature of the particles. Therefore, the correct answer is:
- The position of center of mass does not depend on the nature of the particles.
Final Answer:
The position of center of mass of a system of particles does not depend upon the nature of the particles.
---
The position of the center of mass (COM) for a system of particles is given by the formula:
RCOM=∑miri∑mi
where mi is the mass of the i-th particle and ri is its position vector.
- Mass of the Particles: The formula includes mi, indicating that the center of mass does depend on the mass of the particles.
- Symmetry of the Body: The symmetry affects the distribution of mass and thus influences the center of mass position. For example, a uniform ring has its center of mass at the center, while an asymmetric shape will have a different center of mass.
- Position of the Particles: The position vectors ri are directly used in the formula, meaning the center of mass is dependent on where the particles are located.
- Nature of the Particles: This refers to the intrinsic properties of the particles (e.g., whether they are protons, neutrons, etc.). The formula does not include any characteristics that define the nature of the particles, only their masses and positions.
Based on the analysis, the position of the center of mass does not depend on the nature of the particles. Therefore, the correct answer is:
- The position of center of mass does not depend on the nature of the particles.
The position of center of mass of a system of particles does not depend upon the nature of the particles.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Match the coloumns
9 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
Similar Questions
Knowledge Check
The position of centre of mass does not depend upon the reference frame.
Centre of mass depends only upon the mass of the body.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertionCIf assertion is true but reason is false.DIf both assertion and reason are false.
The center of mass of a system of two particles divides the distance between them.
View SolutionThe center of mass of a system of two particles divides the distance between them.
View SolutionCalculate the position of centre of mass of a system consisting of two particles of masses m_1 and m_2 separated by a distance L apar, from m_1?
View SolutionAdsorption depends upon
View SolutionThe position co-ordinates of the centre of mass of a system of three particles of equal masses which are placed on the verctices of an equilateral triangle of a side a are shown. Locate centre mass?
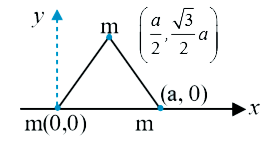
View SolutionThe pitch of sound does not depend upon :
View SolutionThe coordinates of the centre of mass of a system of three particles of mass 1g,2g and 3g are (2,2,2). Where should a fourth particle of mass 4 g be positioned so that the centre of mass of the four particle system is at the origin of the three-dimensional coordinate system ?
View Solution
