The coordinates of the centre of mass of a system of three particles of mass 1g,2g and 3g are (2,2,2). Where should a fourth particle of mass 4 g be positioned so that the centre of mass of the four particle system is at the origin of the three-dimensional coordinate system ?
(a)(1,1,1)
(b)(2,2,2)
(c)(3,3,3)
(d)(−3,−3,−3)
The correct Answer is:D
To solve the problem of finding the position of a fourth particle of mass 4g so that the center of mass of the four-particle system is at the origin, we can follow these steps:
Step 1: Understand the given data
We have three particles with the following masses and coordinates:
- Mass m1=1g at coordinates (2,2,2)
- Mass m2=2g at coordinates (2,2,2)
- Mass m3=3g at coordinates (2,2,2)
The total mass of these three particles is:
m1+m2+m3=1g+2g+3g=6g
Step 2: Calculate the center of mass of the three particles
The center of mass (xcm,ycm,zcm) for the three particles can be calculated using the formula:
xcm=m1x1+m2x2+m3x3m1+m2+m3
ycm=m1y1+m2y2+m3y3m1+m2+m3
zcm=m1z1+m2z2+m3z3m1+m2+m3
Substituting the values:
xcm=1⋅2+2⋅2+3⋅26=126=2
ycm=1⋅2+2⋅2+3⋅26=126=2
zcm=1⋅2+2⋅2+3⋅26=126=2
Thus, the center of mass of the three particles is at (2,2,2).
Step 3: Introduce the fourth particle
Let the fourth particle have mass m4=4g and its coordinates be (x4,y4,z4). We want the center of mass of the four particles to be at the origin (0,0,0).
Step 4: Set up the equations for the center of mass of four particles
The center of mass for the four particles can be expressed as:
xcm=m1x1+m2x2+m3x3+m4x4m1+m2+m3+m4=0
ycm=m1y1+m2y2+m3y3+m4y4m1+m2+m3+m4=0
zcm=m1z1+m2z2+m3z3+m4z4m1+m2+m3+m4=0
Step 5: Substitute the known values into the equations
Substituting the known values into the x-coordinate equation:
0=1⋅2+2⋅2+3⋅2+4x46+4
0=12+4x410
Multiplying both sides by 10:
0=12+4x4
Solving for x4:
4x4=−12⟹x4=−3
Step 6: Repeat for y and z coordinates
Using the same approach for the y-coordinate:
0=1⋅2+2⋅2+3⋅2+4y410
0=12+4y4⟹y4=−3
For the z-coordinate:
0=1⋅2+2⋅2+3⋅2+4z410
0=12+4z4⟹z4=−3
Step 7: Conclusion
The coordinates of the fourth particle should be (−3,−3,−3).
Final Answer
The fourth particle of mass 4g should be positioned at (−3,−3,−3).
---
We have three particles with the following masses and coordinates:
- Mass m1=1g at coordinates (2,2,2)
- Mass m2=2g at coordinates (2,2,2)
- Mass m3=3g at coordinates (2,2,2)
m1+m2+m3=1g+2g+3g=6g
The center of mass (xcm,ycm,zcm) for the three particles can be calculated using the formula:
xcm=m1x1+m2x2+m3x3m1+m2+m3
ycm=m1y1+m2y2+m3y3m1+m2+m3
zcm=m1z1+m2z2+m3z3m1+m2+m3
xcm=1⋅2+2⋅2+3⋅26=126=2
ycm=1⋅2+2⋅2+3⋅26=126=2
zcm=1⋅2+2⋅2+3⋅26=126=2
Let the fourth particle have mass m4=4g and its coordinates be (x4,y4,z4). We want the center of mass of the four particles to be at the origin (0,0,0).
The center of mass for the four particles can be expressed as:
xcm=m1x1+m2x2+m3x3+m4x4m1+m2+m3+m4=0
ycm=m1y1+m2y2+m3y3+m4y4m1+m2+m3+m4=0
zcm=m1z1+m2z2+m3z3+m4z4m1+m2+m3+m4=0
Substituting the known values into the x-coordinate equation:
0=1⋅2+2⋅2+3⋅2+4x46+4
0=12+4x410
Multiplying both sides by 10:
0=12+4x4
Solving for x4:
4x4=−12⟹x4=−3
Using the same approach for the y-coordinate:
0=1⋅2+2⋅2+3⋅2+4y410
0=12+4y4⟹y4=−3
0=1⋅2+2⋅2+3⋅2+4z410
0=12+4z4⟹z4=−3
The coordinates of the fourth particle should be (−3,−3,−3).
The fourth particle of mass 4g should be positioned at (−3,−3,−3).
Similar Questions
Knowledge Check
Centre of mass of three particles of masses 1kg,2kgand3kg lies at the point (1,2,3) and centre of mass of another system of particles 3kgand2kg lies at the point (−1,3,−2). Where should we put a particle of mass 5kg so that the centre of mass of entire system lies at the centre of mass of first system ?
A(0, 0, 0)B(1, 3, 2)C(-1, 2, 3)D(3, 1, 8)
The position of center of mass of a system of particles does not depend upon the
View SolutionThe centre of mass of three particles of masses 1 kg, 2 kg and 3 kg is at (2,2, 2). The position of the fourth mass of 4 kg to be placed in the system as that the new centre of mass is at (0, 0, 0) is
View SolutionThe position co-ordinates of the centre of mass of a system of three particles of equal masses which are placed on the verctices of an equilateral triangle of a side a are shown. Locate centre mass?
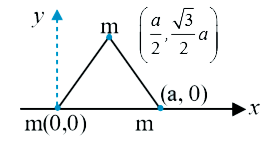
View SolutionThe position of centre of mass of system of particles at any moment does not depend on.
View SolutionThe centre of mass of a system of particles is at the origin. It follows that:
View SolutionLocate the centre of mass of a system of three particles 1.0 kg, 2.0 kg and 3.0 kg placed at the corners of an equilateral traingular of side 1m.
View SolutionIf all the particle of a system lie in a cube is it necessary that the centre of mass be in the cube?
View Solution