The torque and magnetic potential energy of a magnetic dipole in most stable position ina uniform magnetic field →B having magnetic moment →m will be
−mB,zero
mB,zero
zero,mB
zero,−mB
The correct Answer is:D
To solve the problem regarding the torque and magnetic potential energy of a magnetic dipole in its most stable position in a uniform magnetic field, we will follow these steps:
Step 1: Understand the Definitions
- Torque (τ): The torque experienced by a magnetic dipole in a magnetic field is given by the formula:
τ=→m×→B
or in magnitude form:
τ=mBsinθ
where m is the magnetic moment, B is the magnetic field strength, and θ is the angle between →m and →B.
- Magnetic Potential Energy (Um): The potential energy of a magnetic dipole in a magnetic field is given by:
Um=−→m⋅→B
or in magnitude form:
Um=−mBcosθ
Step 2: Determine the Most Stable Position
- The most stable position of a magnetic dipole in a magnetic field occurs when the dipole is aligned with the field, which corresponds to θ=0∘.
Step 3: Calculate Torque at θ=0∘
- Substitute θ=0∘ into the torque equation:
τ=mBsin(0∘)=mB⋅0=0
- Therefore, the torque in the most stable position is:
τ=0
Step 4: Calculate Magnetic Potential Energy at θ=0∘
- Substitute θ=0∘ into the potential energy equation:
Um=−mBcos(0∘)=−mB⋅1=−mB
- Therefore, the magnetic potential energy in the most stable position is:
Um=−mB
Final Answer
- The torque and magnetic potential energy of a magnetic dipole in its most stable position in a uniform magnetic field are:
Torque=0,Magnetic Potential Energy=−mB
- Torque (τ): The torque experienced by a magnetic dipole in a magnetic field is given by the formula:
τ=→m×→B
or in magnitude form:
τ=mBsinθ
where m is the magnetic moment, B is the magnetic field strength, and θ is the angle between →m and →B.
Um=−→m⋅→B
or in magnitude form:
Um=−mBcosθ
- The most stable position of a magnetic dipole in a magnetic field occurs when the dipole is aligned with the field, which corresponds to θ=0∘.
- Substitute θ=0∘ into the torque equation:
τ=mBsin(0∘)=mB⋅0=0
- Therefore, the torque in the most stable position is:
τ=0
- Substitute θ=0∘ into the potential energy equation:
Um=−mBcos(0∘)=−mB⋅1=−mB
- Therefore, the magnetic potential energy in the most stable position is:
Um=−mB
- The torque and magnetic potential energy of a magnetic dipole in its most stable position in a uniform magnetic field are:
Torque=0,Magnetic Potential Energy=−mB
Topper's Solved these Questions
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - MAGNETISM AND GAUSS S LAW
2 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - THE EARTH S MAGNETISM
19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Assertion And Reason
15 Videos
- MOVING CHARGES AND MAGNETISM BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - Assertion And Reason
15 Videos
Similar Questions
Knowledge Check
The work done in moving a dipole from its most stable to most unstable position in a 0.09 T uniform magnetic field is (dipole moment of this dipole = 0.5Am2)
A0.07JB0.08JC0.09JD0.1J
The potential enargy of bar magnet of magnetic moment 8Am2 placed in a uniform magnetic field of 2 T at an angle of 120∘ is equal to
View SolutionState whether the magnetic field lines in part (a) represent a uniform magnetic field or non uniform magnetic field ?
View SolutionWhat is the direction of the force acting on a charged particle q, moving with a velocity →v a uniform magnetic field →B ?
View SolutionA bar magnetic dipole moment M is cut into two equal pieces as shown, magnetic moment of each piece is
View SolutionWhen a current carrying coil is placed in a uniform magnetic field with its magnetic moment anti-parallel to the field.
View SolutionWhen a current carrying coil is placed in a uniform magnetic field with its magnetic moment anti-parallel to the field.
View SolutionA bar magnet of lenth l and magnetic dipole moment 'M' is bent in the form of an arc as shown in figure. The new magnetic dipole moment will be
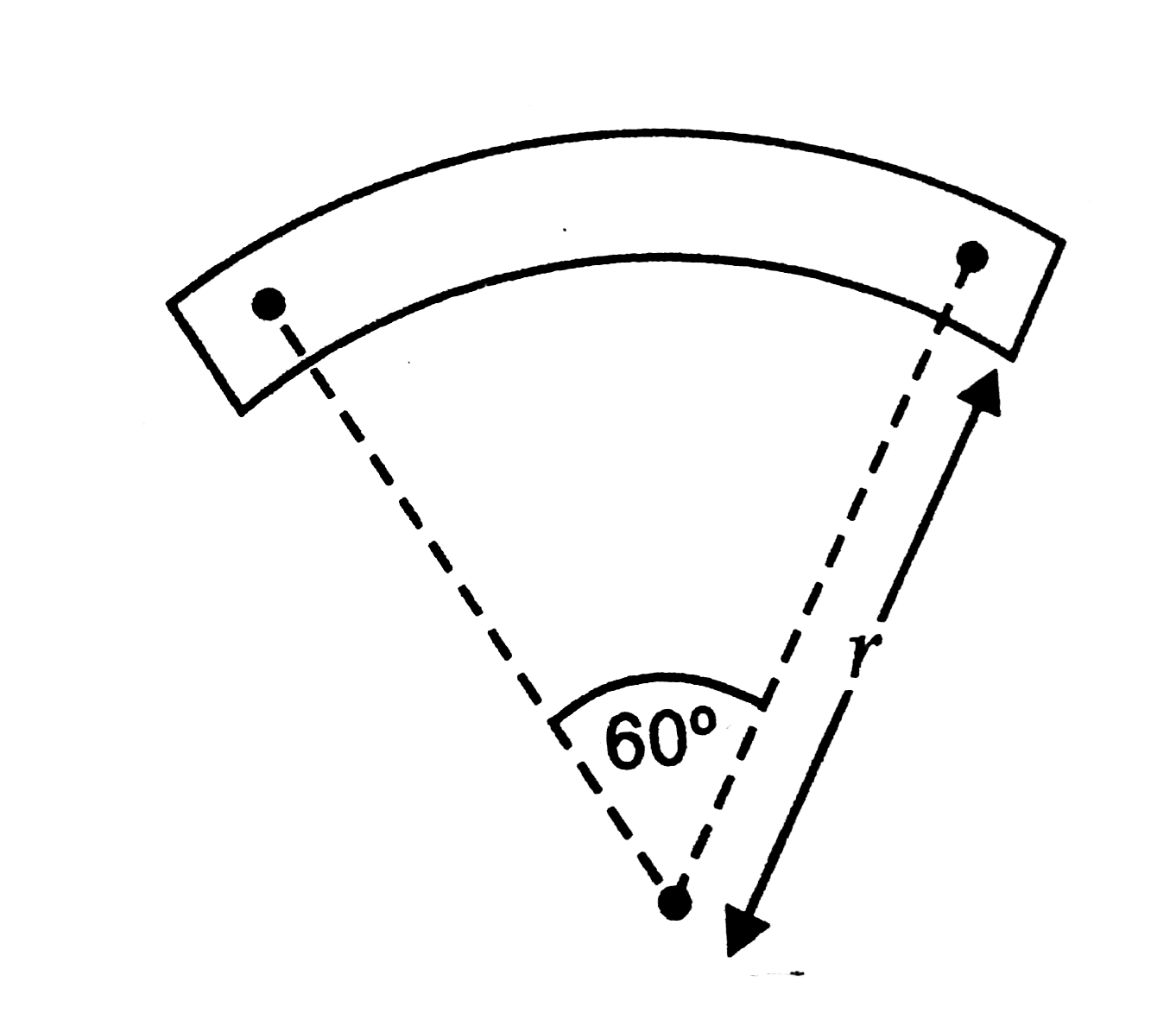
View Solution

