Three particle of mass m each are placed at the three corners of an equilateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.

A

$- \frac{3}{2} \frac{G M^{2}}{a^{2}}$

B

$\frac{- 3 G M^{2}}{a^{2}}$

C

$\frac{3}{2} \frac{G M^{2}}{a^{2}}$

D

$\frac{3 G M^{2}}{l}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:C

To find the work done on the system of three particles of mass $m$ each, placed at the corners of an equilateral triangle with side length $a$ , when the side length is increased to $2 a$ , we will follow these steps:
Step 1: Calculate the Initial Potential Energy
The gravitational potential energy $U$ between two masses $m_{1}$ and $m_{2}$ separated by a distance $r$ is given by:
$U = - \frac{G m_{1} m_{2}}{r}$
For our system, we have three particles (let's call them $P_{1}, P_{2}, P_{3}$ ) at the corners of the triangle. The initial potential energy $U_{i}$ when the side length is $a$ can be calculated as follows:
- The potential energy between $P_{1}$ and $P_{2}$ is:
$U_{12} = - \frac{G m^{2}}{a}$
- The potential energy between $P_{1}$ and $P_{3}$ is:
$U_{13} = - \frac{G m^{2}}{a}$
- The potential energy between $P_{2}$ and $P_{3}$ is:
$U_{23} = - \frac{G m^{2}}{a}$
Thus, the total initial potential energy $U_{i}$ is:
$U_{i} = U_{12} + U_{13} + U_{23} = - \frac{G m^{2}}{a} - \frac{G m^{2}}{a} - \frac{G m^{2}}{a} = - \frac{3 G m^{2}}{a}$
Step 2: Calculate the Final Potential Energy
Now, we need to calculate the final potential energy $U_{f}$ when the side length is increased to $2 a$ :
- The potential energy between $P_{1}$ and $P_{2}$ is:
$U_{12}^{'} = - \frac{G m^{2}}{2 a}$
- The potential energy between $P_{1}$ and $P_{3}$ is:
$U_{13}^{'} = - \frac{G m^{2}}{2 a}$
- The potential energy between $P_{2}$ and $P_{3}$ is:
$U_{23}^{'} = - \frac{G m^{2}}{2 a}$
Thus, the total final potential energy $U_{f}$ is:
$U_{f} = U_{12}^{'} + U_{13}^{'} + U_{23}^{'} = - \frac{G m^{2}}{2 a} - \frac{G m^{2}}{2 a} - \frac{G m^{2}}{2 a} = - \frac{3 G m^{2}}{2 a}$
Step 3: Calculate the Work Done
The work done $W$ on the system is equal to the change in potential energy, which can be calculated as:
$W = U_{f} - U_{i}$
Substituting the values we found:
$W = (- \frac{3 G m^{2}}{2 a}) - (- \frac{3 G m^{2}}{a})$
This simplifies to:
$W = - \frac{3 G m^{2}}{2 a} + \frac{3 G m^{2}}{a} = - \frac{3 G m^{2}}{2 a} + \frac{6 G m^{2}}{2 a} = \frac{3 G m^{2}}{2 a}$
Thus, the work done on the system to increase the sides of the triangle to $2 a$ is:
$W = \frac{3 G m^{2}}{2 a}$
Final Answer
The work done is:
$\frac{3 G m^{2}}{2 a}$

|

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Three point masses each of mass m are placed at the corners of an equilateral triangle of side b. The moment of inertia of the system about an axis coinciding with one side of the triangle is

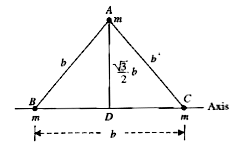A $3 m b^{2}$
B $m b^{2}$
C $(3 / 4) m b^{2}$
D $(2 / 3) m b^{2}$

DC PANDEY ENGLISH-GRAVITATION-Level 1 Single Correct

Home
Ask Doubt
Profile