Three particles of masses 1kg, 2kg and 3kg are placed at the corners A, B and C respectively of an equilateral triangle ABC of edge 1m. Find the distance of their centre of mass from A.
The correct Answer is:A
To find the distance of the center of mass from point A in the given problem, we will follow these steps:
Step 1: Set the Coordinate System
We will place point A at the origin (0, 0). The coordinates of points B and C will be determined based on the geometry of the equilateral triangle.
- Coordinates:
- A (0, 0)
- B (1, 0) (since B is 1 meter to the right of A)
- C (0.5, √32) (the height of the triangle can be calculated using the formula for the height of an equilateral triangle)
Step 2: Identify Masses
The masses at each point are:
- mA=1kg at A
- mB=2kg at B
- mC=3kg at C
Step 3: Calculate the Center of Mass Coordinates
The coordinates of the center of mass (CM) can be calculated using the formula:
xcm=mAxA+mBxB+mCxCmA+mB+mC
Substituting the values:
xcm=(1⋅0)+(2⋅1)+(3⋅0.5)1+2+3=0+2+1.56=3.56=712m
Now for the y-coordinate:
ycm=mAyA+mByB+mCyCmA+mB+mC
Substituting the values:
ycm=(1⋅0)+(2⋅0)+(3⋅√32)1+2+3=0+0+3√326=3√326=√34m
Step 4: Calculate the Distance from A to the Center of Mass
The distance D from point A to the center of mass can be calculated using the Pythagorean theorem:
D=√x2cm+y2cm
Substituting the values we found:
D=
⎷(712)2+(√34)2
Calculating each term:
D=√49144+316
To add these fractions, we need a common denominator. The least common multiple of 144 and 16 is 144:
316=27144
Thus, we have:
D=√49+27144=√76144=√7612
We can simplify √76 as √4⋅19=2√19:
D=2√1912=√196m
Final Answer
The distance of the center of mass from point A is:
√196m
We will place point A at the origin (0, 0). The coordinates of points B and C will be determined based on the geometry of the equilateral triangle.
- A (0, 0)
- B (1, 0) (since B is 1 meter to the right of A)
- C (0.5, √32) (the height of the triangle can be calculated using the formula for the height of an equilateral triangle)
The masses at each point are:
- mA=1kg at A
- mB=2kg at B
- mC=3kg at C
The coordinates of the center of mass (CM) can be calculated using the formula:
The distance D from point A to the center of mass can be calculated using the Pythagorean theorem:
⎷(712)2+(√34)2
The distance of the center of mass from point A is:
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Exercise 11.2
6 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Exercise 11.3
7 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - MiscellaneousExamples
9 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
Similar Questions
Three particles of masses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
View SolutionFour particles of masses 1kg,2kg,3kg and 4kg are placed the four vertices A,B,C and D, respectively, of a square of side 1m. Find the position of centre of mass of the particles.
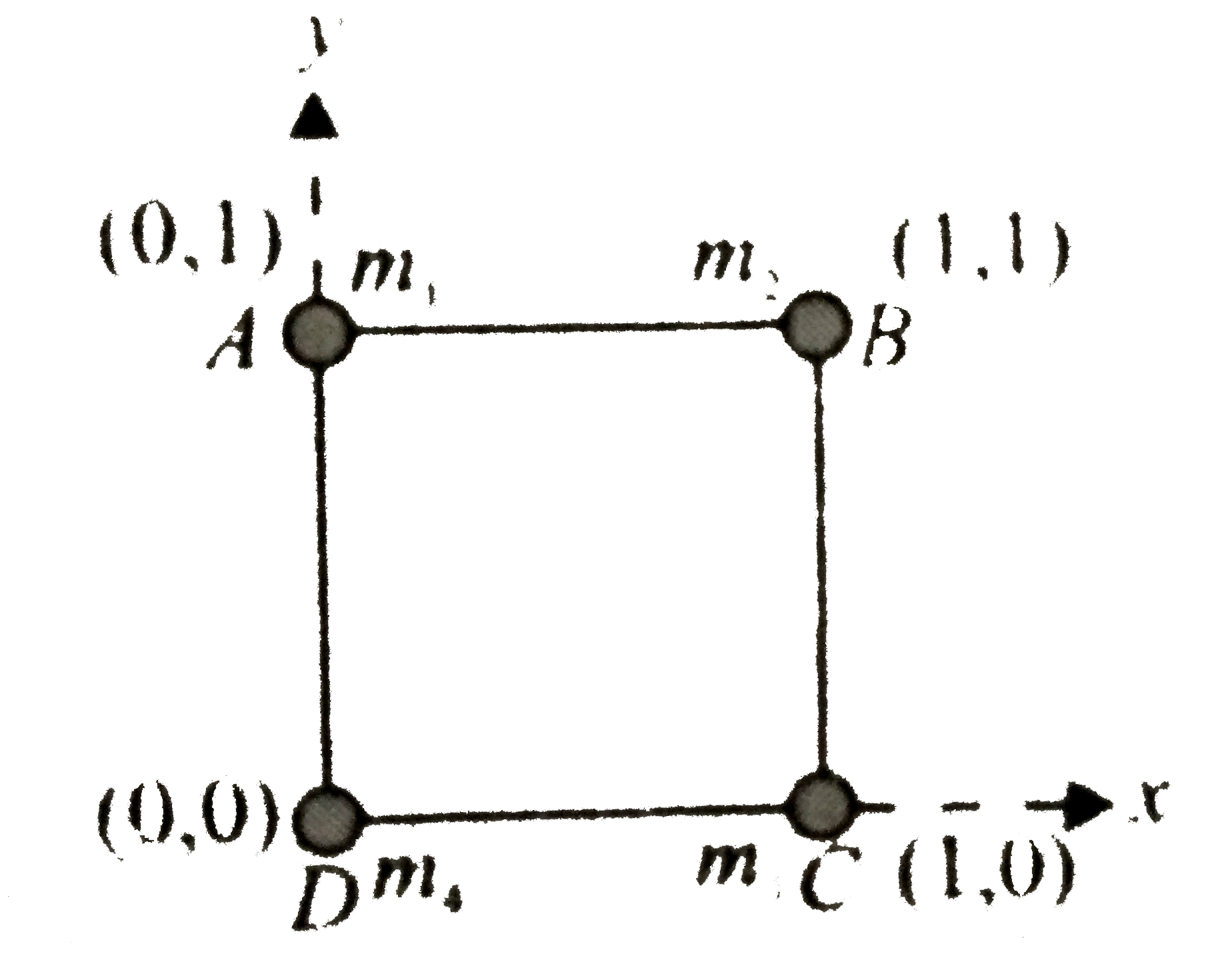
View Solution
Knowledge Check
Three particles of masses 1kg,32kg,and2kg are located the vertices of an equilateral triangle of side a. The x,y coordinates of the centre of mass are.
Aa. 5a9,2a3√3Bb. 2a3√3,5a9Cc. 5a9,2a√3Dd. 2a√3,5a9
4 Masses 8 kg, 2 kg, 4 kg and 2 kg are placed at the corners A, B, C, D respectively of a square ABCD of diagonal 80 cm. The distance of centre of mass from A will be
View SolutionFour particles of masses 1kg, 2kg, 3kg and 4kg are placed at the four vertices A,B,C and D of a square of side 1m. Find square of distance of their centre of mass from A.
View SolutionThree point masses m1=2Kg,m2=4kgandm3=6kg are kept at the three corners of an equilateral triangle of side 1 m. Find the locationo of their center of mass.
View SolutionFour particles of masses 1kg, 2kg, 3kg and 4kg are placed at the four vertices A, B, C and D of a square of side 1m. Find the position of centre of mass of the particles.
View SolutionFour particles of masses 1kg, 2kg, 3kg and 4kg are placed at the four vertices A, B, C and D of a square of side 1m. Find the position of centre of mass of the particles. Assume that D is situated at the origin.
View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :
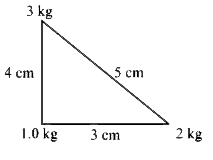
View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :

View Solution
