Two balls A and B are projected vertically upwards with different velocities. What is the relative acceleration between them?
To solve the problem of finding the relative acceleration between two balls A and B projected vertically upwards with different velocities, we can follow these steps:
Step 1: Understand the motion of the balls
Both balls A and B are projected vertically upwards. When objects are projected upwards, they experience a downward acceleration due to gravity.
Step 2: Identify the acceleration of each ball
The acceleration due to gravity (g) acts on both balls A and B. This acceleration is directed downwards and has a magnitude of approximately 9.81m/s2.
Step 3: Write the expression for relative acceleration
The relative acceleration of ball A with respect to ball B is given by the formula:
aAB=aA−aB
where aA is the acceleration of ball A and aB is the acceleration of ball B.
Step 4: Substitute the values of acceleration
Since both balls are under the influence of gravity, we have:
aA=−gandaB=−g
(Note: The negative sign indicates that the acceleration is directed downwards.)
Step 5: Calculate the relative acceleration
Substituting the values into the relative acceleration formula:
aAB=(−g)−(−g)=−g+g=0
Conclusion
The relative acceleration between balls A and B is:
aAB=0
This means that both balls A and B have the same acceleration due to gravity, and thus, their relative acceleration is zero.
---
Both balls A and B are projected vertically upwards. When objects are projected upwards, they experience a downward acceleration due to gravity.
The acceleration due to gravity (g) acts on both balls A and B. This acceleration is directed downwards and has a magnitude of approximately 9.81m/s2.
The relative acceleration of ball A with respect to ball B is given by the formula:
aAB=aA−aB
where aA is the acceleration of ball A and aB is the acceleration of ball B.
Since both balls are under the influence of gravity, we have:
aA=−gandaB=−g
(Note: The negative sign indicates that the acceleration is directed downwards.)
Substituting the values into the relative acceleration formula:
aAB=(−g)−(−g)=−g+g=0
The relative acceleration between balls A and B is:
aAB=0
Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Assertion And Reason
12 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Objective
45 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.8
5 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
Two balls of different masses are thrown in air with different velocities. While they are in air acceleration of centre of mass of the system. (neglect air resistance)?
View SolutionIf two particles of masses m1 and m2 are projected vertically upwards with speed v1 and v2, then the acceleration of the centre of mass of the system is
View Solution
Knowledge Check
A player throws a ball vertically upwards with velocity u. At highest point,
A1. both the velocity and acceleration of the ball are zero.B2. the velocity of the ball is u but its accelerationC3. the velocity of the ball is zero but its acceleration is g.D4. the velocity of the ball is u but its acceleration is g.
A particle is projected vertically upwards in vacuum with a speed u.
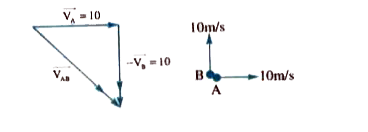
View SolutionTwo particles A and B are projected in air .A is thrown horizontally .B is thrown vertically up . What is the separation between them after 1 sec .
View SolutionA particle is projected vertically upwards with a velocity of 20m/sec. Find the time at which distance travelled is twice the displacement
View SolutionSTATEMENT - 1 : Two bodies projected vertically upward with different initial speeds cover same distances in last second of their upward journey.
and
STATEMENT - 2 : For uniformly accelerated motion, distance travelled in a time interval before coming to rest does not depend on initial speed.
View SolutionTwo bodies are thrown vertically upwards with their initial velocity in the ratio 2:3. Then the ratio of the maximum height attained by them is
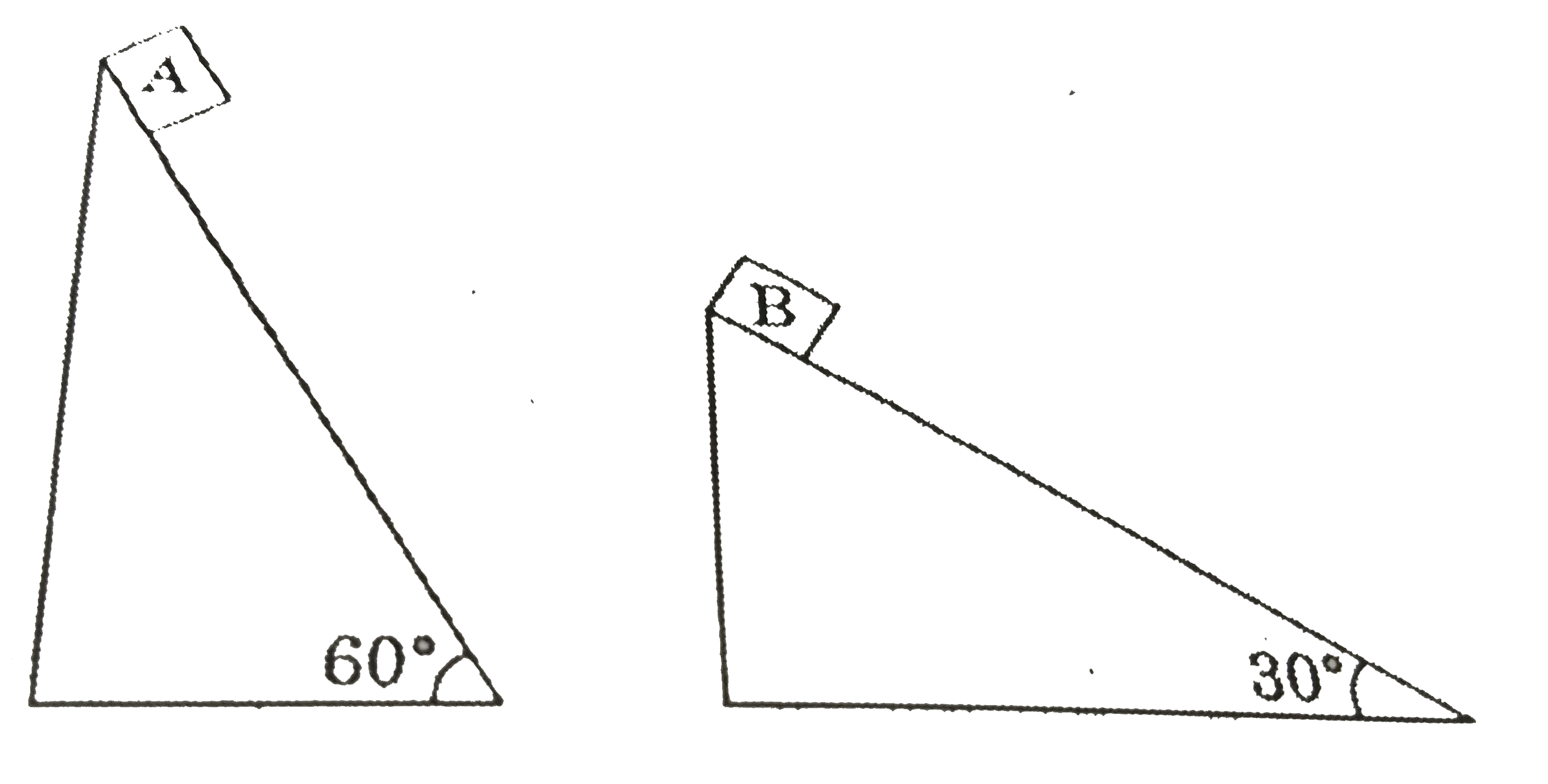
View SolutionTwo fixed frictionless inclined plane making an angle 30∘ and 60∘ with the vertical are shown in the figure. Two block A and B are placed on the two planes. What is the relative vertical acceleration of A with respect to B?
View SolutionA body is projected vertically upward from the surface of the earth, then the velocity-time graph is:-
View Solution
