An aeroplane has to go from a point A to another point B, 500km away due 30∘ east of north. Wind is blowing due north at a speed of 20m/s. The air-speed of the plane is 150m/s. (a) Find the direction in which the pilot should head the plane to reach the point B. (b) Find the time taken by the plane to go from A to B.
The correct Answer is:A, B
Applying sine law in ΔABC, we have
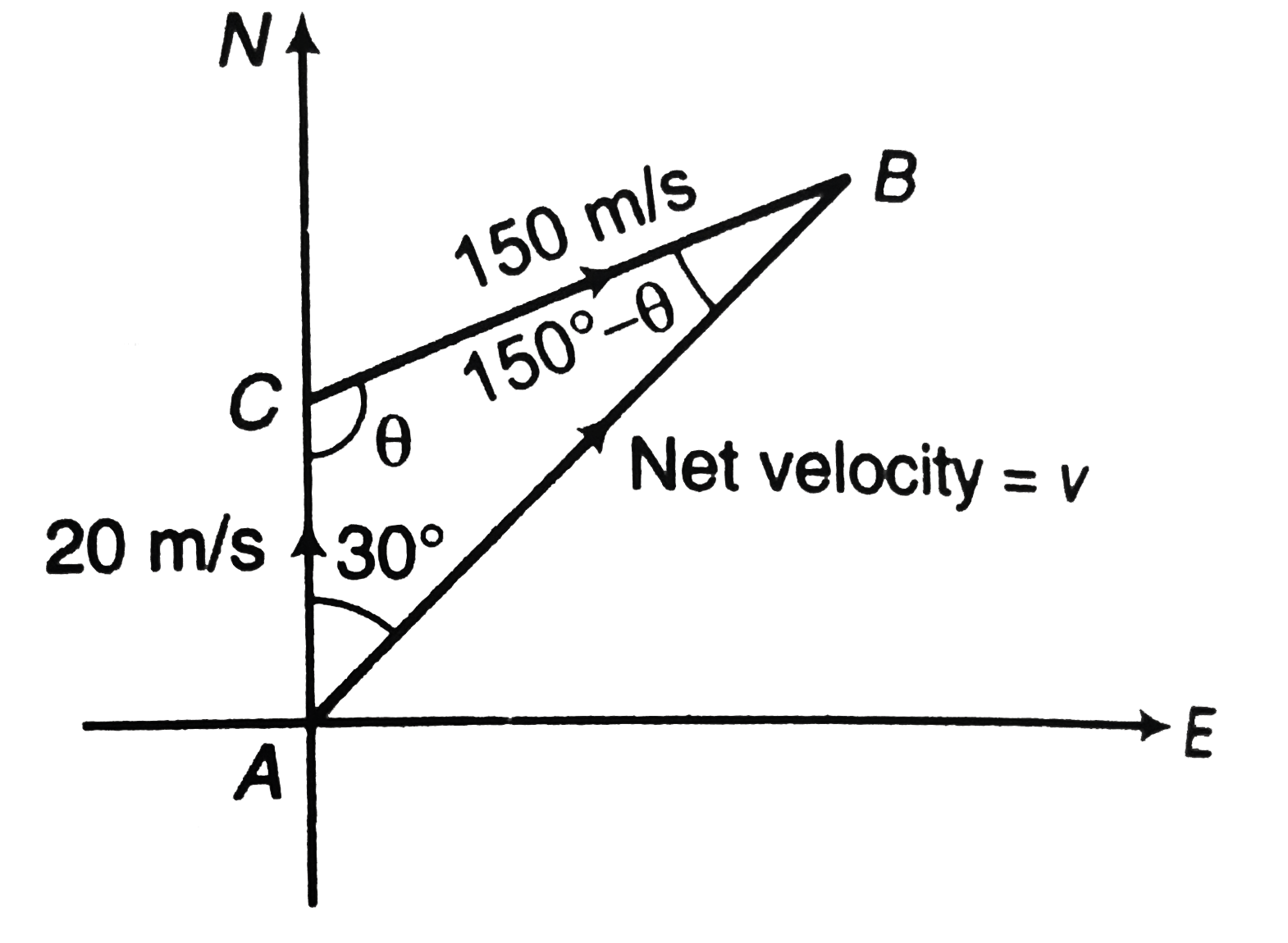
150sin30∘=vsinθ=20sin(150−θ)
From first and third we have,
sin(150−θ)=20sin30∘150=115
∴150−θ=sin−1(115)
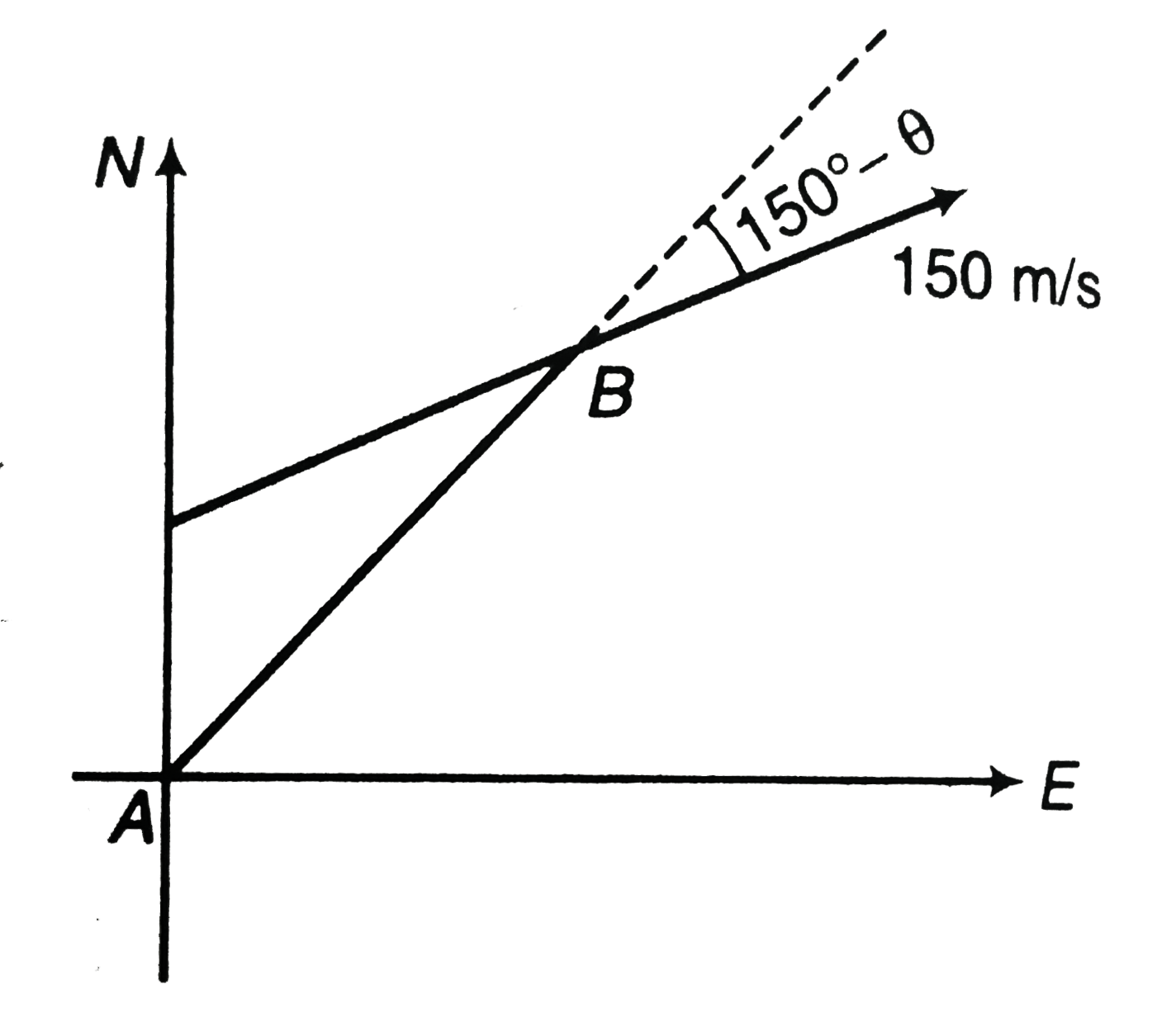
Hence, we can see that direction of 150 m//s is
150∘−θ[orsin−1(115)] east of the line AB
(b) 150∘−θ=sin−1(115)=3.8∘
∴θ=146.2∘
From first and second relation,
v=150sinθsin30∘
Substituting the values we get,
v=167m/s
∴t=ABv
=500×1000167×60min=49.9min
≈50min
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Assertion And Reason12videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Objective45videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.85videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises45videos
GRAVITATION
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
Similar Questions
An aeroplane has to go from a point P to another point Q, 1000 km away due north. Wind is blowing due east at a speed of 200km/h. The air speed of plane is 500km/h.
(a) Find the direction in which the pilot should head the plane to reach the point Q.
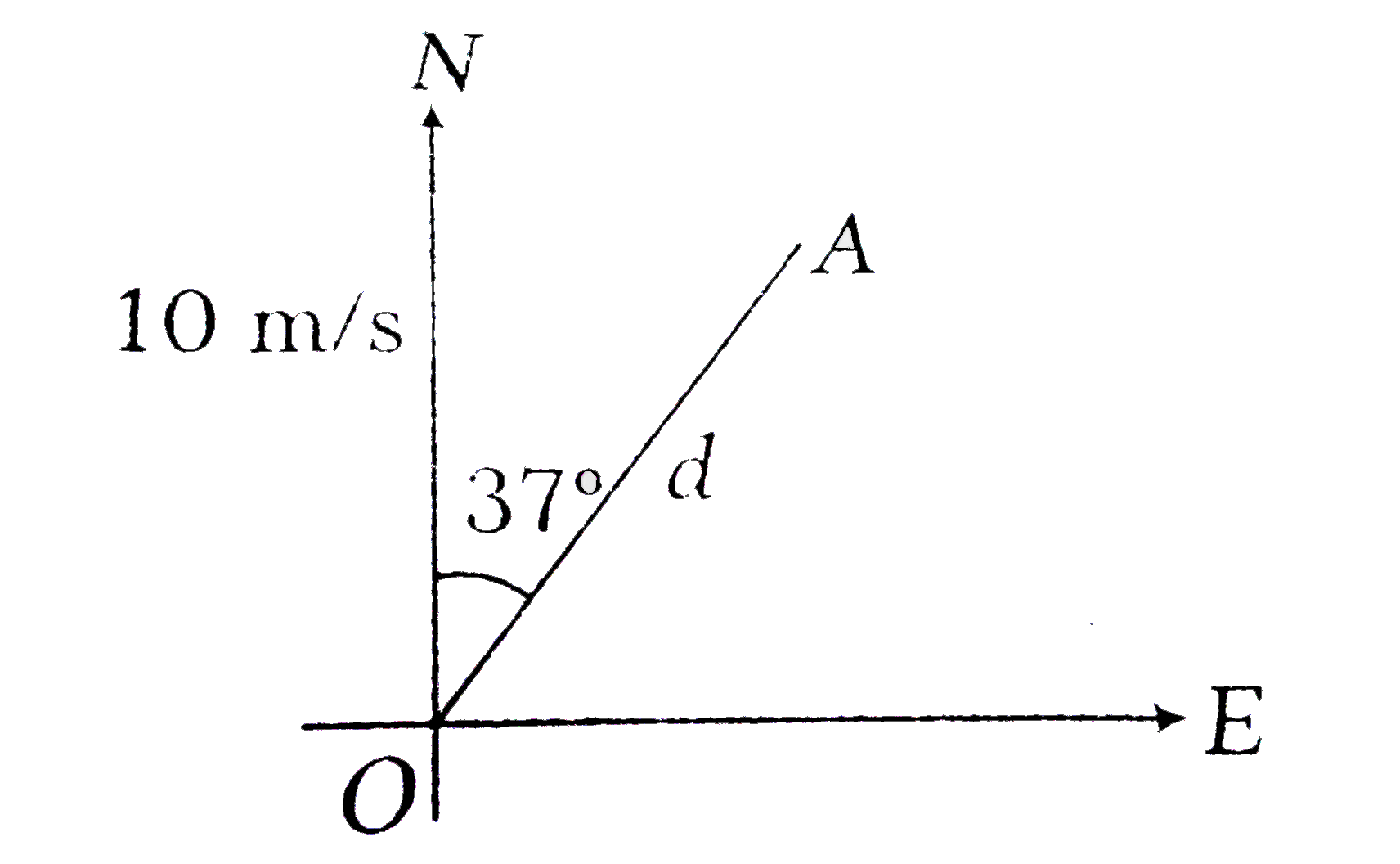
(b) Find the time taken by the plane to go from P to Q.View SolutionAn aeroplane has to go from a point O to another point A, at distance d due 37∘ East of North. A wind is blowing due North at a speed of 10ms−1. The air speed of the plane is v. (i) Find the direction in which the pilot should head the plane to reach the point A. (ii) Find the time taken by the plane to go from O to A.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A train of 150 m length is going towards north direction at a speed of 10 m/s. A bird flies at a speed of 5 m/s towards south direction parallel to the railway track. The time taken by the bird to cross the train is equal to
A20 secB16 secC12 secD10 sec
An aeroplane takes off from Mumbai to Delhi with velocity 50 kph at an angle 30∘ north of east. Wind is blowing at 25 kph from north to south. What is the resultant displacement of aeroplane in 2 hrs. (in km)
View SolutionA ship is travelling due east at a speed of 15km/h. Find the speed of a boat heading 30∘ east of north if it appears always due north from the ship.
View SolutionRam moves in east direction at a speed of 6 m/s and Shyam moves 30∘ east of north at a speed of 6 m/s. The magnitude of their relative velocity is
View SolutionThe wind is blowing due south with speed of 3m/sec. How fast should a car travel due east in order that the wind shall hasve a speed of 5m/sec relative to the car.
View SolutionA 210 meter long train is moving due north at a of 25 m/s. a small bird is flying due south a little above the train with speed 5 m/s. The time taken by the bird to cross the train is
View SolutionAn aeroplane pilot wishes to fly due west A wind of 100kmh−1 is blowing towards south.
(a) If the speed of the plane (its speed in still air) is 300kmh−1 in which direction should the pilot head ?
(b) What is the speed of the plane with respect tom ground ? Illustrate with a vector diagram.View SolutionAn aeroplane has a velocity of 110 m/s directed due north and is subjected to a wind blowing from west to east at a speed of 40 m/s . Calculate the actual velocity of the aeroplane relative to the earth .
View Solution

