Two balls of masses 10 kg and 20 kg approach each other with velocities 30 m/s and 20 m/s respectively . Calculate their velocities after they undergo a perfectly eleastic collision .
To solve the problem of two balls undergoing a perfectly elastic collision, we will use the principles of conservation of momentum and the properties of elastic collisions.
1. Identify the Given Data:
- Mass of ball 1 (m1) = 10 kg
- Initial velocity of ball 1 (u1) = 30 m/s (approaching)
- Mass of ball 2 (m2) = 20 kg
- Initial velocity of ball 2 (u2) = -20 m/s (approaching from the opposite direction)
2. Conservation of Momentum:
The total momentum before the collision is equal to the total momentum after the collision.
m1u1+m2u2=m1v1+m2v2
Substituting the values:
10×30+20×(−20)=10v1+20v2
This simplifies to:
300−400=10v1+20v2
−100=10v1+20v2(Equation 1)
3. Elastic Collision Condition:
For perfectly elastic collisions, the relative velocity of approach is equal to the relative velocity of separation:
u1−u2=v2−v1
Substituting the values:
30−(−20)=v2−v1
This simplifies to:
50=v2−v1(Equation 2)
4. Solving the Equations:
From Equation 2, we can express v1 in terms of v2:
v1=v2−50
Now, substitute v1 into Equation 1:
−100=10(v2−50)+20v2
Expanding this gives:
−100=10v2−500+20v2
Combining like terms:
−100=30v2−500
Adding 500 to both sides:
400=30v2
Dividing by 30:
v2=40030=13.33m/s
5. Finding v1:
Now substitute v2 back into the equation for v1:
v1=13.33−50=−36.67m/s
Final Velocities:
- Final velocity of ball 1 (v1) = -36.67 m/s (indicating it moves in the opposite direction)
- Final velocity of ball 2 (v2) = 13.33 m/s
- Mass of ball 1 (m1) = 10 kg
- Initial velocity of ball 1 (u1) = 30 m/s (approaching)
- Mass of ball 2 (m2) = 20 kg
- Initial velocity of ball 2 (u2) = -20 m/s (approaching from the opposite direction)
The total momentum before the collision is equal to the total momentum after the collision.
m1u1+m2u2=m1v1+m2v2
Substituting the values:
10×30+20×(−20)=10v1+20v2
This simplifies to:
300−400=10v1+20v2
−100=10v1+20v2(Equation 1)
For perfectly elastic collisions, the relative velocity of approach is equal to the relative velocity of separation:
u1−u2=v2−v1
Substituting the values:
30−(−20)=v2−v1
This simplifies to:
50=v2−v1(Equation 2)
From Equation 2, we can express v1 in terms of v2:
v1=v2−50
Now, substitute v1 into Equation 1:
−100=10(v2−50)+20v2
Expanding this gives:
−100=10v2−500+20v2
Combining like terms:
−100=30v2−500
Adding 500 to both sides:
400=30v2
Dividing by 30:
v2=40030=13.33m/s
Now substitute v2 back into the equation for v1:
v1=13.33−50=−36.67m/s
- Final velocity of ball 1 (v1) = -36.67 m/s (indicating it moves in the opposite direction)
- Final velocity of ball 2 (v2) = 13.33 m/s
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - PRACTICE PROBLEMS27videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Conceptual Questions20videos
WORK, ENERGY AND POWER
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST14videos
WAVES
Similar Questions
A 10kg ball and a 20kg ball approach each other with velocities 20m/s and 10m/s respectively. What are their velocities after collision, if the collision is perfectly elastic ?
View SolutionA 10 kg ball and 20 kg ball approach each other with velocities 20ms−1 and 10ms−1 respectively . What are their velocities after collision if the collision is perfectly elastic ?
View Solution
Knowledge Check
- Question 1 - Select One
Two steel balls A and B of mass 10 kg and 10 g rolls towards each other with 5m/s and 1 m/s respectively on a smooth floor. After collision, with what speed B moves [perfectly elastic collision]?
A8 m/sB10 m/sC11 m/sDZero - Question 2 - Select One
Two steel balls A and B of mass 10 kg and 10 g rolls towards each other with 5 m/s and 1 m/s respectively on a smooth floor. After collision, with what speed B moves (perfectly elastic collision)?
A8msB10msC11msDZero - Question 3 - Select One
Two balls of masses m each are moving at right angle to each other with velocities 6 m/s and 8 m/s respectively. If collision between them is perfectly inelastic, the velocity of combined mass is
A15 m/sB10m/sC5 m/sD2.5 m/s
Two bodies of masses 2 kg and 4 kg are moving with velocities 2 m/s and 10 m/s respectively along same direction. Then the velocity of their centre of mass will be
View SolutionTwo balls of masses 2kg and 4kg are moved towards each other with velocities 4m/s and 2m/s, respectively, on a frictionless surface. After colliding, the 2kg ball returns back with velocity 2m/s. then find
a. velocity of the 4kg after collision
b. coefficient of restitution e,
c. impulse of deformation JD
d. maximum potential energy of deformation,
e. impulse of reformation JR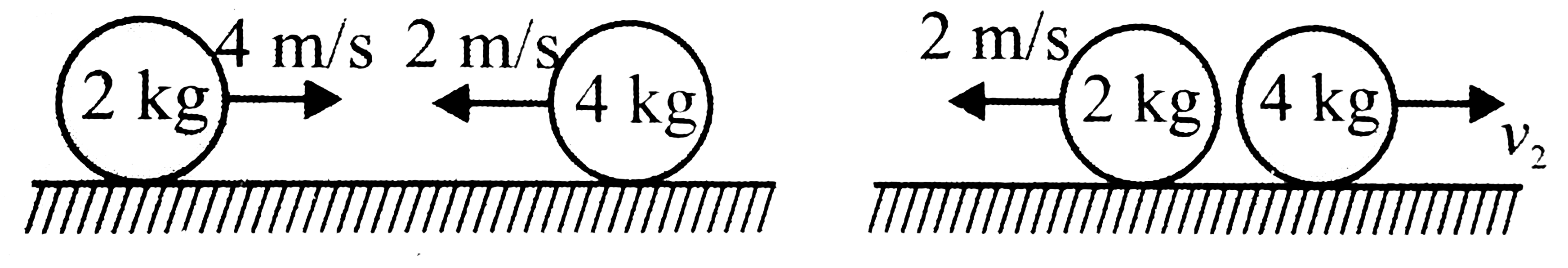 View Solution
View SolutionTwo perfectly elastic particles A and B of equal masses travelling along a line joining them with velocities 15m/s and 10m/s respectively collide. Their velocities after the elastic collision will be (in m/s) respectively
View SolutionTwo spheres of masses 1 kg and 2 kg are moving with velocities of 10 m/s and 5 m/s respectively in the same direction. After the collision the velocity of the lighter sphere decreases by 4 m/s. What is the coefficient of restitution?
View SolutionTwo identical balls each of mass 4 kg are moving towards each other with speeds 2 m/s and 3 m/s respectively. They undergo head on perfectly elastic collision. Then impulse imparted by one ball on other is
View Solution

