Two identical balls each of mass 4 kg are moving towards each other with speeds 2 m/s and 3 m/s respectively. They undergo head on perfectly elastic collision. Then impulse imparted by one ball on other is
12 Ns
8 Ns
20 Ns
40 Ns
The correct Answer is:C
To solve the problem of finding the impulse imparted by one ball on the other during a perfectly elastic collision, we can follow these steps:
Step 1: Understand the Problem
We have two identical balls, each with a mass of 4 kg. One ball is moving towards the right with a speed of 2 m/s, and the other is moving towards the left with a speed of 3 m/s. We need to find the impulse imparted by one ball on the other after they collide elastically.
Step 2: Set Up the Conservation of Momentum
In a perfectly elastic collision, the total momentum before the collision is equal to the total momentum after the collision.
Let:
- Mass of ball 1 (m1) = 4 kg, initial velocity (u1) = 2 m/s
- Mass of ball 2 (m2) = 4 kg, initial velocity (u2) = -3 m/s (negative because it's moving in the opposite direction)
The total initial momentum (p_initial) can be calculated as:
pinitial=m1⋅u1+m2⋅u2
pinitial=4⋅2+4⋅(−3)
pinitial=8−12=−4kg m/s
Step 3: Set Up the Conservation of Kinetic Energy
In a perfectly elastic collision, the total kinetic energy before the collision is equal to the total kinetic energy after the collision.
The total initial kinetic energy (KE_initial) can be calculated as:
KEinitial=12m1u21+12m2u22
KEinitial=12⋅4⋅(22)+12⋅4⋅(−3)2
KEinitial=4⋅2+4⋅4.5=8+18=26J
Step 4: Use the Equations for Elastic Collisions
For elastic collisions, we can use the following equations:
1. v1+v2=u1+u2 (conservation of momentum)
2. v2−v1=−(u2−u1) (relative velocity)
Let v1 and v2 be the final velocities of ball 1 and ball 2, respectively.
From the first equation:
v1+v2=2−3=−1(1)
From the second equation:
v2−v1=−(−3−2)=5(2)
Step 5: Solve the Equations
Now we have two equations:
1. v1+v2=−1
2. v2−v1=5
From equation (1):
v2=−1−v1
Substituting into equation (2):
(−1−v1)−v1=5
−1−2v1=5
−2v1=6
v1=−3m/s
Now substituting back to find v2:
v2=−1−(−3)=2m/s
Step 6: Calculate the Impulse
Impulse (J) is defined as the change in momentum. For ball 2:
J=m2(v2−u2)
J=4(2−(−3))
J=4(2+3)=4⋅5=20Ns
Final Answer
The impulse imparted by one ball on the other is 20 Ns.
---
We have two identical balls, each with a mass of 4 kg. One ball is moving towards the right with a speed of 2 m/s, and the other is moving towards the left with a speed of 3 m/s. We need to find the impulse imparted by one ball on the other after they collide elastically.
In a perfectly elastic collision, the total momentum before the collision is equal to the total momentum after the collision.
- Mass of ball 1 (m1) = 4 kg, initial velocity (u1) = 2 m/s
- Mass of ball 2 (m2) = 4 kg, initial velocity (u2) = -3 m/s (negative because it's moving in the opposite direction)
pinitial=m1⋅u1+m2⋅u2
pinitial=4⋅2+4⋅(−3)
pinitial=8−12=−4kg m/s
In a perfectly elastic collision, the total kinetic energy before the collision is equal to the total kinetic energy after the collision.
KEinitial=12m1u21+12m2u22
KEinitial=12⋅4⋅(22)+12⋅4⋅(−3)2
KEinitial=4⋅2+4⋅4.5=8+18=26J
For elastic collisions, we can use the following equations:
1. v1+v2=u1+u2 (conservation of momentum)
2. v2−v1=−(u2−u1) (relative velocity)
v1+v2=2−3=−1(1)
v2−v1=−(−3−2)=5(2)
Now we have two equations:
1. v1+v2=−1
2. v2−v1=5
v2=−1−v1
(−1−v1)−v1=5
−1−2v1=5
−2v1=6
v1=−3m/s
v2=−1−(−3)=2m/s
Impulse (J) is defined as the change in momentum. For ball 2:
J=m2(v2−u2)
J=4(2−(−3))
J=4(2+3)=4⋅5=20Ns
The impulse imparted by one ball on the other is 20 Ns.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - A)
67 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - B)
39 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-J (AAKASH CHALLENGERS QUESTIONS)
14 Videos
- WAVESBOOK - AAKASH INSTITUTECHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
Two balls of masses 10 kg and 20 kg approach each other with velocities 30 m/s and 20 m/s respectively . Calculate their velocities after they undergo a perfectly eleastic collision .
View SolutionTwo identical balls are approaching towards each other on a straight line with velocity 2m/s and 4m/s, respectiely. Find the final velocities after elastic collision between them.
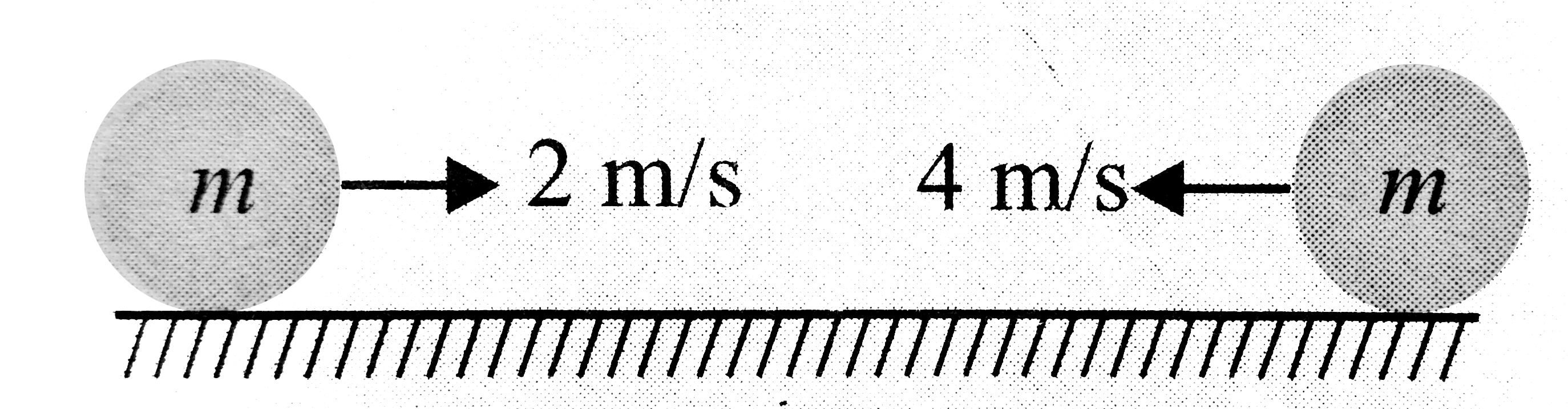
View Solution
Knowledge Check
Two identical balls moving in opposite directions with speed 20 m/s and 25 m/s undergo head on perfectly inelastic collision. The speed of combined mass after collision is
A20 m/sB22.5 m/sC25 m/sD2.5 m/sTwo identical balls moving in opposite direction with speed 20 m/s and 25 m/s undergo head on perfectly inelastic collision. The spped of combined mass after collision is
A20msB22.5msC25msD2.5msTwo balls of masses m each are moving at right angle to each other with velocities 6 m/s and 8 m/s respectively. If collision between them is perfectly inelastic, the velocity of combined mass is
A15 m/sB10m/sC5 m/sD2.5 m/s
Two particles A and B of equal size but of masses m kg and 2 m kg are moving directly towards each other with speeds 21 m/s and 4 m/s respectively. After collision, A moves with a speed of 1 m/s in the original direction. Then:
View SolutionTwo balls each of mass 0.25kg are moving towards each other in a straight line,one at 3m/s the other at 1m/s collide.The balls stick together after the collision.The magnitude of the final velocity of the combined mass in ms−1 is?
View SolutionA ball A of mass 3 kg and a ball B of mass 4 kg are moving alogn the same straight line with speeds of 7m/s and 5 m/s respectively. They approach each other and collide. What is the speed of B after collision, If the coefficient of restitution is 34?
View SolutionTwo steel balls A and B of mass 10 kg and 10 g rolls towards each other with 5m/s and 1 m/s respectively on a smooth floor. After collision, with what speed B moves [perfectly elastic collision]?
View SolutionTwo steel balls A and B of mass 10 kg and 10 g rolls towards each other with 5 m/s and 1 m/s respectively on a smooth floor. After collision, with what speed B moves (perfectly elastic collision)?
View Solution
