Two equal masses each in are hung from a balance whose scale pans differ in vertical height by h. The error in weighing in terms of density of the earth ρ is
πGρmh
12πGρmh
83πGρmh
43πGρmh
The correct Answer is:C
To solve the problem of determining the error in weighing due to the difference in vertical height h of the scale pans, we can follow these steps:
Step 1: Understanding the Problem
We have two equal masses m hanging from a balance, but the scale pans are at different heights. This difference in height affects the gravitational acceleration experienced by the masses.
Step 2: Gravitational Acceleration Change
The gravitational acceleration at a height h above the Earth's surface can be expressed as:
g′=g(1−hR)
where g is the standard gravitational acceleration at the surface, and R is the radius of the Earth.
Step 3: Calculate the Weights
The weight of the mass at the lower pan (height h) is:
W1=mg
The weight of the mass at the higher pan (height h) is:
W2=mg′=mg(1−hR)
Step 4: Determine the Error in Weighing
The error in weighing, E, can be defined as the difference between the two weights:
E=W2−W1=mg(1−hR)−mg
E=mg(−hR)=−mghR
Step 5: Substitute the Value of g
Using the relation g=43πR3ρ (where ρ is the density of the Earth), we can substitute for g:
E=−m(43πR3ρ)hR
E=−43πR2ρhm
Step 6: Final Expression for Error
The error in weighing can be expressed as:
E=−43πR2ρhm
This indicates that the error is proportional to the height difference h, the density of the Earth ρ, and the mass m.
Conclusion
The final expression for the error in weighing due to the height difference h is:
E=−83πGmρh
We have two equal masses m hanging from a balance, but the scale pans are at different heights. This difference in height affects the gravitational acceleration experienced by the masses.
The gravitational acceleration at a height h above the Earth's surface can be expressed as:
g′=g(1−hR)
where g is the standard gravitational acceleration at the surface, and R is the radius of the Earth.
The weight of the mass at the lower pan (height h) is:
W1=mg
The weight of the mass at the higher pan (height h) is:
W2=mg′=mg(1−hR)
The error in weighing, E, can be defined as the difference between the two weights:
E=W2−W1=mg(1−hR)−mg
E=mg(−hR)=−mghR
Using the relation g=43πR3ρ (where ρ is the density of the Earth), we can substitute for g:
E=−m(43πR3ρ)hR
E=−43πR2ρhm
The error in weighing can be expressed as:
E=−43πR2ρhm
This indicates that the error is proportional to the height difference h, the density of the Earth ρ, and the mass m.
The final expression for the error in weighing due to the height difference h is:
E=−83πGmρh
Topper's Solved these Questions
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Multiple Correct
24 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Assertion- Reasoning
13 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Subjective
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
Similar Questions
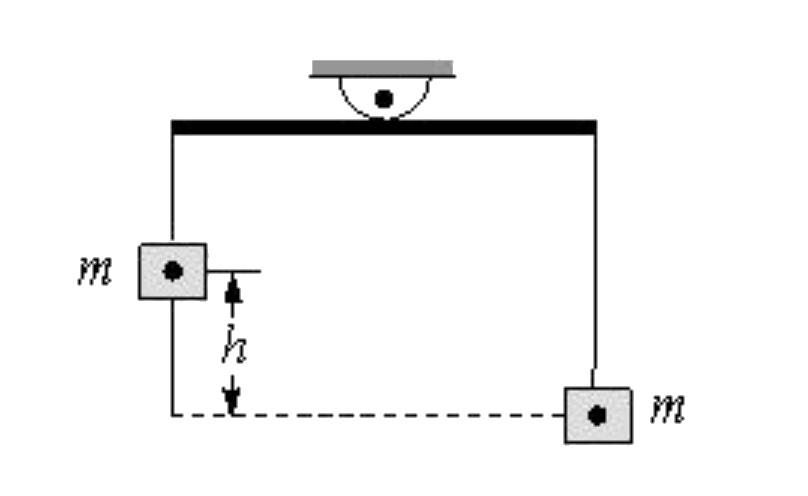
Two identical blocks of mass m are suspended from a beam balance whose scale pans differ in vertical height by h(h<<R), if R and ρ are the radius and density of the earth, then the error in weighing is
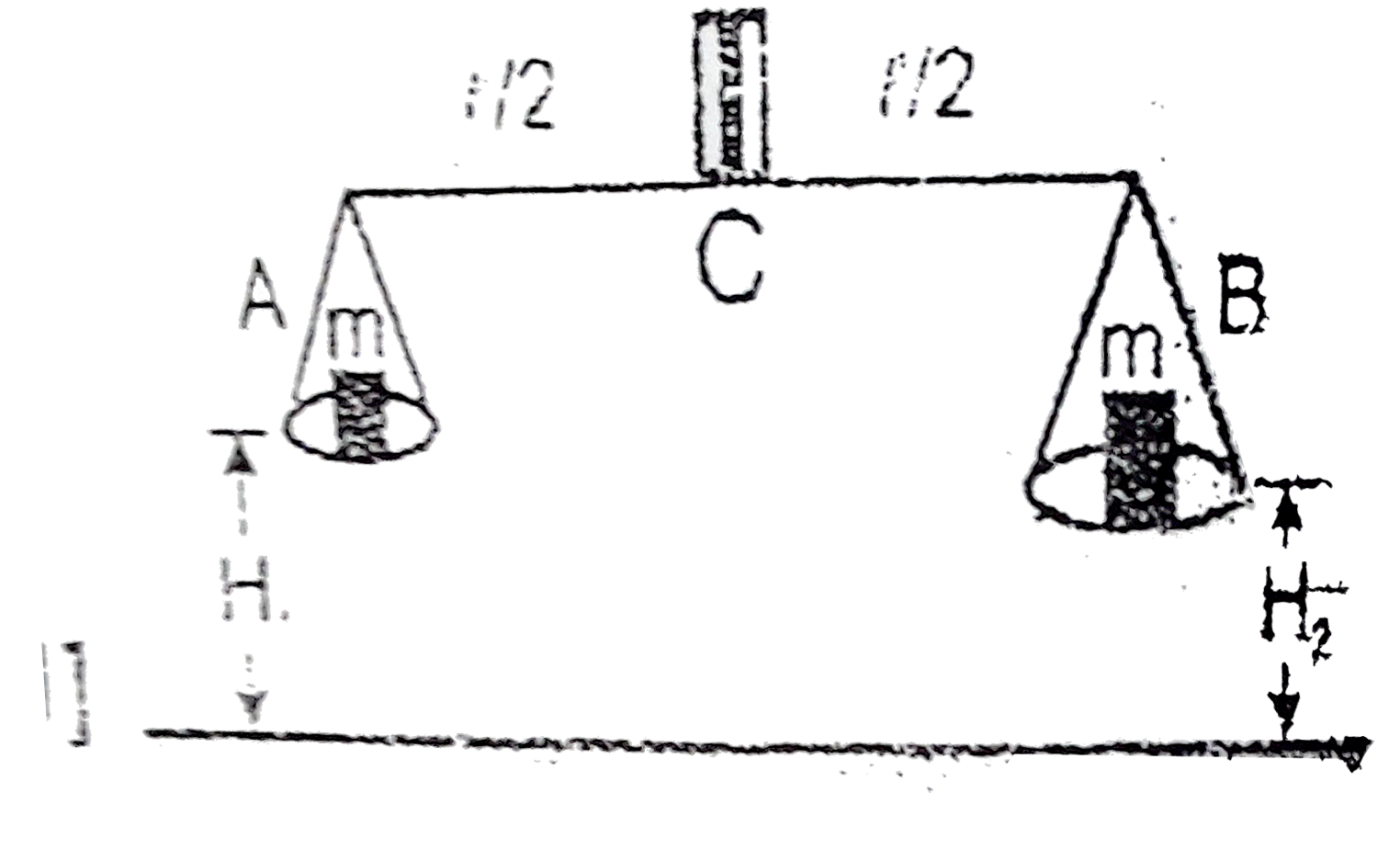
View SolutionTwo blocks of masses m each are hung from a balances as shown in the figure. The scale pan A is at height H1 whereas scale pan B is at height H2 Net torque of weights acting on the system about point C will be (length of the rod is l and H1 & H2 are lt ltR ) (H1>H2)
 .
.
View SolutionTwo objects of equal mass rest on the opposite pans of an arm balance. Does the scale remain balance when it is accelerated up or down in a life ?
View SolutionThere are two bodies A and B of same mass. A is placed near equator of earth and B is placed at a height h above the pole of earth. if both the bodies weighs equally. Find h in terms of radius R of earth, angular speed ω of earth and g acceleration due to gravity close to earth.
View SolutionOn the opposite sides of vertical vessel filled with water, two identical holes are opened. If area of cross-section for each is a and difference of height of these two is h and p is density of water, then force on the vessel is
View SolutionTwo vertical plates submerged partially in a wetting liquid form a wedge with a very small angle δφ. The edge of this wedge is vertical. The density of the liquid is ρ, its surface tension is α, the contact angle is θ. Find the height h, to which the liquid rises, as a function of the distance x from the edge.
View SolutionA rock is found to weigh w in air when suspended from a spring scale. When completely submerged in water while attached to the spring scale, it weighs wsubmerged as shown in Fig. Find the density of the rock ρrock in terms of Rock the scale reading and the density of water
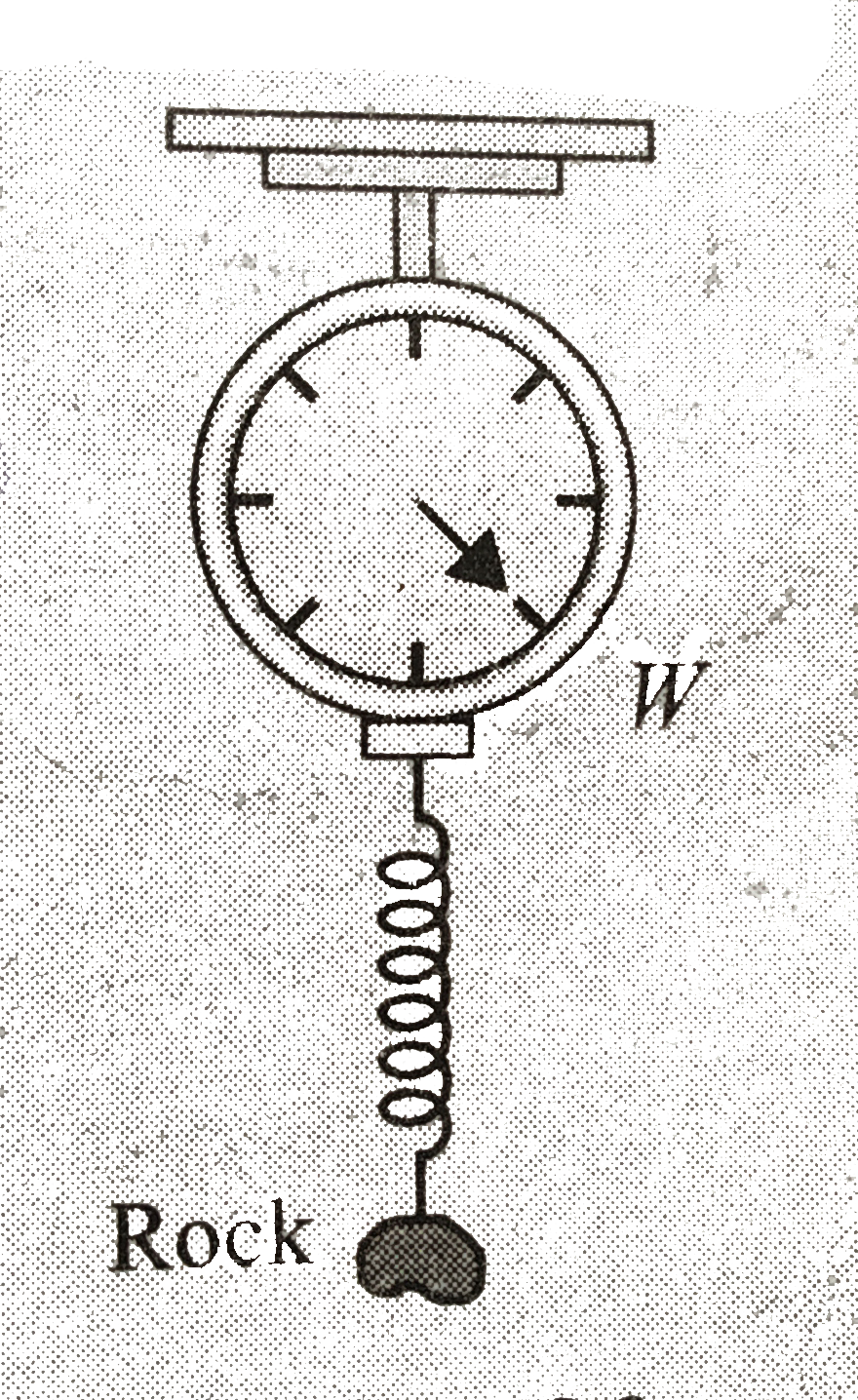
View SolutionThe work done to raise a mass m from the surface of the earth to a height h, which is equal to the radius of the earth, is :
View SolutionTwo identical balls each having a density ρ are suspended from as common point by two insulating strings of equal length. Both the balls have equal mass and charge. In equilibrium each string makes an angle θ with vertical. Now, both the balls are immersed in a liquid. As a result the angle θ does not change. The density of the liquid is σ. Find the dielectric constant of the liquid.
View SolutionTwo identical balls each having a density ρ are suspended from as common point by two insulating strings of equal length. Both the balls have equal mass and charge. In equilibrium each string makes an angle θ with vertical. Now, both the balls are immersed in a liquid. As a result the angle θ does not change. The density of the liquid is σ. Find the dielectric constant of the liquid.
View Solution
