Two identical balls each having a density ρ are suspended from as common point by two insulating strings of equal length. Both the balls have equal mass and charge. In equilibrium each string makes an angle θ with vertical. Now, both the balls are immersed in a liquid. As a result the angle θ does not change. The density of the liquid is σ. Find the dielectric constant of the liquid.
To solve the problem step by step, we need to analyze the forces acting on the balls both in air and in the liquid.
Step 1: Analyze the Forces in Air
When the balls are in air, the forces acting on each ball are:
- The weight of the ball (W_A) acting downwards, given by WA=mg.
- The electrostatic force (F_E) acting horizontally due to the charges on the balls.
- The tension (T) in the string acting at an angle θ with the vertical.
The tension can be resolved into two components:
- Vertical component: Tcosθ
- Horizontal component: Tsinθ
In equilibrium, we have:
1. Tcosθ=mg (1)
2. Tsinθ=FE (2)
Step 2: Divide the Equations
Dividing equation (2) by equation (1):
TsinθTcosθ=FEmg
This simplifies to:
tanθ=FEmg(Equation 3)
Step 3: Analyze the Forces in Liquid
When the balls are immersed in a liquid, the forces acting on each ball change:
- The weight of the ball in the liquid (W_A') is reduced due to the buoyant force. The effective weight is given by:
W′A=mg−Buoyant Force=mg−σVg
where σ is the density of the liquid and V is the volume of the ball.
- The electrostatic force in the liquid (F_E') is reduced by the dielectric constant (k):
F′E=FEk
The tension in the string now has components:
- Vertical component: T′cosθ=W′A (4)
- Horizontal component: T′sinθ=F′E (5)
Step 4: Divide the New Equations
Dividing equation (5) by equation (4):
T′sinθT′cosθ=F′EW′A
This simplifies to:
tanθ=F′EW′A(Equation 6)
Step 5: Equate the Two Equations
Since the angle θ does not change, we can equate the right-hand sides of equations (3) and (6):
FEmg=F′EW′A
Step 6: Substitute the Forces
Substituting F′E=FEk and W′A=mg−σVg:
FEmg=FEkmg−σVg
Step 7: Solve for the Dielectric Constant (k)
Cross-multiplying gives:
FE(mg−σVg)=mg⋅FEk
This leads to:
k=mgmg−σVg
Step 8: Express Volume in Terms of Density
The volume V of the ball can be expressed as:
V=mρ
Substituting this into the equation for k:
k=mgmg−σ(mρ)g
Step 9: Simplify the Expression
This simplifies to:
k=mgmg−σmgρ=ρρ−σ
Final Result
Thus, the dielectric constant of the liquid is:
k=ρρ−σ
When the balls are in air, the forces acting on each ball are:
- The weight of the ball (W_A) acting downwards, given by WA=mg.
- The electrostatic force (F_E) acting horizontally due to the charges on the balls.
- The tension (T) in the string acting at an angle θ with the vertical.
- Vertical component: Tcosθ
- Horizontal component: Tsinθ
1. Tcosθ=mg (1)
2. Tsinθ=FE (2)
Dividing equation (2) by equation (1):
TsinθTcosθ=FEmg
This simplifies to:
tanθ=FEmg(Equation 3)
When the balls are immersed in a liquid, the forces acting on each ball change:
- The weight of the ball in the liquid (W_A') is reduced due to the buoyant force. The effective weight is given by:
W′A=mg−Buoyant Force=mg−σVg
where σ is the density of the liquid and V is the volume of the ball.
F′E=FEk
- Vertical component: T′cosθ=W′A (4)
- Horizontal component: T′sinθ=F′E (5)
Dividing equation (5) by equation (4):
T′sinθT′cosθ=F′EW′A
This simplifies to:
tanθ=F′EW′A(Equation 6)
Since the angle θ does not change, we can equate the right-hand sides of equations (3) and (6):
FEmg=F′EW′A
Substituting F′E=FEk and W′A=mg−σVg:
FEmg=FEkmg−σVg
Cross-multiplying gives:
FE(mg−σVg)=mg⋅FEk
This leads to:
k=mgmg−σVg
The volume V of the ball can be expressed as:
V=mρ
Substituting this into the equation for k:
k=mgmg−σ(mρ)g
This simplifies to:
k=mgmg−σmgρ=ρρ−σ
Thus, the dielectric constant of the liquid is:
k=ρρ−σ
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Example Type 5
5 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Example Type 6
4 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
Two identical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View SolutionTwo indentical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
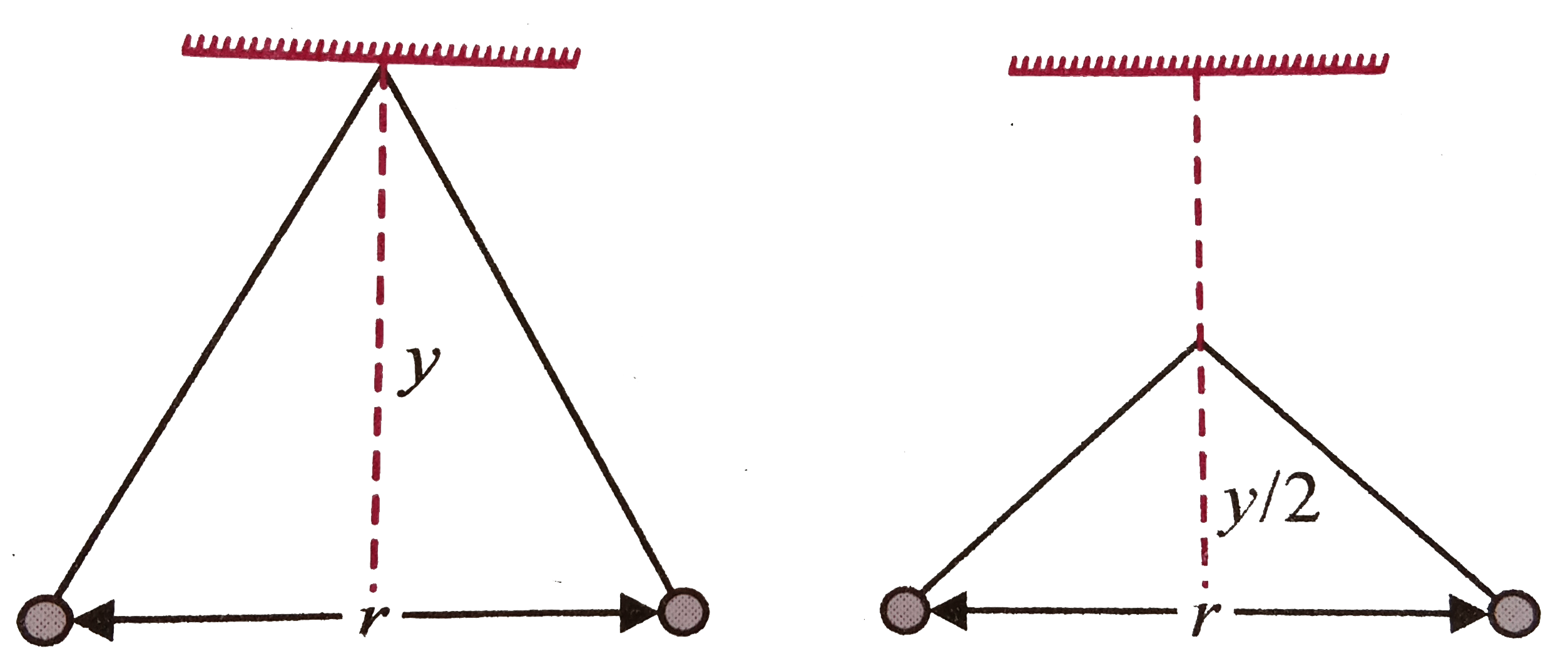
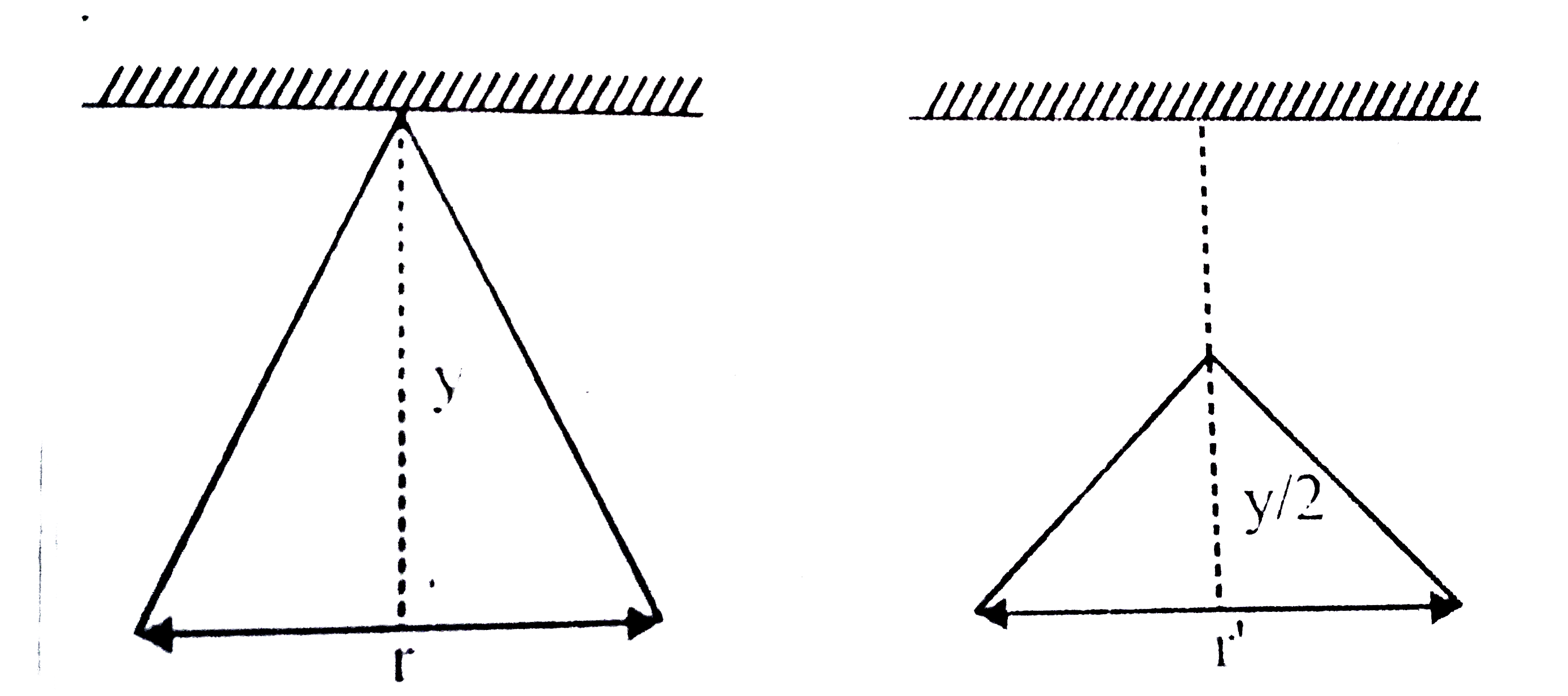
View SolutionTwo path balls carrying equal charges are suspended from a common point by strings of equal length, the strings are rightly clamped at half the height. The equilibrium separation between the balls, now becomes :
View SolutionTwo small spheres, each having a mass of 20 g, are suspended form a common point by two insulating strings of length 40 cm each. The spheres are identically charged and the speration between the balls at equilibrium is found to be 4 cm . Find the charge on each sphere.
View SolutionTwo identical balls , each having a charge q and mass m , are suspended from a common point by two insultating strings each of length L. The balls are held at a separation x and then released. Find
(a) the electric force on each ball
(b) the component of the resultant force on a ball along and perpendicular to string
(c ) the tension in the string
(d) the acceleration of one of the balls. Consider the situation only for the instant just after the release.
View SolutionTwo pitch balls carrying equal charges are suspended from a common point by strings of equal length, the equilibrium separation between them is r. Now the strings are rigidly clamped at half the height. The equilibrium separation between the balls now become
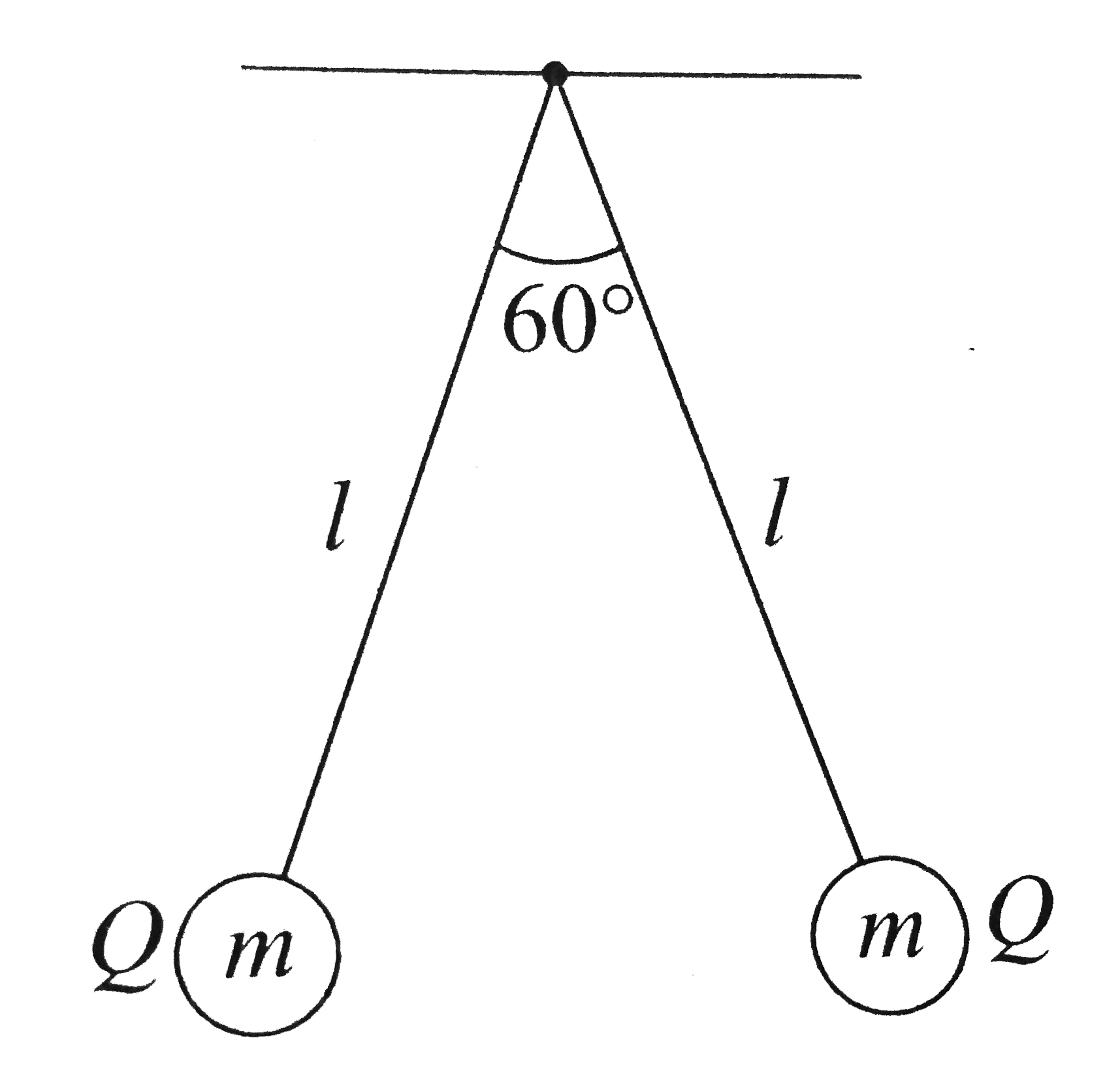
View SolutionTwo small balls, each having equal positive charge Q are suspended by two insulationg strings of equal length l from a hook fixed to a stand. It mass of each ball =m and total angle between the two strings is 60∘m, then find the charge on each ball.
View SolutionTwo identical charged spheres are suspended by strings of equal lengths. The strings make an angle of 30∘ with each other. When suspended in a liquid of density 0.8gcm−3, the angle remains the same. If density of the material of the sphere is 1.6gcm−3, the dielectric constant of the liquid is
View SolutionTwo identical charged spheres are suspended by strings of equal lengths. The strings make an angle of 30∘ with each other. When suspended in a liquid of density 0.8gcm−3, the angle remains the same. If density of the material of the sphere is 1.6gcm−3, the dielectric constant of the liquid is
View SolutionTwo identical charged spheres of material density ρ, suspended from the same point by inextensible strings of equal length make an angle θ between the string. When suspended in a liquid of density σ the angle θ remains the same. The dielectric constant K of the liquid is
View Solution
