Two men each of mass m stand on the rim of a horizontal circular disc, diametrically opposite to each other. The disc has a mass M and is free to rotate about a vertical axis passing through its centre of mass. Each mass start simultaneously along the rim clockwise and reaches their original starting positions on the disc. The angle turned through by disc with respect to the ground (in radian) is
8mπ4m+M
2mπ4m+M
mπM+m
4mπ2M+m
The correct Answer is:A
To solve the problem, we will use the principle of conservation of angular momentum. Let's go through the solution step by step.
Step 1: Understand the System
We have a disc of mass M with two men, each of mass m, standing on its rim, diametrically opposite to each other. When the men start moving clockwise, the disc will rotate in the opposite direction due to conservation of angular momentum.
Step 2: Define the Angular Displacements
Let:
- The angle turned by the disc be θ.
- The total angle covered by each man when they return to their starting position is 2π.
Since the men move clockwise, the disc will rotate counterclockwise. Therefore, the angle covered by the men relative to the ground is 2π−θ.
Step 3: Apply Conservation of Angular Momentum
According to the conservation of angular momentum:
Linitial=Lfinal
The initial angular momentum Linitial is zero because the system is initially at rest.
The final angular momentum can be expressed as:
Lfinal=Lmen+Ldisc
Where:
- Lmen=Imen⋅ωmen
- Ldisc=Idisc⋅ωdisc
Step 4: Calculate the Angular Momentum of the Men
The moment of inertia of each man about the center of the disc is:
Imen=2⋅m⋅R2=2mR2
The angular velocity of the men is:
ωmen=2π−θt
Thus, the angular momentum of the men is:
Lmen=2mR2⋅2π−θt
Step 5: Calculate the Angular Momentum of the Disc
The moment of inertia of the disc about its center is:
Idisc=12MR2
The angular velocity of the disc is:
ωdisc=θt
Thus, the angular momentum of the disc is:
Ldisc=12MR2⋅θt
Step 6: Set Up the Equation
Setting the total angular momentum of the men equal to that of the disc:
2mR2⋅2π−θt=12MR2⋅θt
Cancel R2 and t from both sides:
2m(2π−θ)=12Mθ
Step 7: Solve for θ
Expanding and rearranging:
4mπ−2mθ=12Mθ
4mπ=2mθ+12Mθ
4mπ=θ(2m+12M)
Thus,
θ=4mπ2m+12M
Multiplying through by 2 to simplify:
θ=8mπM+4m
Final Answer
The angle turned through by the disc with respect to the ground is:
θ=8mπM+4m
We have a disc of mass M with two men, each of mass m, standing on its rim, diametrically opposite to each other. When the men start moving clockwise, the disc will rotate in the opposite direction due to conservation of angular momentum.
Let:
- The angle turned by the disc be θ.
- The total angle covered by each man when they return to their starting position is 2π.
According to the conservation of angular momentum:
Linitial=Lfinal
The initial angular momentum Linitial is zero because the system is initially at rest.
Lfinal=Lmen+Ldisc
Where:
- Lmen=Imen⋅ωmen
- Ldisc=Idisc⋅ωdisc
The moment of inertia of each man about the center of the disc is:
Imen=2⋅m⋅R2=2mR2
The angular velocity of the men is:
ωmen=2π−θt
Thus, the angular momentum of the men is:
Lmen=2mR2⋅2π−θt
The moment of inertia of the disc about its center is:
Idisc=12MR2
The angular velocity of the disc is:
ωdisc=θt
Thus, the angular momentum of the disc is:
Ldisc=12MR2⋅θt
Setting the total angular momentum of the men equal to that of the disc:
2mR2⋅2π−θt=12MR2⋅θt
Cancel R2 and t from both sides:
2m(2π−θ)=12Mθ
Expanding and rearranging:
4mπ−2mθ=12Mθ
4mπ=2mθ+12Mθ
4mπ=θ(2m+12M)
Thus,
θ=4mπ2m+12M
Multiplying through by 2 to simplify:
θ=8mπM+4m
The angle turned through by the disc with respect to the ground is:
θ=8mπM+4m
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - A Only One Option is Correct
86 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - More than one option is correct
36 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
Similar Questions
A disc is free to rotate about a smooth horizontal axis passing through its centre of mass. A particle is fixed at the top of the disc. A slight push is given to the disc and it starts rotating. During the process.
View SolutionA horizontal disc is rotating about a vertical axis passing through its centre. If an insect moves from centre to rim then the angular momentum of the system
View SolutionA circular disc has a mass of 1kg and radius 40 cm. It is rotating about an axis passing through its centre and perpendicular to its plane with a speed of 10rev/s. The work done in joules in stopping it would be-
View SolutionIf a circular concentric hole is made on a disc then about an axis passing through the centre of the disc and perpendicular to its plane
View SolutionA metallic circular disc having a circular hole at its centre rotates about an axis passing through its centre and perpendicular to its plane. When the disc is heated:
View SolutionA metallic circular disc having a circular hole at its centre rotates about an axis passing through its centre and perpendicular to its plane. When the disc is heated:
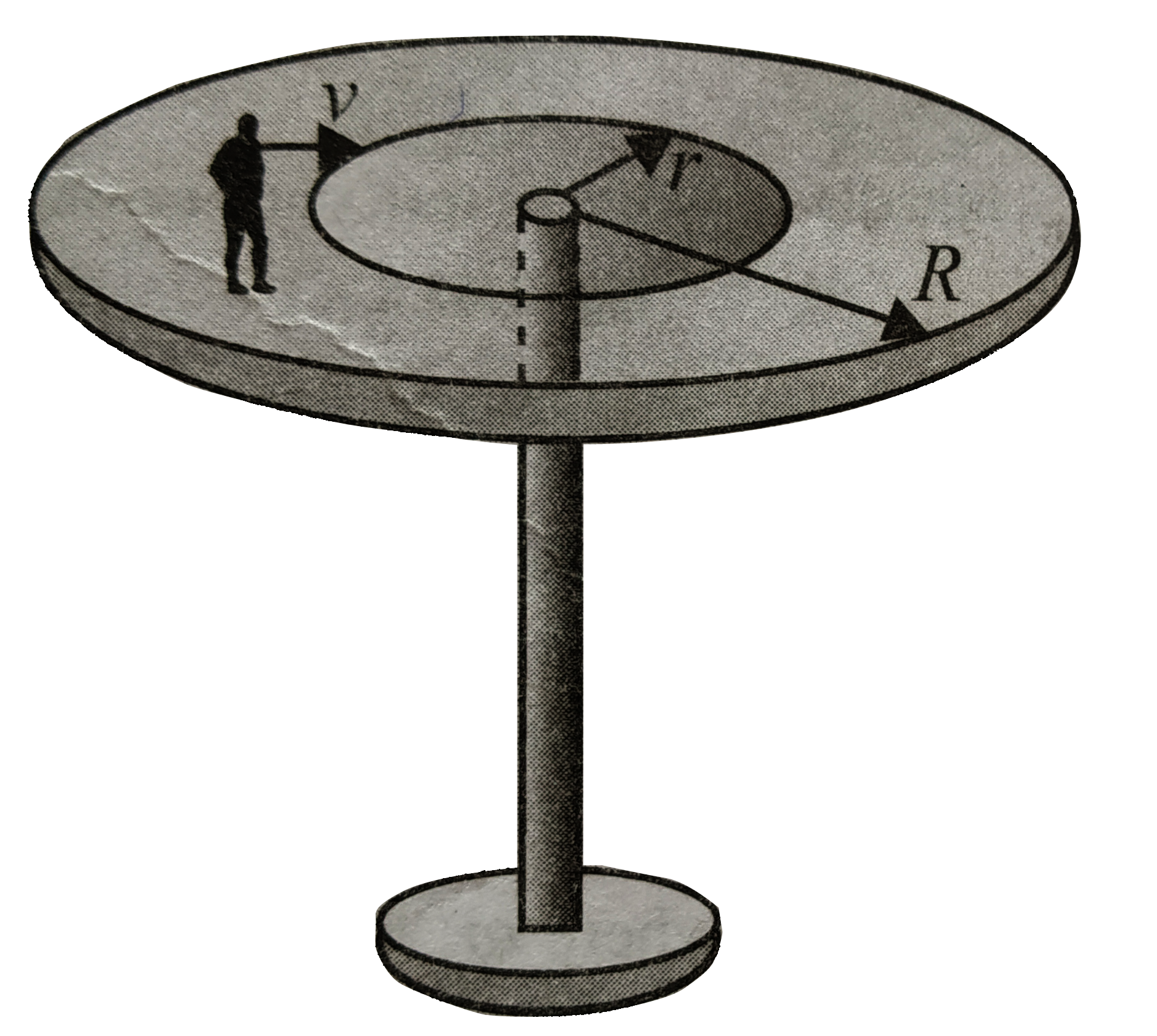
View SolutionA man of mass m stands on a horizontal platform in the shape of a disc of mass m and radius R, pivoted on a vertical axis thorugh its centre about which it can freely rotate. The man starts to move aroung the centre of the disc in a circle of radius r with a velocity v relative to the disc. Calculate the angular velocity of the disc.
View SolutionThe ratio of radii of gyration of a circular ring and a circular disc, of the same mass and radius about an axis passing through their centres and perpendicular to their planes are
View SolutionA uniform disc of mass m and radius R rotates about a fixed vertical axis passing through its centre with angular velocity ω. A particle of same mass m and having velocity of 2ωR towards centre of the disc collides with the disc moving horizontally and sticks to its rim. Then
View SolutionFive particles of mass 2 kg are attached to the rim of a circular disc of radius 0.1 m & negligible mass. Moment of inertia of the system about an axis passing through the centre of the disc & perpendicular to its plane is
View Solution