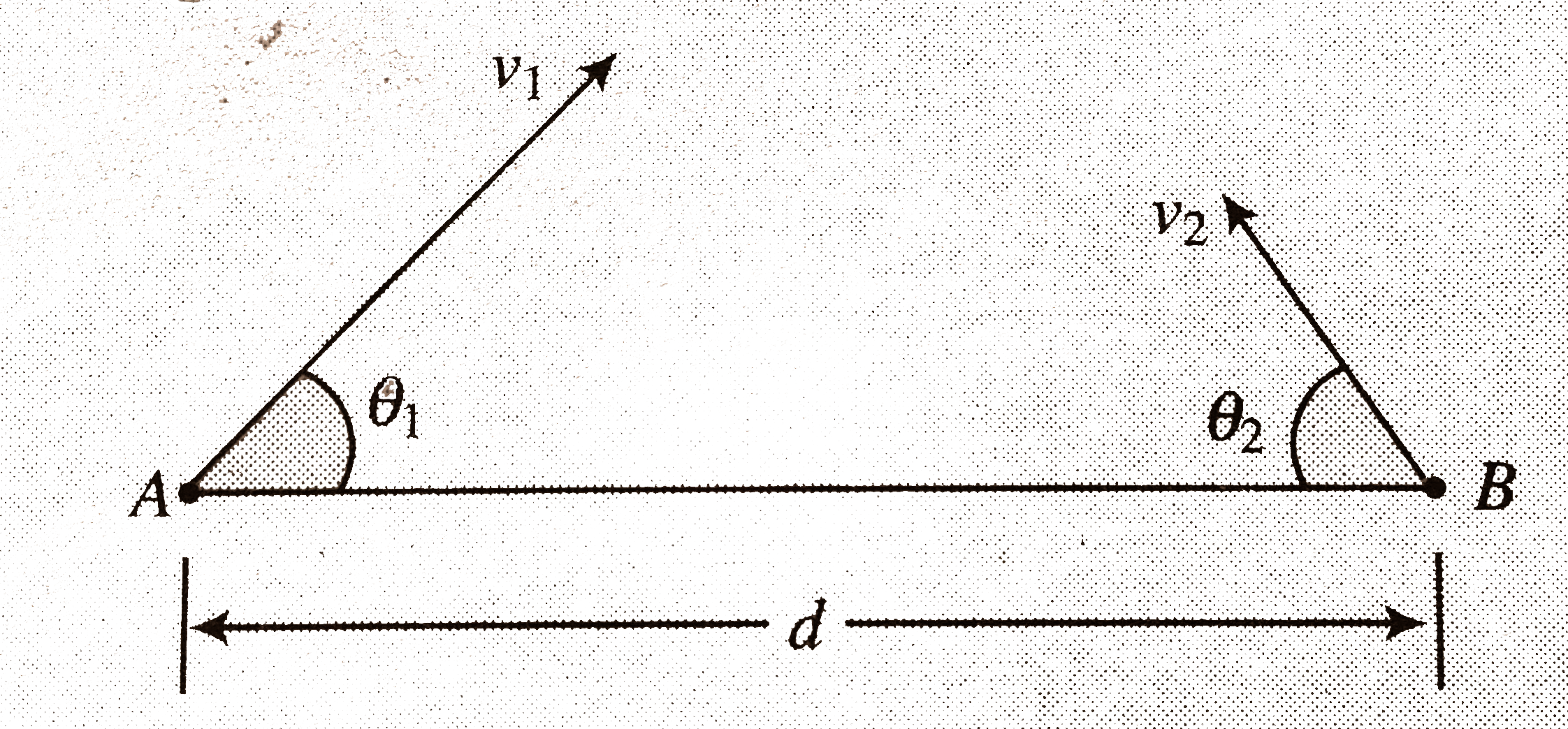
Two particles AandB are moving with constant velocities v1andv2. At t=0, v1 makes an angle θ0 with the line joining AandBandv2 makes an angle θ2 with the line joining AandB.
(a) Find the condition for AandB to collide.
(b) Find the time after which AandB will collide if separation between them is datt=0.
 .
.
(a) For AandB to collide, their relative velocity must be directed along the line joining them.
Therefore their relative velocity along the perpendicular to this line must be zero.
Thus v1sinθ1=v2sinθ2.
(b) vAPP=v1cosθ1+v2cosθ2
t=dvaPP=dv1cosθ1+v2cosθ2.
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Solved Examples
7 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.1
15 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
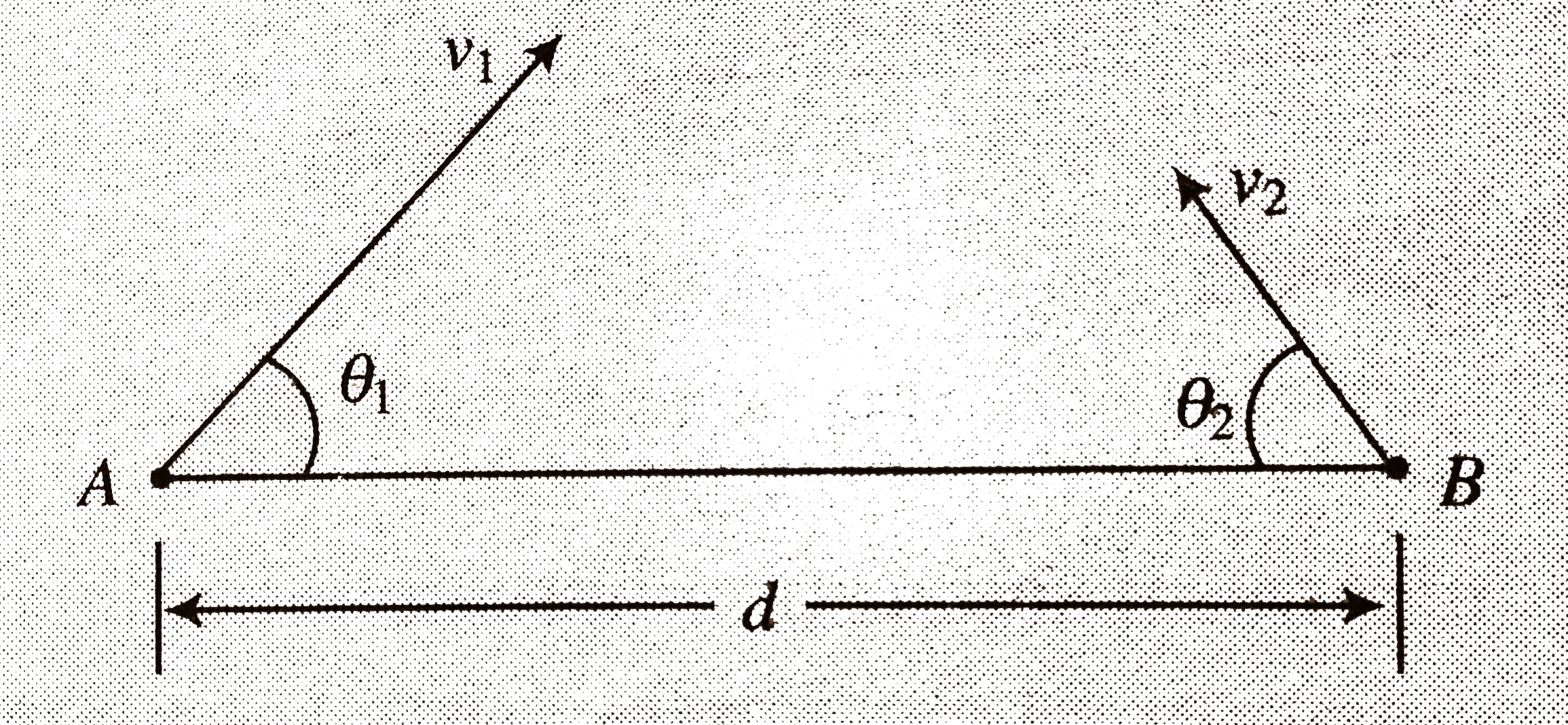
Two particles AandB are moving with constant velocities v1andv2. At t=0, v1 makes an angle θ0 with the line joining AandB and v2 makes an angle θ2 with the line joining AandB. Find their velocity of approach.
 .
.
View SolutionTwo particles 1and2 move with velocities →v1 and →v2 making the angles θ1andθ2 with the line joining them, respectively. Find angular velocity of relative→1.
 .
.
View Solution
Knowledge Check
Two particles are moving with velocities v1andv2 . Their relative velocity is the maximum, when the angle between their velocities is
AzeroBπ/4Cπ/2DπTwo projectiles A and B are thrown with the same speed such that A makes angle θ with the horizontal and B makes angle θ with the vertical, then -
ABoth must have same time of flightBBoth must achieve same maximum heightCA must have more horizontal range than BDBoth may have same time of flightTwo particle A and B, move with constant velocities →v1and→v2. At the initial moment their position vectors are →r1 and →r2 respectively . The condition for particles A and B for their collision is
A→r1×→v1=→r2×→v2B→r1−→r2=→v1−→v2C→r1−→r2∣∣→r1−→r2∣∣=→v2−→v1∣∣→v2−→v1∣∣D→r1.→v1=→r2.→v2
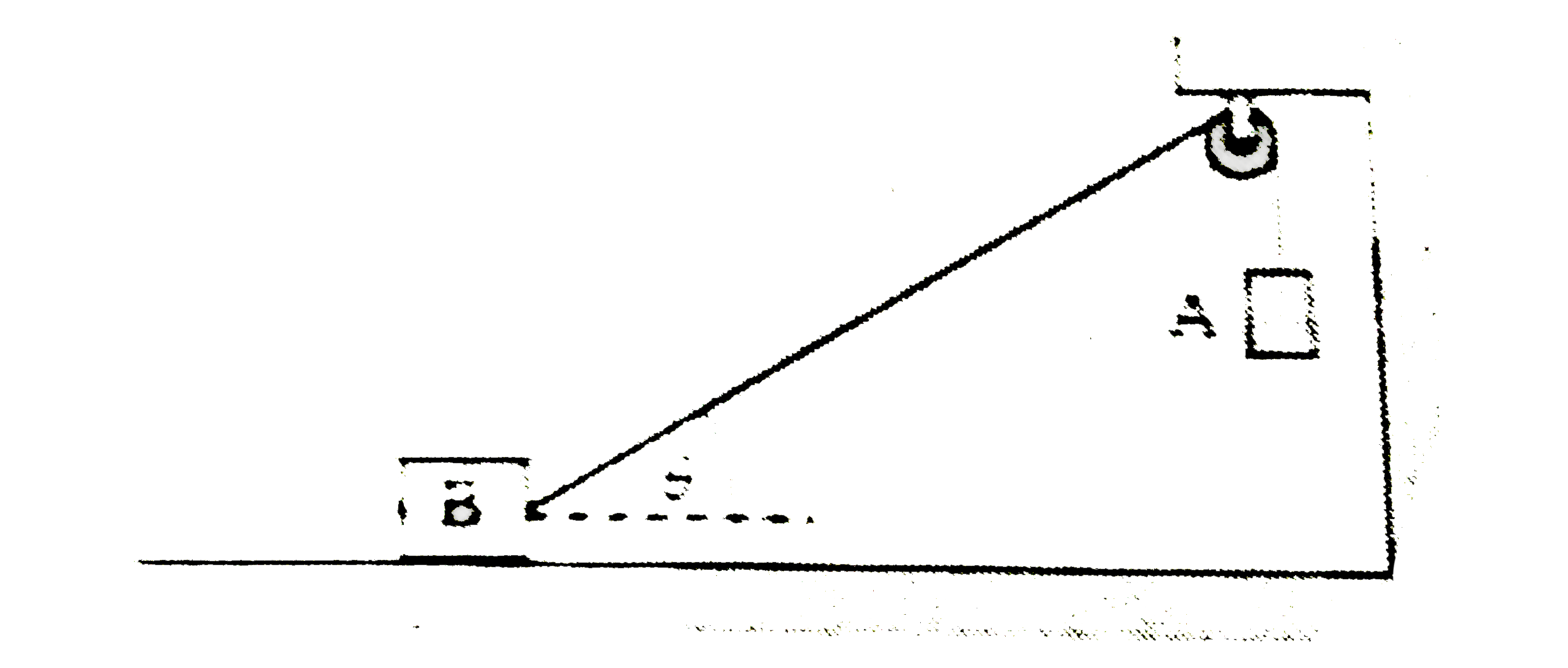
The block A is moving downward with constant velocity v0. Find the velocity of the block B. When the string makes an angle θ with the horizontal
View SolutionTwo bodies A and B are moving with velocities vA and vB, making an angle θ with each other. Determine the relative velocity of A w.r.t. B. What will be the relative velocity when the two bodies move in same direction
View SolutionTwo bodies A and B are moving with velocities vA and vB, making an angle θ with each other. Determine the relative velocity of A w.r.t. B. What will be the relative velocity when the two bodies move in opposite directions ?
View SolutionTwo particles, 1 and 2, move with constant velocities v1 and v2. At the initial moment their radius vectors are equal to r1 and r2. How must these four vectors be interrelated for the particles to collide?
View SolutionTwo particles A and B, move with constant velocities →v1 and →v2. At the initial moment their position vectors are →r1 and →r2 respectively. The condition for particle A and B for their collision is
View Solution
