Question

Two rigid bodies A and B of masses 1 kg and 2 kg respectively are rigidly connected to a spring of force constant 400 $N{m}^{-1}$ . The body B rests on a horizontal table. From the rest position , the body A is compressed by 2 cm and then released . Deduce (i) teh frequency of oscillation, (ii) total oscillation energy, (iii) the amplitude of the harmonic vibration of the reaction of the table on body B.

Explanation

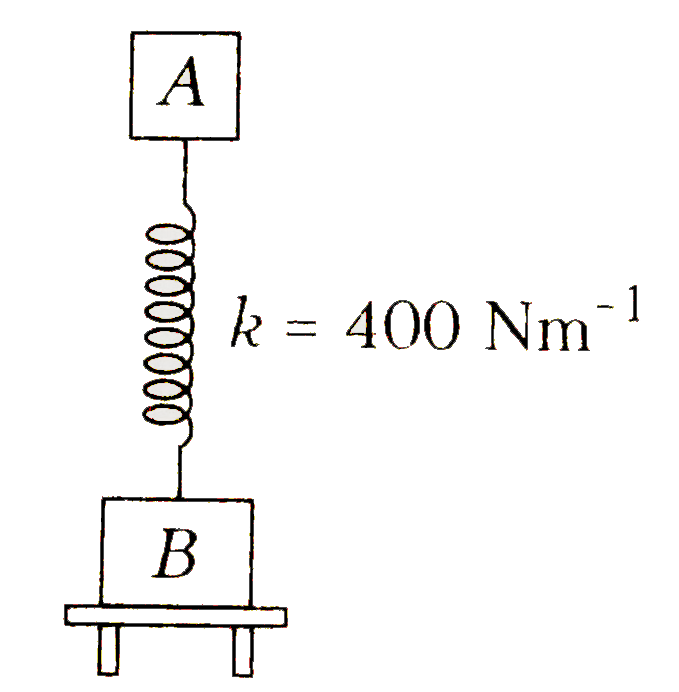
Since it is body A which is oscillation , m=1 kg
(i) Frequency of osillation , `v=(1)/(2pi)sqrt((k)/(m))`
or `v=(1)/(2pi)sqrt((400)/(1)) Hz=(10)/(pi)Hz=3.182 Hz`
(ii) Total oscillation energy, `E=(1)/(2)mA^(2)omega^(2)`
or `E=(1)/(2)mA^(2)((k)/(m))=(1)/(2)kA^(2)`
`=(1)/(2)xx400xx(0.02)^(2) J=0.08 J (because a=2 cm=0.02 m)`
Total force acting on the table
`=(1+2 ) kg wt=3 kg wt=3xx9.8 N=29.4 N`
This is the mean upward reaction. Now, due to oscillation, the maximum tension developed in the spring is given by `F=kA=400xx 0.02 N=8 N`
Thus , the amplitude of the vibration of reaction of the table will vary from (29.4+8) N to (29.4)N.