Two sinusoidal waves with the same amplitude and wavelength travel along a string that is stretched along an x axis, the resultant wave due to their superposition was recorded on video tape. The curves in Fig. 16-31 represent the resultant wave in four freeze frames in the sequence of a, b, c, and d, with 1.0 ms elapsing between curves a and d. The grid lines along the x axis are 1.0 cm apart, and the string elements oscillated perpendicular to the r axis by 16.0 mm (between the extreme displacements shown by curves a and d) as the resultant wave passed through them. Write equations for the two superposition waves and for their resultant wave. 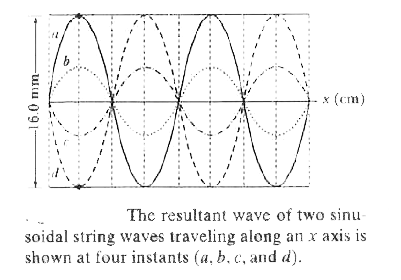
Figure 16-31, shows a standing wave and so the two waves must be travelling in opposite directions.
The two waves have the forms given by
y1(x,t)=ymsin(kx−ωt)
and y2(x,t)=ymsin(kx+ωt)
Hence, we can write the resultant wave as
y.(x,t)=(2ym,sinkx)cosωt
We need to find the common values of ym,k, and a in the three equations.
Calculations: To find ym we use the key idea that the amplitude of a standing wave is a maximum at an antinode, and there the amplitude is 2ym. Here the maximum amplitude of the standing wave is 8.0 mm, so
ym=4.0mm
To find the angular wave number k, we use two more key ideas. First, the common angular wave number k in Eqs. 16-71, 16-72 and 16-73 is related to the common wavelength λ by equation k=2π/λ. Second, the nodes in a standing wave arc separated by 0.502. Because the antinodes in Fig. 16-31 are separated by 2.0 cm, we have λ=2×2.0cm=4.0cm .Then
k=2πλ=2π0.040m=157m−1≈160m−1
To find the common angular frequency ω we again use two more key ideas: We can relate the common value of ω in Eqs. 16-71, 16-72, and 16-73 to the period T of the standing wave with equation o = 24/7. A string element at an antinode takes time T to move through a full oscillation from one extreme and back to the first extreme. According to the given data, the string element at the dot on curve a in Fig. 16-31 takes 10 ms to move to the position of the dot on curve d, which is one half a full oscilations. Thus, T=2.0 ms, and
ω=2πT=2π0.0020s=3142s−1≈3100s−1
Now we can write Eqs. 16-71 and 16-72 for the interfering waves as
y1(x,t)=(4.0mm)sin(160x−3100t)
andy2(x,t)=(4.0mm)sin(160x−3100t)
with x in meters and t in seconds. We can also write the standing wave of Eq. 16-73 as
y.(x,t)=(8.0mm)(sin160x)(cos3100t) again with x in meters and t in seconds.
Topper's Solved these Questions
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Checkpoint
8 Videos
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Problems
60 Videos
- WAVE - IIBOOK - RESNICK AND HALLIDAYCHAPTER - WAVE - IIEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)
5 Videos
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (Integer Type)
5 Videos
Similar Questions
Two sinusoidal waves with the same amplitude of 4.0mm and the same wavelength traveled together along a string that is stretched along an x axis, the resultant wave due to their interference was recorded on video tape. The curves in Fig. 16-20 represent the resultant wave in two freeze frames, first as the solid curve and then, 1.0 ms later, as the dotted curve. The grid lines along the x axis are 1.0 cm apart, and the string clements oscillated vertically (perpendicular to the x axis) by 6.0 mm as the resultant wave passed through them. That wave moved a distance d =4.20 cm to the right in the 1.0 ms time interval. Write equations for the two interfering waves and for their resultant wave.
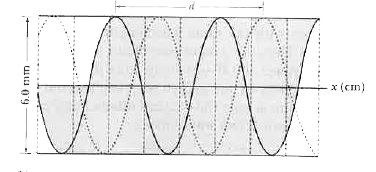
Figure 16-20 The resultant wave of two sinusoidal string wave traveling along an x axis is shown at two instants, between which the resultant wave travels distance d.
View SolutionTwo sinusoidal waves with the same amplitude and wavelength travel through each other along a string that is stretched along an x axis. Their resultant wave is shown twice in Fig. 16-53, as the antinode A travels from an extreme upward displacement to an extreme downward displacement in 6.0 ms. The tick marks along the axis are separated by 15 cm, height H is 1.20 cm. Let the equation for one of the two waves be of the form y(x,t)=ymsin(kx+ω). In the equation for the other wave, what are (a)ym(b)k,(c)ω, and (d) the sign in front of ω?

View Solution
Knowledge Check
What is phase difference between two waves, if the resultant amplitude due to their superposition is same as that of the waves?
Aπ/2BπC2π/3Dnone of theseLight waves travel in vacuum along the y-axis. Which of the following may represent the wavefront?
Ay = constantBx = constantCt = constantDx+y+z= constantIf a wave is propagated along the stretched string in the form
ALongitudinal waveBElectromagnetic waveCTransverse waveDAll of the above
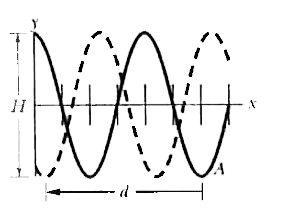
Two sinusoidal waves with the same amplitude of 6.00 mm and the same wavelength travel together along a string that is stretched along an x axis. Their resultant wave is shown twice in Fig. 16 39. as velley travels in the negative direction of the x axis by distance d= 56.0 cm in 8.0 ms. The lick matks along the axis are separated by 10cm, and height H is 8.0mm. Let the equation for one wive be of the form y(x,t) =ymsin(kx±ωt+ϕ1), where ϕ1=0 and you must choose the correct sign in front of ω. For the equation for the other wave, what are (a) ym,(b)k,(c)ω,(d)ϕ2, and (e) the sign in front of ω?
View SolutionA harmonic wave is travelling along +ve x-axis, on a stretched string. If wavelength of the wave gets doubled, then
View SolutionTwo sinusoidal waves of the same period, with amplitudes of 5.0 and 7.0 mm, travel in the same direction along a stretched string, they produce a resultant wave with an amplitude of 10.0 mm. The phase constant of the 5.0mm wave is 0. What is the phase constant of the 7.0mm wave?
View SolutionA harmonic wave is travelling along +ve x-axis, on a stretched string. If wavelength of the wave gets doubled, then
View SolutionLight wave is travelling along X-axis in a medium. Which of the following can represent wavefront for this light wave ?
View Solution
