Two spherical bodies of mass m1 and m2 fall freely through a distance h. before the body m2 collides with the ground. If the coefficient of restitution of all collisions is e, find the velocity of m1 just after it collides with m2
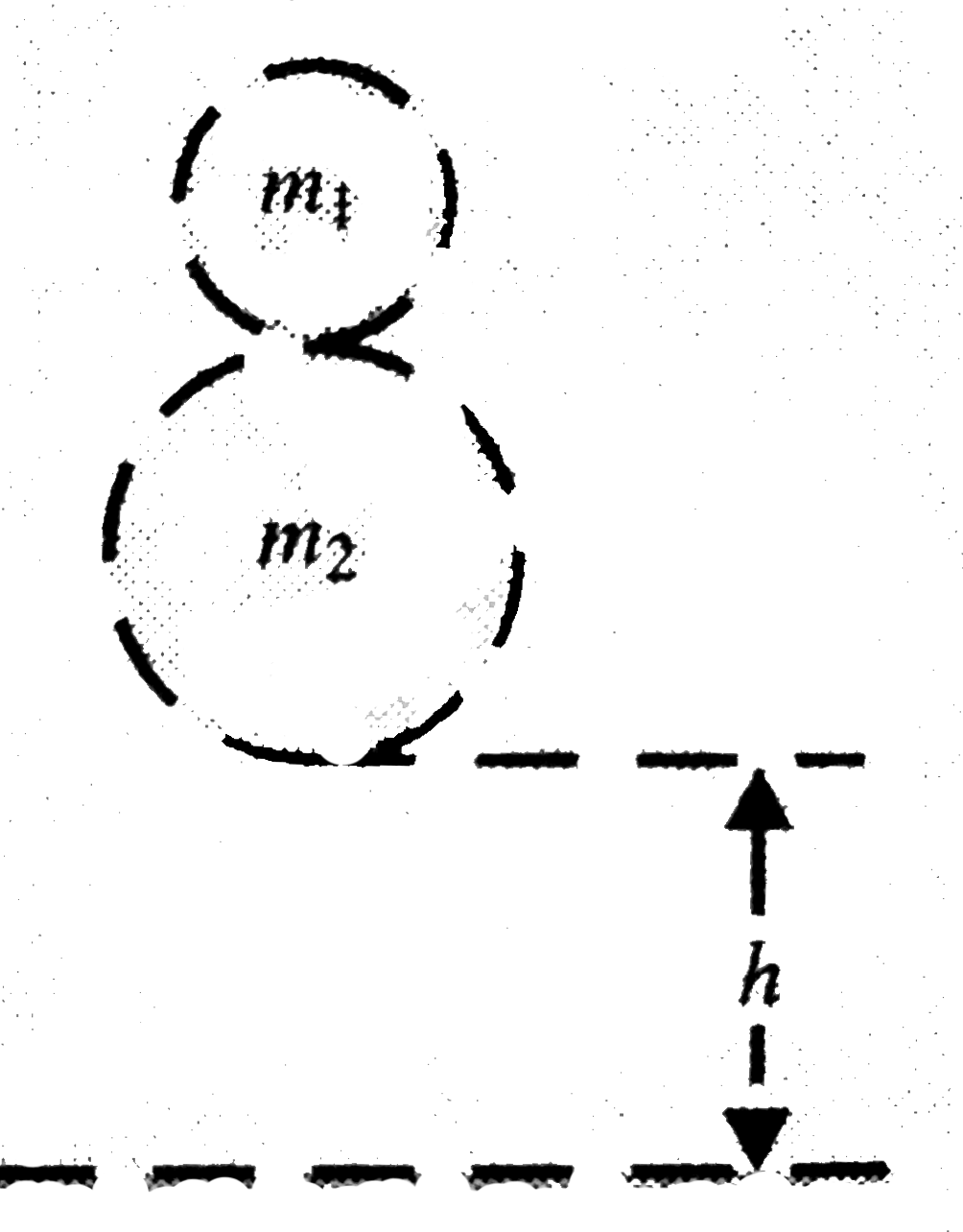
As the bodies m1 and m2 fall freely through a distance h, m2 collides with ground with velocity v0=√2gh. Hence m2 bounces up with a velocity ev0 just after the collision with the ground.
Hence m2 bounces up with a velocity ev0 just after the collision with the ground. then it collides with the freely falling body m1. Just after the collision, let us assume that v1 and v2 are the velocities of m1 and m2 respectively.
ignoring the impulses due to the weights of the bodies during the period of collision. Let us conserve the momentum of the system (m1+m2).
Conservation of linear momentum
P=−m1v0+m2ev0=m1v1+m2v2.......i
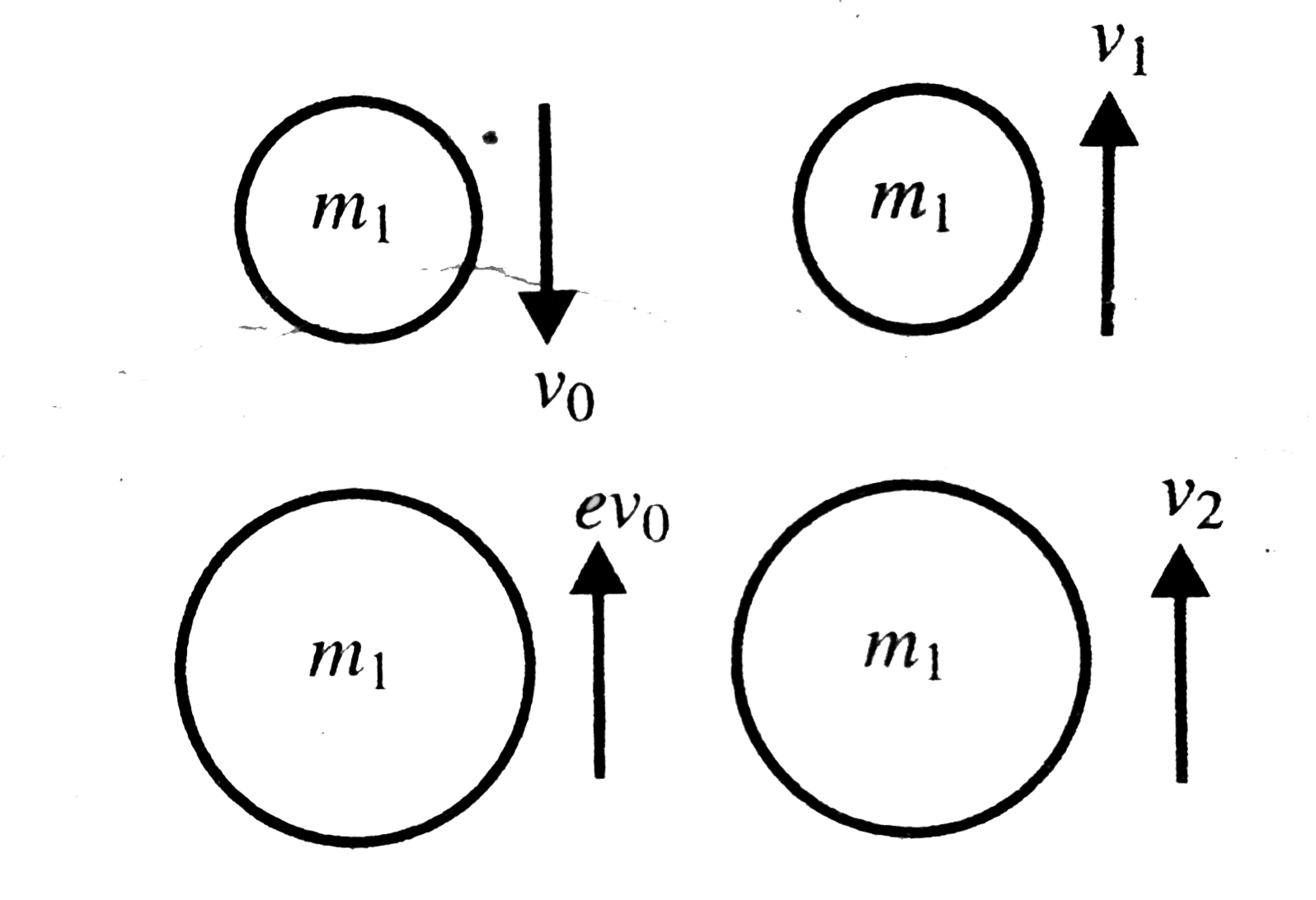
Newton's impact formula −e[u1−u2]=v1−v2
where u1=−v0 and u2=ev0
Then −e[(−v0)−(ev0)]=v1−v2.............ii
Solving eqn i and ii we have
v1=−(m1−em2)m1+m2v0+(1+e)m2m1+m2ev0
Putting m1=m2,e=12 and v0=√2gh we have v1=√gh32↑⏐
⏐
⏐
⏐⏐
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.1
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.2
23 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
Two bodies of masses m1andm2(<m1) are connected to the ends of a massless cord and allowed to move as shown in figure. The pulley is both massless and frictionless. The acceleration of the centre of mass is (m1−m2)n(m1+m2)2g. Find the value of n.

View SolutionA glass ball collides with an identical ball at rest with v0=2 m/sec. If the coefficient of restitution of collision is e = 0.5, find the velocities of the glass balls just after the collision.
View Solution
Knowledge Check
A ball of mass m moving with a speed 2v0 collides head-on with an identical ball at rest. If e is the coefficient of restitution, then what will be the ratio of velocity of two balls after collision?
A1−e1+eBe−1e+1C1+e1−eDe+1e−1A ball of mass m moving with a speed 2v0 collides head-on with an identical ball at rest. If e is the coefficient of restitution, then what will be the ratio of velocity of two balls after collision?
A1−e1+eB1+e1−eCe−1e+1De+1e−1
A block of mass m moving at a velocity υ collides head on with another block of mass 2m at rest. If the coefficient of restitution is 1/2, find the velocities of the blocks after the collision.
View SolutionTwo bodies of masses m1andm2 fall from heights h1andh2 respectively. The ratio of their velocities when the hit the ground is
View SolutionA ball of mass m moving with velocity u collides head-on which the second ball of mass m at rest. If the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then
View SolutionA ball of mass m moving with velocity v collides head-on which the second ball of mass m at rest. I the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then
View SolutionTwo blocks A and B of mass m and 2m respectively are moving towards a massive (mass>>2m) cliff with velocities 2v and v respectively. The cliff moves with a velocity v. If the coefficient of restitution of collision at the surface of the cliff is e=1/2, find the:

a. velocity of the block B just after colliding with the cliff.
b. work done by the cliff during collision.
c. maximum compression of the spring of stiffness k which is fitted with the block B.
View SolutionA body of mass m falls from height h, on the ground, If e is the coefficient of restitution between body and the ground, then the height upto which it rises after ten collisions is
View Solution
