Two bodies of masses m1andm2(<m1) are connected to the ends of a massless cord and allowed to move as shown in figure. The pulley is both massless and frictionless. The acceleration of the centre of mass is (m1−m2)n(m1+m2)2g. Find the value of n.

The correct Answer is:2
If →a is the acceleration ofm1 then −→a is the acceleration of m2.
We know that (m1+m2)→Acm.m1→a1+m2→a2
or (m1+m2)→Acm=m1→a−m2→a or →acm.=m1−m2m1+m2→a
But, →a=m−1−m2m1+m2→g [connected - body problem] ∴ →Acm.=(m1−m2)2(m1+m2)2→g.
Topper's Solved these Questions
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - Level - 1 PARAGRAPH QUESTIONS
3 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - LEVEL - 2
48 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - LEVEL - 0 - LONG ANSWER TYPE
5 Videos
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)
89 Videos
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )
111 Videos
Similar Questions
Two bodies with masses m1 and m2(m1>m2) are joined by a string passing over fixed pulley. Assume masses of the pulley and thread negligible. Then the acceleration of the centre of mass of the system (m1+m2) is
View SolutionTwo bodies of mass m1andm2 are initially at rest placed infinite distance apart. They are then allowed to move towards each other under mutual gravitational attraction. Show that their relative velocity of approach at separation r between them is:
View Solution
Knowledge Check
Two bodies of masses m and 4 m are moving with equal K.E. The ratio of their linear momentum is
A4:1B1:1C1:2D1:4
Two bodies of masses m1, and m2, moving with velocity u1, and u2, respectively in the same direction collide with each other elastically. Calculate their velocities after the collision. Discuss what happens when
(i) Both the colliding bodies have the same mass.
(ii) One of the bodies is initially at rest.
(iii) A light body collides with a heavy body at rest
(iv) A heavy body collides with a light body at rest
View Solution
Two blocks of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is :
View SolutionTwo bodies of masses m and 2m are moving in same direction with speed 2v and v respectively just after collision body of mass m come to rest and body of mass 2m splits in two equal parts and move at 45∘ from initial direction of body of mass m. Find out speed of one part after collision
View SolutionTwo particles of masses m1andm2 are connected to a string and the system is rotated in a horizontal plane with 'P' as center. The ratio of tension in the two parts of string is

View SolutionTwo bodies of masses m and 4 m are attached with string as shown in the figure. The body of mass m hanging from a string of length l is executing oscillations of angular amplitude θ0 while the other body is at rest. The minimum coefficient of friction between the mass 4m and the horizontal surface should be
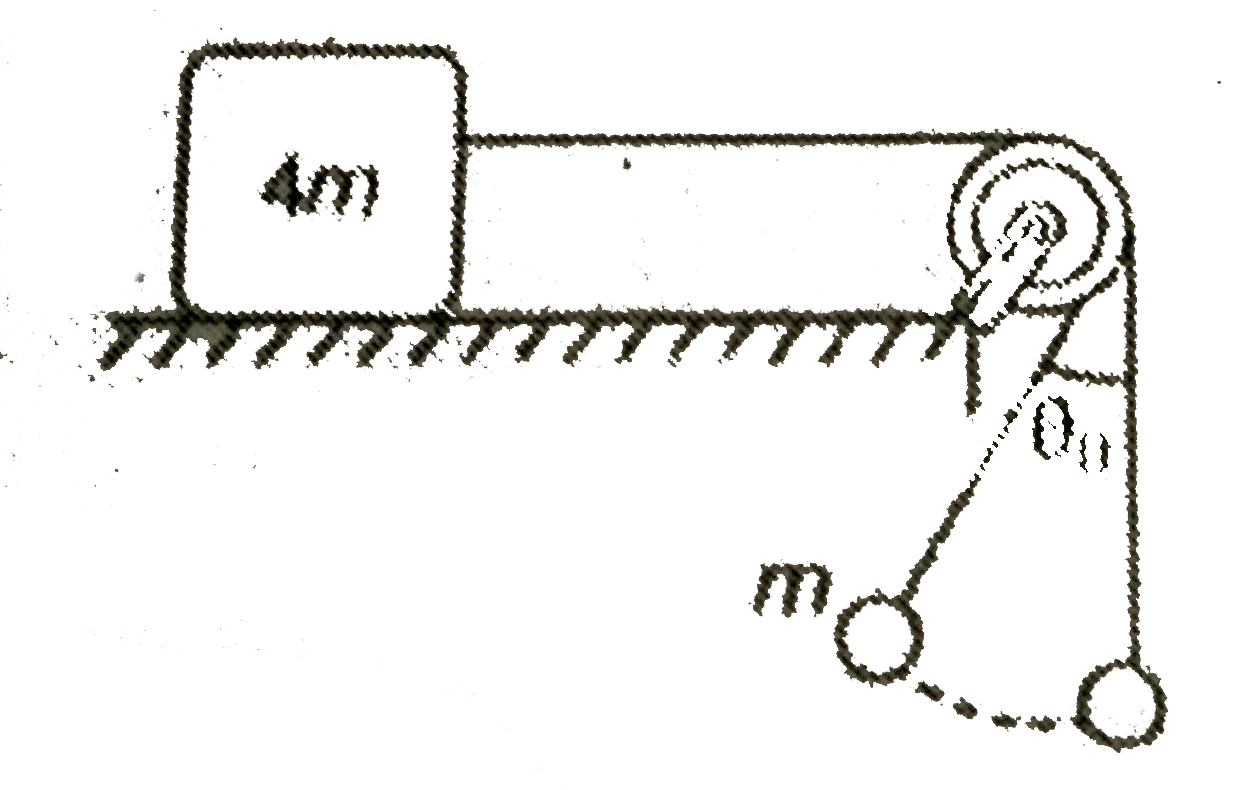
View SolutionTwo bodies of masses m and 2m are moving along positive x and y axes respectively with equal speed 4ms−1. They collide at origin and stick together. The final velocity of combined mass is
View SolutionTwo bodies having masses m1 and m2 and velocities v1 and v2 colide and form a composite system. If m1v1+m2v2=0(m1≠m2. The velocity of composite system will be
View Solution
