Two uniformly charged large plane sheets S1 and S2 having charge densities σ1 and σ2 (σ1>σ2) are placed at a distance d parallel to each other. A charge q0 is moved along a line of length a(altd) at an angle 45∘ with the normal to S1. Calculate the work done by the electric field
The correct Answer is:W=q0Ea√2
To solve the problem, we need to calculate the work done by the electric field when a charge q0 is moved along a line at an angle of 45∘ with the normal to the charged plane sheet S1.
1. Identify the Electric Fields:
The electric field due to a uniformly charged infinite plane sheet is given by:
E=σ2ϵ0
where σ is the surface charge density and ϵ0 is the permittivity of free space.
For the two sheets S1 and S2:
- The electric field E1 due to sheet S1 is:
E1=σ12ϵ0
- The electric field E2 due to sheet S2 is:
E2=σ22ϵ0
2. Determine the Net Electric Field:
Since the charge densities are given as σ1>σ2, the net electric field E between the two sheets is:
E=E1−E2=σ12ϵ0−σ22ϵ0=σ1−σ22ϵ0
3. Calculate the Force on the Charge:
The force F acting on the charge q0 in the electric field is given by:
F=q0E=q0(σ1−σ22ϵ0)
4. Determine the Displacement:
The charge q0 is moved along a line of length a at an angle of 45∘ with the normal to S1. The displacement s can be expressed as:
s=d
5. Calculate the Work Done:
The work done W by the electric field when moving the charge is given by:
W=F⋅s⋅cos(θ)
where θ=45∘. Thus, substituting the values:
W=(q0σ1−σ22ϵ0)dcos(45∘)
Since cos(45∘)=1√2:
W=q0σ1−σ22ϵ0d⋅1√2
Therefore, the final expression for the work done is:
W=q0d(σ1−σ2)2ϵ0√2
Final Answer:
W=q0d(σ1−σ2)2ϵ0√2
The electric field due to a uniformly charged infinite plane sheet is given by:
E=σ2ϵ0
where σ is the surface charge density and ϵ0 is the permittivity of free space.
- The electric field E1 due to sheet S1 is:
E1=σ12ϵ0
- The electric field E2 due to sheet S2 is:
E2=σ22ϵ0
Since the charge densities are given as σ1>σ2, the net electric field E between the two sheets is:
E=E1−E2=σ12ϵ0−σ22ϵ0=σ1−σ22ϵ0
The force F acting on the charge q0 in the electric field is given by:
F=q0E=q0(σ1−σ22ϵ0)
The charge q0 is moved along a line of length a at an angle of 45∘ with the normal to S1. The displacement s can be expressed as:
s=d
The work done W by the electric field when moving the charge is given by:
W=F⋅s⋅cos(θ)
where θ=45∘. Thus, substituting the values:
W=(q0σ1−σ22ϵ0)dcos(45∘)
Since cos(45∘)=1√2:
W=q0σ1−σ22ϵ0d⋅1√2
Therefore, the final expression for the work done is:
W=q0d(σ1−σ2)2ϵ0√2
W=q0d(σ1−σ2)2ϵ0√2
Topper's Solved these Questions
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Single Correct
56 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Multiple Correct
10 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Exercise 3.3
9 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s
38 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q
2 Videos
Similar Questions
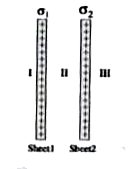
Two paralle plane sheets 1 and 2 carry uniform charge densites σ1andσ2 (see fig. The electric field in the region marked I is (σ1>σ2):
View SolutionTwo infinitely large sheets having charge densities σ1andσ2 respectively (σ1>σ2) are placed near each other separated by distance 'd'. A small charge 'q' is placed in between two plates such that there is no effect on charge distribution on plates. Now this charge is moved at an angle of 45∘ with the horizontal towards plate having charge density σ2 by distance 'a' (a<<d). Find the work done by electric field in the process.
View SolutionTwo non-conduction hollow uniformly charged spheres of radil R1 and R2 with charge Q1 and Q2 respectively are places at a distance r. Find out total energy of the system.

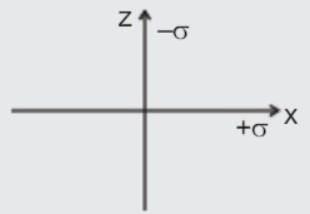
View SolutionTwo infinitely large charged planes having uniform surface change density +σ and -σ are placed along xy plane and yz plane resp as shown in figure. Then nature of electric lines of force in xz plane is given by
View SolutionTwo charged thin infinite plane sheets of uniform surface charge density σ+andσ− where |σ+|>|σ−| , intersect right angle Which of the following best represents the electric field lines for this system:
View SolutionTwo infinitely large charged planes having uniform suface charge density +σ and −σ are placed along x−y plane and yz plane respectively as shown in the figure. Then the nature of electric lines of forces in x−z plane is given by:

View SolutionTwo point electric charges of value q and 2q are kept at a distance d apart from each other in air. A third charge Q is to be kept along the same line in such a way that the net force action on q and 2q is zero. Calculate the position of charge Q in terms of q and d.
View SolutionAnswer the following:
(i) If two similar large plates, each of area A having surface charge densities +σ and −σ are separated by a distance d in air,find the expression for
(a) field at points between the two plates and on outer side of the plates. Specify the direction of the field in each case.
(b) the potential difference between the plates.
(c) the capacitance of the capacitor so formed.
(ii) Two metallic spheres of radii R and 2R are charged so that both of these have same surface charge density σ. If they are connected to each other with a conducting wire, in which direction will the charge flow and why ?
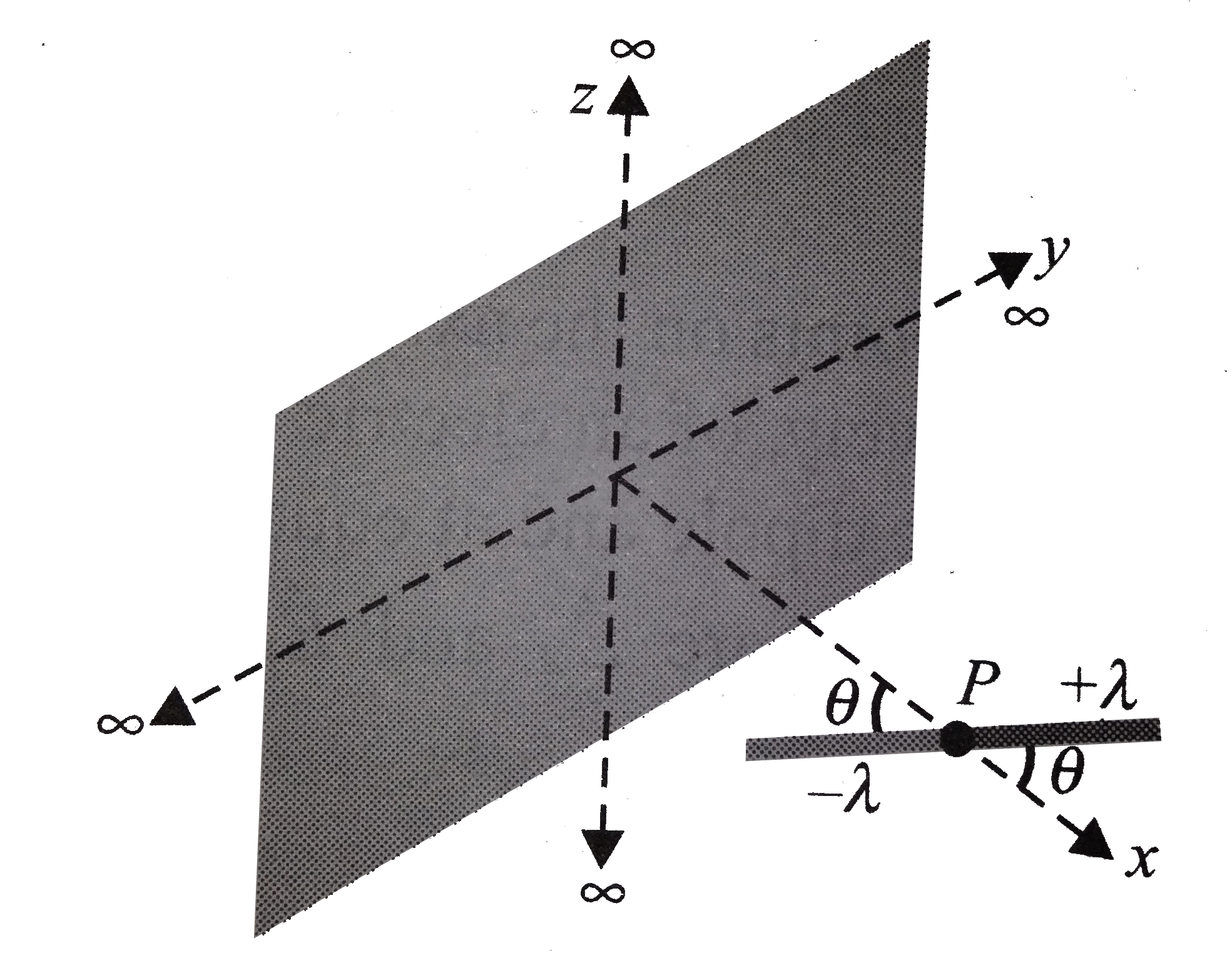
View SolutionA large sheet carries uniform surface charge density σ . A rod of length 2l has a linear charge density λ on one half and −λ on the second half. The rod is hinged at the midpoint O and makes an angle θ with the normal to the sheet. The torque experience by the rod is
View SolutionTwo conducting plates A and B are placed parallel to each other. A is given a charge. Q1 and B a charge Q2. Find the distribution of charges on the four surface.
View Solution
