White coherent light (400 nm- 700 nm) is sent through the slits of a young.s double slit experiment. The separation between the slits is 0.5mm and the screen is 50 cm away from the slits. There is a hole in the screen at a point 1 mm from the centre.
In the above problem which wavelength have a strong intensity at the hole?
To solve the problem, we need to determine which wavelengths of light will produce a strong intensity at a point 1 mm from the center of the screen in a Young's double slit experiment. We will use the formula for the position of bright fringes in the interference pattern.
1. Identify Given Values:
- Wavelength range: λ=400nm to 700nm
- Slit separation: d=0.5mm=0.5×10−3m
- Distance to screen: D=50cm=0.5m
- Distance from center to the hole: Yn=1mm=1×10−3m
2. Use the Formula for Bright Fringes:
The position of the nth bright fringe is given by:
Yn=nλDd
Rearranging this formula to find the wavelength λ:
λ=YndnD
3. Calculate for n=1:
Substitute n=1:
λ1=(1×10−3m)×(0.5×10−3m)1×0.5m
Simplifying this:
λ1=0.5×10−60.5=1×10−6m=1000nm
Since 1000nm is outside the given range (400 nm to 700 nm), we discard this wavelength.
4. Calculate for n=2:
Substitute n=2:
λ2=(1×10−3m)×(0.5×10−3m)2×0.5m
Simplifying this:
λ2=0.5×10−61=0.5×10−6m=500nm
Since 500nm is within the range of 400 nm to 700 nm, this wavelength will have a strong intensity at the hole.
5. Conclusion:
The only wavelength that will have a strong intensity at the hole is 500nm.
Final Answer:
The wavelength that has a strong intensity at the hole is 500 nm.
- Wavelength range: λ=400nm to 700nm
- Slit separation: d=0.5mm=0.5×10−3m
- Distance to screen: D=50cm=0.5m
- Distance from center to the hole: Yn=1mm=1×10−3m
The position of the nth bright fringe is given by:
Yn=nλDd
Rearranging this formula to find the wavelength λ:
λ=YndnD
Substitute n=1:
λ1=(1×10−3m)×(0.5×10−3m)1×0.5m
Simplifying this:
λ1=0.5×10−60.5=1×10−6m=1000nm
Since 1000nm is outside the given range (400 nm to 700 nm), we discard this wavelength.
Substitute n=2:
λ2=(1×10−3m)×(0.5×10−3m)2×0.5m
Simplifying this:
λ2=0.5×10−61=0.5×10−6m=500nm
Since 500nm is within the range of 400 nm to 700 nm, this wavelength will have a strong intensity at the hole.
The only wavelength that will have a strong intensity at the hole is 500nm.
The wavelength that has a strong intensity at the hole is 500 nm.
Topper's Solved these Questions
Similar Questions
White coheral light (400 nm 700 nm ) is sent through the slit of a Young.s double slit experiment. The separation between the slits is 0.5 mm and the screen is 50 away from the slits . There is a hole in the screen at a point 1.0 mm away (along the width of the fringes) from the central line. Which wavelengths (s) will be absent in the light coming from the hole ?
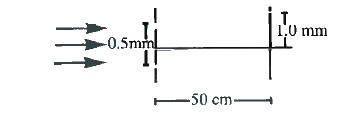 View Solution
View SolutionWhite light is used to illuminate the two slits in a Young's
double slit experiment. The separation between the slits is b and the screen is at
a distance d(>>b) from the slits. At a point on the screen directly in front of
one of the slits, certain wavelengths are missing. Some of these missing
wavelength are
View SolutionWhite light is used to illuminate the two slits in a Young's double slit experiment. The separation between the slits is b and the screen is at a distance d(>>b) from the slits At a point on the screen directly in front of one of the slits, certain wavelengths are missing some of these missing wavelengths are
View SolutionIn a Young's double slit experiment, the slit separation is 1mm and the screen is 1m from the slit. For a monochromatic light of wavelength 500nm, the distance of 3rd minima from the central maxima is
View SolutionWhite light is used to illuminate the two slits in a Young's double slit experiment. The separation between the slits is b and the screen is at a distance d(>>b) from the slits At a point on the screen directly in front of one of the slits, certain wavelengths are missing some of these missing wavelengths are
View SolutionIn the Young's double slit experiment, the spacing between two slits is 0.1mm. If the screen is kept at a distance of 1.0m from the slits and the wavelength of ligth is 5000Å, then the fringe width is
View SolutionIn Young's double slit experiement when wavelength used is 6000Å and the screen is 40cm from the slits, the fringes are 0.012cm wide. What is the distance between the slits?
View SolutionIn Young's double-slit experiment, the slit separation is 0.5 mm and the screen is 0.5 m away from the slit. For a monochromatic light of wavelength 500 nm, the distance of 3rd maxima from the 2nd minima on the other side of central maxima is
View SolutionWhite coherent light (400nm−700nm) is sent through the slits of a YDSE. d=0.5mm, D=50 cm. There is a hole in the screen at a point 1.0mm away (along the width of the fringes) from the central line.
(a) Which wavelength will be absent in the light coming from the hole?
(b) Which wavelength(s) will have a strong intensity?
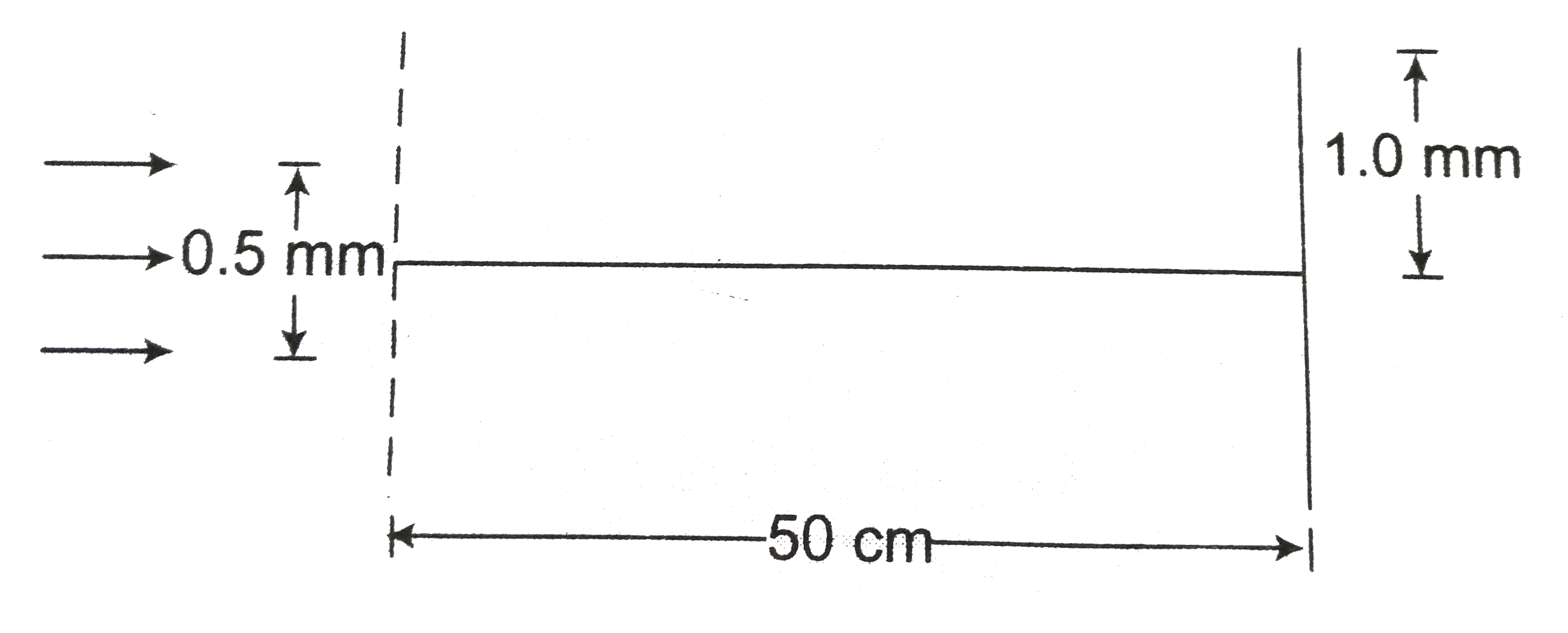 View Solution
View SolutionWhite coherent light (400nm−700nm) is sent through the slits of a YDSE. d=0.5mm, D=50 cm. There is a hole in the screen at a point 1.0mm away (along the width of the fringes) from the central line.
(a) Which wavelength will be absent in the light coming from the hole?
(b) Which wavelength(s) will have a strong intensity?
 View Solution
View Solution

