A cone of radius 8 cm and height 12 cm is divided into two parts by a plane through the mid-point of its axis parallel to its base Find the ratio of the volumes of two parts
The correct Answer is:1:7
Let ORN be the cone then , given radius of the base of the bases of the coner r1=8cm.
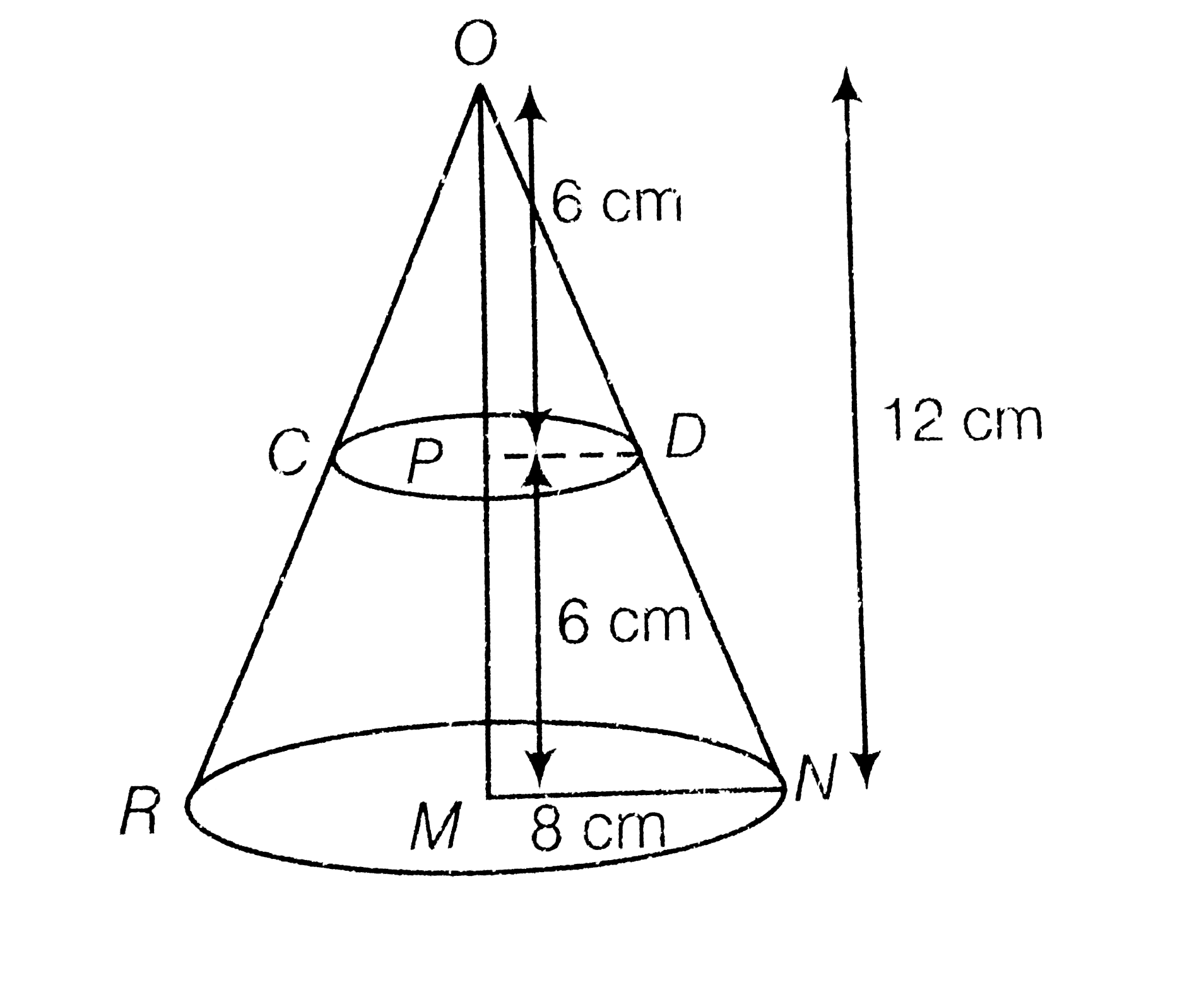
and height of the cone, (h) OM = 12 cm
Let P be the mid-point of OM, then
OP=PM=122=6cm
Now, ΔOPD~ΔOMN
∴ OPOM=PDMN
⇒ 612=PD8⇒12=PD8
⇒ PD=4cm
The plane along CD divides the cone into two parts , namely
(i) a smalle cone of radius 4 cm and height 6 cm and (ii) frustum of a cone for which
Radius of the top the frustum , r1=4cm
Radius of the bottom, r2=8cm
and height of the frustum, h = 6 cm
∴ Volume of smaller cone =(13π×4×4×6)=32πcm3
and volume of the frustum of cone =12×π×6[(8)2+(4)2+8×4]
=2π(64+16+32)=224πcm3
∴ Required ratio = volume of furstum : Volume of cone=224π:32π=1:7
Topper's Solved these Questions
Similar Questions
A cone of radius 8 cm and height 12 cm is divided into two parts by a plane through the mid-point of its axis parallel to its base Find the ratio of the volumes of two parts
View SolutionA cone of radius 8cm and height 12cm is divided into two parts by a plane through the mid-point of its axis parallel to its base. Find the ratio of the volumes of the two parts.
View Solution
Knowledge Check
- Question 1 - Select One
A cone of radius r сm and height h cm is divided into two parts by drawing a plane through the middle point of its height and parallel to the base. What is the ratio of the volume of the original cone to the volume of the smaller cone?
A4:1B8:1C2:1D6:1 - Question 2 - Select One
If a cone is cut into two parts by a horizontal plane passing through the mid-point of its axis, then the ratio of the volumes of the upper part and the given cone is
A1 : 2B1 : 4C1 : 6D1 : 8
A cone is divided in to two parts by drawing a plane through the mid point of its adis parallel to its base. Compare the volume of two parts.
View SolutionA cone of radius r сm and height h cm is divided into two parts by drawing a plane through the middle point of its height and parallel to the base. What is the ratio of the volume of the original cone to the volume of the smaller cone?
View SolutionIf a cone of radius 10cm is divided into two parts by drawing a plane through the mid-point of its axis, parallel to its base. Compare the volumes of the two parts.
View SolutionA solid cone of base radius 10 cm is cut into two parts through the mid-point of its height, by a plane parallel to its base. Find the ratio in the volumes of two parts of the cone.
View SolutionIf a cone is cut into two parts by a horizontal plane passing through the mid-points of its axis, find the ratio of the volume of the upper part and the cone.
View SolutionThe height of a cone is 10 cm. The cone is divided into two parts using a plane parallel to its base at the middle of its height. Find the ratio of the volumes of the two parts.
View Solution

