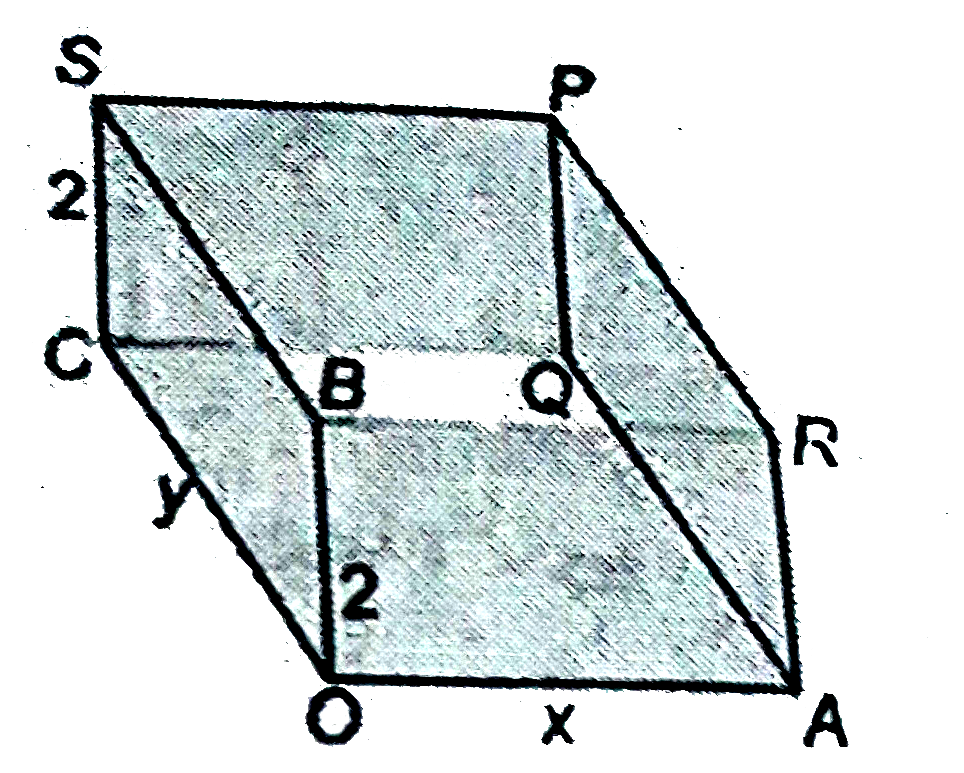
A tank with rectangular base and rectangular sides, open at the top is to be constructed so that its depth is 2 m and volume is 8 m3. If building of tank costs Rs 70 per square metre for the base and Rs 45 per square metre for sides, what is the cost of least expensive tank?

Video Solution

Text Solution

Verified by Experts

Let the length of tank be x metre, breadth by y metre and depth be 2 metre.
$∴$ Volume of tank = 2xy
$\Rightarrow 2 x y = 8$
$\Rightarrow x y = 4 \Rightarrow y = \frac{4}{x}$ ….(1)
$∴$ area of base = xy = 4 and area of four walls
$= 2 x + 2 y + 2 x + 2 y$
$= 4 x + 4 y = 4 (x + y)$
Given that cost of maunfacturing the base of tank is Rs. $70 / m^{2} and for walls, it is R s . 45 / m^{2}$ .
$∴$ Cost of manufacturing,
$C = R s . [70 \times x y + 45 \times 4 (x + y)]$
$= R s . [70 x y + 180 (x + y)]$
$C = 70 \times 4 + 180 (x + \frac{4}{x})$ [ From eqn.(1)]
$= 280 + 180 (x + \frac{4}{x})$
$\Rightarrow \frac{d C}{d x} = 0 + 180 (1 - \frac{4}{x^{2}}) = 180 (\frac{x^{2} - 4}{x^{2}})$
For minimum cost, $\frac{d C}{d x} = 0$
$\Rightarrow 180 (\frac{x^{2} - 4}{x^{2}}) = 0 \Rightarrow x^{2} = 4$
$\Rightarrow x = \pm 2$
Now $\frac{d^{2} C}{{d x}^{2}} = 180 \times \frac{8}{x^{3}}$
at $x = 2, \frac{d^{2} C}{{d x}^{2}} > 0$
$∴$ at x = 2, C is minimum.
$∴ a t x = 2, y = \frac{4}{x} = \frac{4}{2} = 2$
From equation (2), minimum cost of manufacture,
$= R s . [280 + 180 (2 + \frac{4}{2})]$
$= R s . [280 + 720] = R s . 1000$ .

Updated on:21/07/2023

|

Class 12 MATHS APPLICATION OF DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Priya used four similar rectangular carpets and a square carpet for the flooring in her study room as shown below.

If it cost her Rs. 6 per square metre for the rectangular carpets and Rs. 8 per square metre for the square carpet, how much did she pay in all ?
ARs. 512
BRs. 600
CRs. 417
DRs. 416

NAGEEN PRAKASHAN-APPLICATIONS OF DERIVATIVES-Miscellaneous Exercise

Home
Ask Doubt
Profile