ABC is a right angled triangle with ABC=90∘. D is any point on AB and DE is perpendicular to AC. Prove that:

Find, area of AADE: area of quadrilateral BCED.
The correct Answer is:1:8
Step by step video & image solution for ABC is a right angled triangle with ABC = 90^@. D is any point on AB and DE is perpendicular to AC. Prove that: Find, area of AADE: area of quadrilateral BCED. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(D)18videos
SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)
- BOOK - ICSECHAPTER - SIMILARITY EXERCISE - MULTIPLE CHOICE QUESTIONS (COMPETENCY BASED QUESTIONS)10videos
SIMILARITY
- BOOK - ICSECHAPTER - SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)EXERCISE - Exercise 6(e )18videos
SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)
Similar Questions
ABC is a right angled triangle with ABC=90∘. D is any point on AB and DE is perpendicular to AC. Prove that:
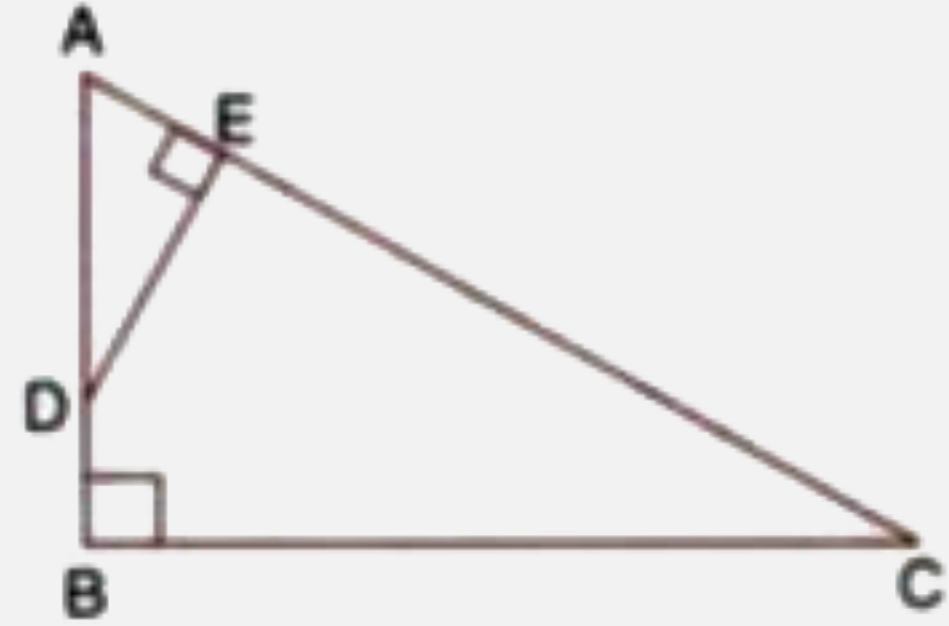
ΔADE~ΔACB.View SolutionABC is a right angled triangle with ∠ABC=90∘, D is any point on AB and DE is perpendicular to AC. Prove that :
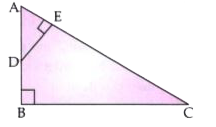
(iii) Find area of Δ ADE : area of quadrilateral BCED.If AC = 13 cm, BC = 5 cm and AE = 4 cm.View SolutionABC is a right angled triangle with ∠ABC=90∘, D is any point on AB and DE is perpendicular to AC. Prove that :

(i) ΔADE~ΔACB.View SolutionABC is a right angled triangle with ABC=90∘. D is any point on AB and DE is perpendicular to AC. Prove that:

If AC = 13 cm, BC = 5 cm and AE = 4 cm. Find DE and AD.View SolutionABC is a right angled triangle with ∠ABC=90∘, D is any point on AB and DE is perpendicular to AC.
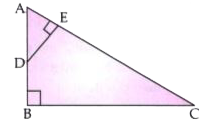
(ii) If AC = 13 cm, BC = 5 cm and AE = 4 cm. Find DE and AD.View SolutionABC is a right triangle right-angled at B. Let D and E be any points on AB and BC respectively. Prove that AE2+CD2=AC2+DE2
View SolutionIn triangle ABC, AB=AC and BD is perpendicular to AC. Prove that : BD2−CD2=2CD×AD
View SolutionThe median BE and CF of a triangle ABC intersect at G. Prove that the area of ΔGBC= area of the quadrilateral AFGE.
View SolutionIn a right triangle ABC, right angled at A, AD is drawn perpendicular to BC. Prove that:
AB2−BD2=AC2−CD2View SolutionABC is a triangle in which AB=ACandD is a point on AC such that BC2=AC×CD.Prove that BD=BC.
View Solution

