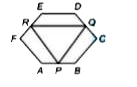
ABCD is a rhombus and P, Q, R and S are wthe mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.

Video Solution

Text Solution

Verified by Experts

Given, ABCD is a rhombus and P, Q, R and S are mid-points of AB, BC, CD and DA.
$∴$ by mid-point theorem,
$I n Δ A D C, S R || A C and S R = \frac{1}{2} A C ... (1)$
$I n Δ A B C, P Q || A C and P Q = \frac{1}{2} A C ... (2)$
From (1) and (2), we get
$P Q || S R and P Q = S R = \frac{1}{2} A C$
$∴ P Q R S is a parallelogram. (∵ one pair of opposite sides is equal and parallel)$
Now, we know that diagonals of a rhombus bisect each other at right angles.
$∴ ∠ E O F = 90^{\circ}$
$N o w, R Q || B D (by mid-point theorem)$
$\Rightarrow R E || F O$
$A l s o, S R || A C [from (1)]$
$\Rightarrow F R || O E$
$∴ O E R F is parallelogram. (∵ pairs of opposite sides are parallel)$
$S o, ∠ E R F = ∠ E O F = 90^{\circ}$
$(∵ pairs of opposite sides are parallel)$
Thus, PQRS is a parallelogram with $∠ R = 90^{\circ} .$
Hence, PQRS is a rectangle.

If ABCD be a rectangle and P, Q, R, S be the mid-points of AB, BC, CD and DA respectively, then the area of the quadrilateral PQRS is equal to
A $\frac{1}{3}$ are (ABCD)
B $\frac{3}{4}$ area (ABCD)
C $\frac{1}{2}$ area (ABCD)
Darea (ABCD)
M and N are the mid-points of the diagonals AC and BD respectively of quadrilateral ABCD, then $A B + A D + C B + C D$ is equal to
A2 MN
B2NM
C4 MN
D4 NM