An are of the catenary y=a2(exa+e−xa)=acoshxa,
whose end-points have abscissas 0 and x, respectively, revolves about the x-axis. Show that the surface area P and the volume V of the solid thus generated are related by the formula P=2Va
The correct Answer is:P=2Va
Topper's Solved these Questions
- APPLICATIONS OF THE DEFINITE INTEGRALBOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Geometrical Applications of the Definite Integral
10 Videos
- APPLICATIONS OF THE DEFINITE INTEGRALBOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Computing Pressure, Work and Other Physical Quantities by the Definite Integrals
7 Videos
- APPLICATIONS OF THE DEFINITE INTEGRALBOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - The Arc Length of a Curve in Polar Coordinates
7 Videos
- APPLICATION OF DIFFERENTIAL CALCULUS TO INVESTIGATION OF FUNCTIONSBOOK - IA MARONCHAPTER - APPLICATION OF DIFFERENTIAL CALCULUS TO INVESTIGATION OF FUNCTIONSEXERCISE - ADDITIONAL PROBLEMS
9 Videos
- BASIC CLASSES OF INTEGRABLE FUNCTIONS BOOK - IA MARONCHAPTER - BASIC CLASSES OF INTEGRABLE FUNCTIONS EXERCISE - 5.8 INTEGRATION OF OTHER TRANSCENDENTAL FUNCTIONS
5 Videos
Similar Questions
The figure bounded by an arc of the sinusoid y= sin x, the axis of ordinates and the straight line y=1 resolves about the y-axis
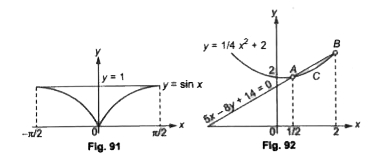
Compute the volume V of the solid of revolution thus generated.
View SolutionThe planer region bounded by the parabola y=2x2+3, the x-axis and the verticals x=0 and x=1 revolves about the y-axis. Compute the volume of the solid of revolution thus generated.
View Solution
Knowledge Check
The part of straight line y=x+1 beween x=2 and x=3 is revolved about x axis then the curved surface of solid thus generated is
A37π3B7π√3C37πD7π√2The part of straight line y=x+1 beween x=2 and x=3 is revolved about x axis then the curved surface of solid thus generated is
A37π3B7π√3C37πD7π√2The volume of the solid generated by the revolution of the curve y=a3a2+x2 about x-axis is :
A12π3a2Bπ3a2C12π2a3Dπ2a3
Compute the volume of the solid generated by revolving about the y-axis the figure bounded by the parabolas y=x2and8x=y2
View SolutionFind the coordinates of the centre of gravity of the catenary y=12(ex+e−x)= cos h x between A(0, 1) and B(a, cosh a).
View SolutionGiven: the cycloid x=a(t−sint),y=a(1−cost),0≤t≤2π
Compute. (a) the areas of the surface formed by revolving the arc OBA about the x and y-axis,
(b) the volumes of the solids generated by revolving the figure OBAO about the y-axis and the axis BC,
(c ) the area of the surface generated by revolving the are BA about the axis BC,
(d) the volume of the solid generated by revolving the figure ODBEABO about the tangent line DE touching the figure at the vertex B,
(e ) the area of the surface formed by revolving the are of the cycloid (see item (d)]
View SolutionAn isosceles triangle of a given perimeter 2p=12 revolves about its base. Write the function V(x), where V is the volume of the solid of revolution thus obtained and x is the length of the lateral side of the triangle.
View SolutionThe volume of the solid generated by the revolution of the curve y=a3a2+x2 about x-axis is :
View Solution
