Assertion: The solution set of −1<3+4x≤23,x∈R on the number line is
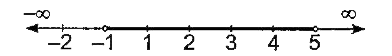
Reason: In the solution set, the number -1 is not included and the number 5 is included.
A
Both assertion and reason are correct and reason is the correct explanation of assertion
B
Both assertion and reason are correct but reason is not the correct explanation of assertion
C
Assertion is correct but reason is incorrect
D
Assertion is incorrect but reason is correct
Text Solution
The correct Answer is:A
|
Topper's Solved these Questions
- LINEAR INEQUATIONSBOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - Competency Based Questions
15 Videos
- LINEAR INEQUATIONSBOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - Multiple Choice Questions
47 Videos
- HEIGHTS AND DISTANCES BOOK - ICSECHAPTER - HEIGHTS AND DISTANCES EXERCISE - Exercise 22 C
28 Videos
- LOCI (LOCUS AND ITS CONSTRUCTIONS)BOOK - ICSECHAPTER - LOCI (LOCUS AND ITS CONSTRUCTIONS)EXERCISE - EXERCISE 16(B)
49 Videos
Similar Questions
Knowledge Check
The solution set of −1≤3+4x<23,x∈W is
A{0, 1, 2, 3, 4}B{−1,0,1,2,3,4}C{0,1,2,3,4,5}D{−1,0,1,2,3,4,5}The solution set of 11x−4<15x+4≤13x+14,x∈I, on the number line is
A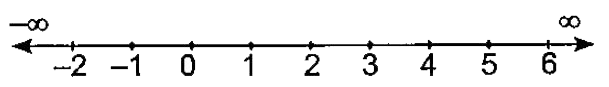 B
B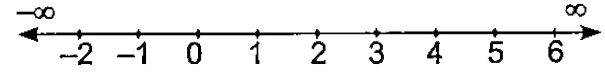 C
C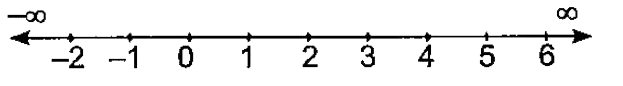 D
D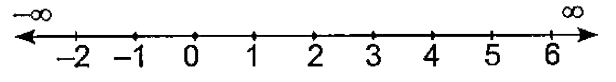
The solution set of 2x+13<3x−25x∈R on the number line is
A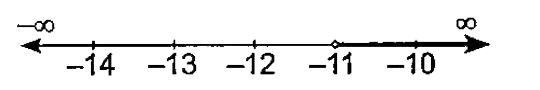 B
B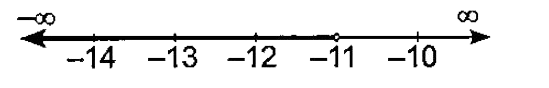 C
C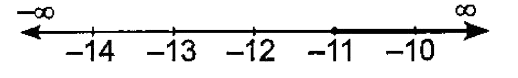 D
D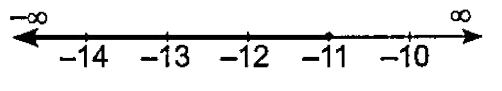
The solution set of 6≤−3(2x−4)<12,x∈R is
View SolutionThe solution set of 3x5+2<x+4≤x2+5,x∈R on the number line is:
View SolutionThe solution set of −812<−12−4x<712,x∈W on the number line is:
View SolutionIf −1<3+4x≤23,x∈R (real numbers), then the solution set of x is
View SolutionAssertion: The solution set of 2x−3≤x+1≤4x+7,x∈N is {1, 2, 3,4} .
Reason: If the solution of a linear inequation is x>2,x∈R then on the number line, it is represented as
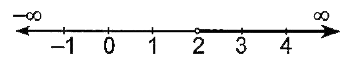
View Solution
