BE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles.
To prove that triangle ABC is isosceles using the RHS (Right angle-Hypotenuse-Side) congruence rule, we will follow these steps:
Step 1: Identify the Right Triangles
We have triangle ABC with altitudes BE and CF from points B and C to side AC. We will consider the two right triangles:
- Triangle BEC (where BE is the altitude)
- Triangle CFB (where CF is the altitude)
Step 2: Establish Right Angles
Since BE and CF are altitudes, we know that:
- Angle EBF = 90 degrees (BE is perpendicular to AC)
- Angle FCB = 90 degrees (CF is perpendicular to AC)
Step 3: Identify the Common Side
Both triangles BEC and CFB share the side BC. Therefore, we have:
- BC = BC (common side)
Step 4: Use Given Information
According to the problem, the altitudes BE and CF are equal. Thus, we have:
- BE = CF (given)
Step 5: Apply the RHS Congruence Rule
Now we can apply the RHS congruence rule:
- We have two right angles (Angle EBF and Angle FCB are both 90 degrees).
- The hypotenuses (BE and CF) are equal.
- The common side (BC) is equal.
Thus, we can conclude that:
- Triangle BEC is congruent to triangle CFB by the RHS congruence rule.
Step 6: Conclude with Angles
By the property of congruent triangles (CPCTC), we know that:
- Angle BEC = Angle CFB
Since angles opposite to equal sides in congruent triangles are equal, we have:
- Angle B = Angle C
Step 7: State the Isosceles Triangle Property
Since angles B and C are equal, triangle ABC is isosceles by definition (a triangle with at least two equal angles is isosceles).
Conclusion
Therefore, we have proved that triangle ABC is isosceles.
---
We have triangle ABC with altitudes BE and CF from points B and C to side AC. We will consider the two right triangles:
- Triangle BEC (where BE is the altitude)
- Triangle CFB (where CF is the altitude)
Since BE and CF are altitudes, we know that:
- Angle EBF = 90 degrees (BE is perpendicular to AC)
- Angle FCB = 90 degrees (CF is perpendicular to AC)
Both triangles BEC and CFB share the side BC. Therefore, we have:
- BC = BC (common side)
According to the problem, the altitudes BE and CF are equal. Thus, we have:
- BE = CF (given)
Now we can apply the RHS congruence rule:
- We have two right angles (Angle EBF and Angle FCB are both 90 degrees).
- The hypotenuses (BE and CF) are equal.
- The common side (BC) is equal.
- Triangle BEC is congruent to triangle CFB by the RHS congruence rule.
By the property of congruent triangles (CPCTC), we know that:
- Angle BEC = Angle CFB
- Angle B = Angle C
Since angles B and C are equal, triangle ABC is isosceles by definition (a triangle with at least two equal angles is isosceles).
Therefore, we have proved that triangle ABC is isosceles.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
BE and CF are two altitudes of a triangle ABC. If AB=6cm,AC=5cmand CF=4cm, then the length of BE is
A4.8 cmB7.5 cmC3.33 cmD5.5 cm - Question 2 - Select One
If AD, BE, CF are the altitudes of ΔABC whose orthocentre is H, then C is the orthocentre of :
A△ABHB△BDHC△ABDD△BEA
If the altitudes from two vertices of a triangle to the opposite sides are equal, prove that the triangle is isosceles.
In△ABEand △ACF∠AEB=∠AFC(900each)
∠BAE=∠CAF(common angle)
∠ABE=∠ACF using angle sum property
BE=CF,△ABE≅△ACF
⇒AB=AC
Hence triangle ABC is an isosceles tangent as two sides are equal to each otherView SolutionIf in a ΔABC,c(a+b)cosB/2=b(a+c)cosC/2, prove that the triangle is isosceles.
View SolutionIn △ABC, if cosA+sinA−2cosB+sinB=0 then prove that triangle is isosceles right angled.
View SolutionIn figure, AD and BE are respectively altitudes of an isosceles triangle ABC with AC=BC . Prove that AE=BD.
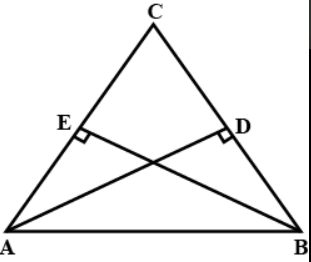 View Solution
View SolutionIf AD, BE, CF are the altitudes of triangle ABC whose ortho centre is H then C is ortho centre of
View SolutionIn a ΔABC if (a+b)cos(B2)=b(a+c)cos(C2) then prove that the triangle ABC is isosceles.
View Solution

