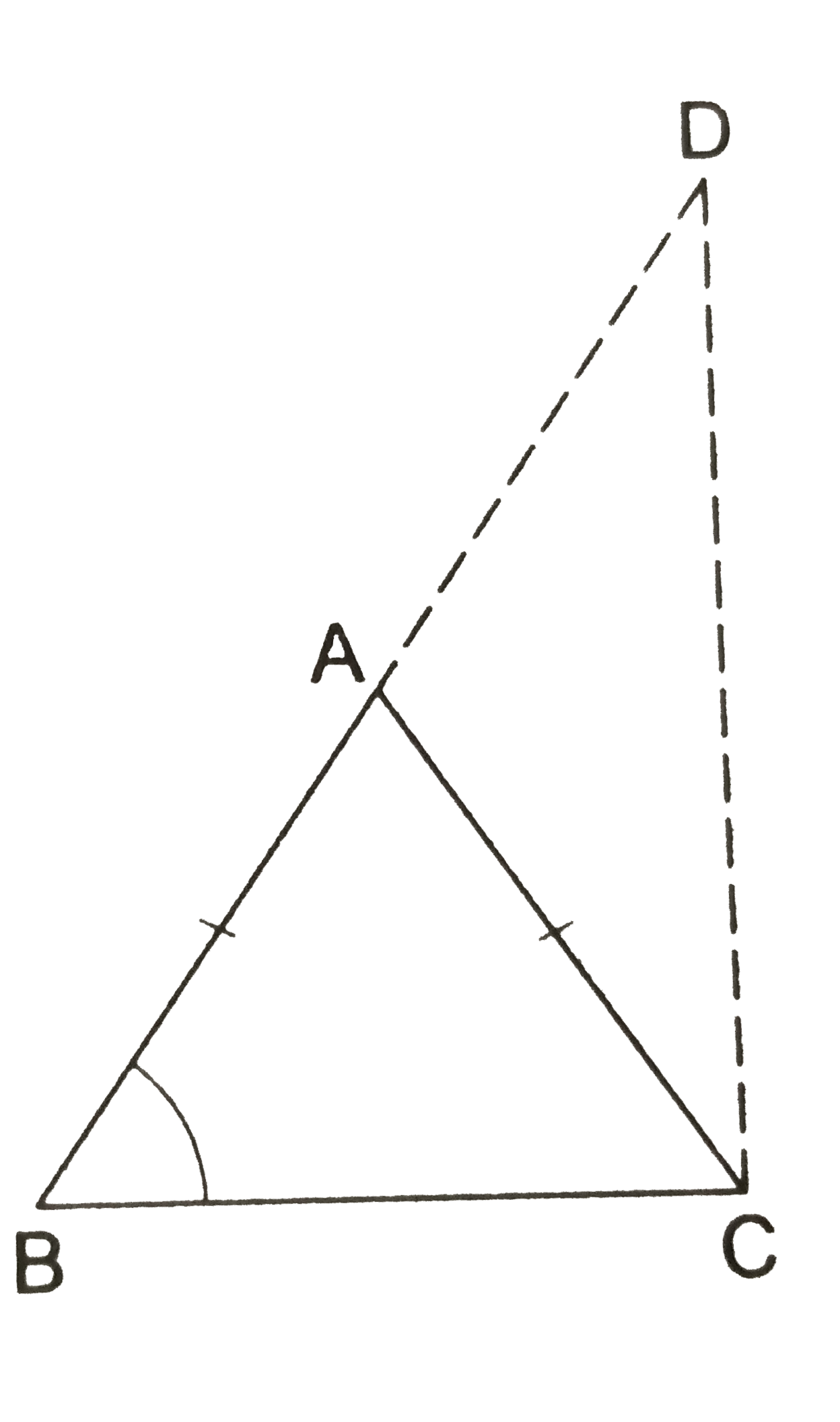
is an isosceles triangle in which . Side BA is produced to D such that (see Fig. 7.34). Show that is a right angle.
Video Solution
Text Solution
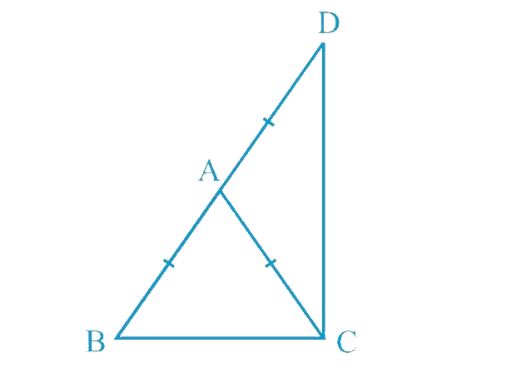
In isosceles triangle ABC,
(Given)
(Angles opposite to equal sides of a triangle are equal)
Let be x. ----------- (1)
In ,
(Since, AB = AD)
(Angles opposite to equal sides of a triangle are equal)
Let be y. ----------- (2)
Thus, ----------- (3)
In ,
(Angle sum property of a triangle)
Substituting the values we get,
[From equation (1), (2) and (3)]
[From equation(3)]
∴
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
ΔABC is an isosceles triangle with AB=AC, side BA is produced to D such that AB=AD. Prove that ∠BCD is a right angle.
A60∘B90∘C120∘Dcan't be determined - Question 2 - Select One
ΔABC is an isosceles triangle with AB=AC, side BA is produced to D such that AB=AD. Prove that ∠BCD is a right angle.
A60∘B90∘C120∘Dcan't be determined - Question 3 - Select One
ABC is an isosceles triangle with AB = AC. Side BA is produced to D such that AB=AD. Find the ∠BCD:
A45∘B90∘C30∘D60∘
ΔABC is an isosceles triangle with AB=AC, side BA is produced to D such that AB=AD. Prove that ∠BCD is a right angle.
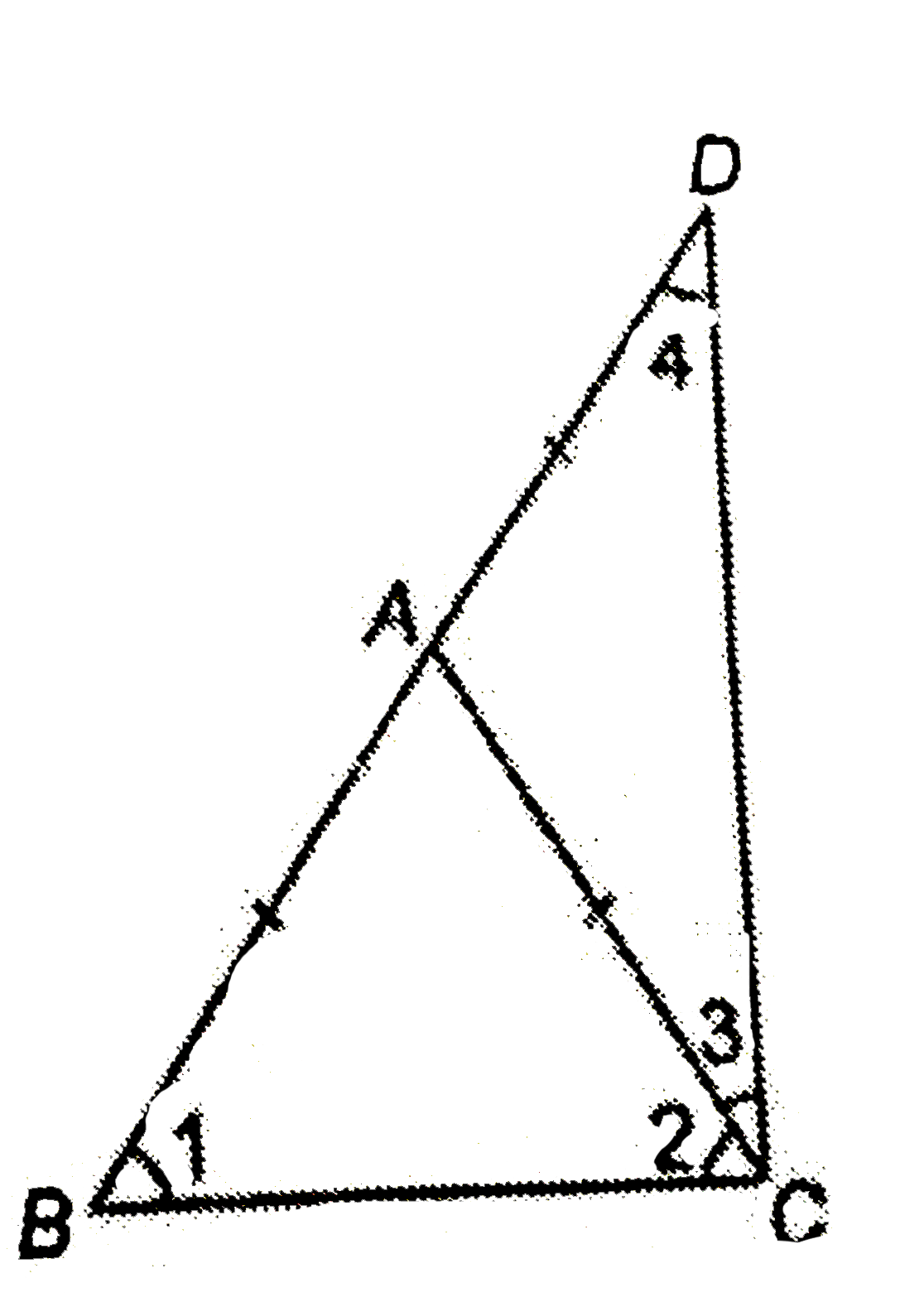 View Solution
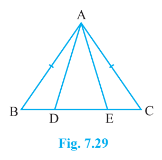
View SolutionIn an isosceles triangle ABC with AB=AC, D and E are points on BCsuch that BE=CD(see Fig. 7.29). Show that AD=AE
 View Solution
View SolutionD is a point on side BC of ΔABCsuch that AD = AC(see Fig. 7.47).Show that AB > AD.
View SolutionABC is an isosceles triangle in which AB=AC. AD bisects exterior angle PAC and CD∣∣AB. Show that(i) ∠DAC=∠BCA and (ii) ABCD is a parallelogram.
View SolutionABC is an isosceles triangle with AB = AC. Side BA is produced to D such that AB=AD. Find the ∠BCD:
View Solution