Consider the graph of the function f(x)=x+√|x| Statement-1: The graph of y=f(x) has only one critical point Statement-2: f'(x) vanishes only at one point
Statement 1 is true, Statement 2 is true, Statement 2 is a correct explanation for Statement 2 is a correct explanation for Statement 1.
Statement 1 is true, Statement 2 is true, Statement 2 is not a correct explanation for Statement 1.
Statement 1 is true, Statement 2 is false.
Statement 1 is false, Statement 2 is true.
The correct Answer is:D
f(x)={x+√xif≥0x+√−xifx<0
The graph of f(x) is shown with f'(x)=0 as x=−1/4. Also derivative fails at x = 0. Hence there are two cirtical points.

Topper's Solved these Questions
- MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSBOOK - CENGAGECHAPTER - MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSEXERCISE - Multiple Correct Answer Type
10 Videos
- MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSBOOK - CENGAGECHAPTER - MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSEXERCISE - Comprehension Type
6 Videos
- MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSBOOK - CENGAGECHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - JEE Advanced Previous Year
17 Videos
- PAIR OF STRAIGHT LINESBOOK - CENGAGECHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Exercise (Numerical)
5 Videos
Similar Questions
Knowledge Check
If the graph of the function y=f(x) is as shown :

The graph of y=1/2(|f(x)|−f(x)) isA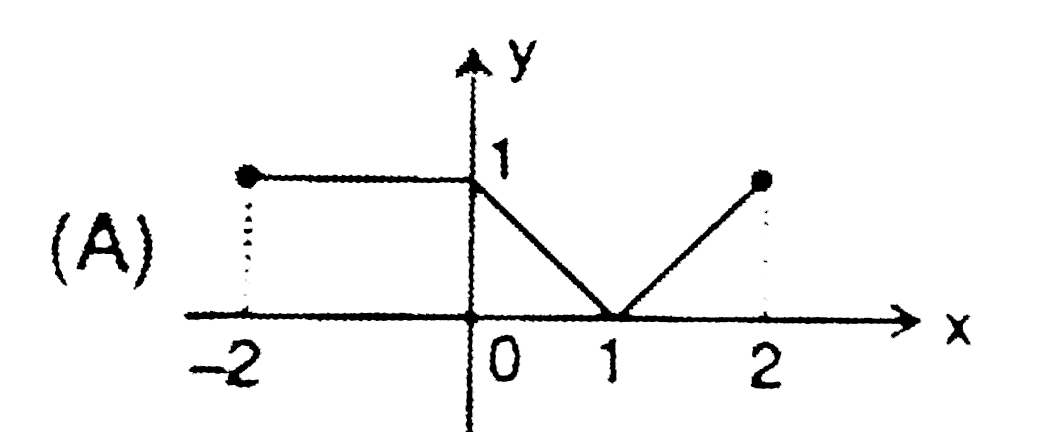 B
B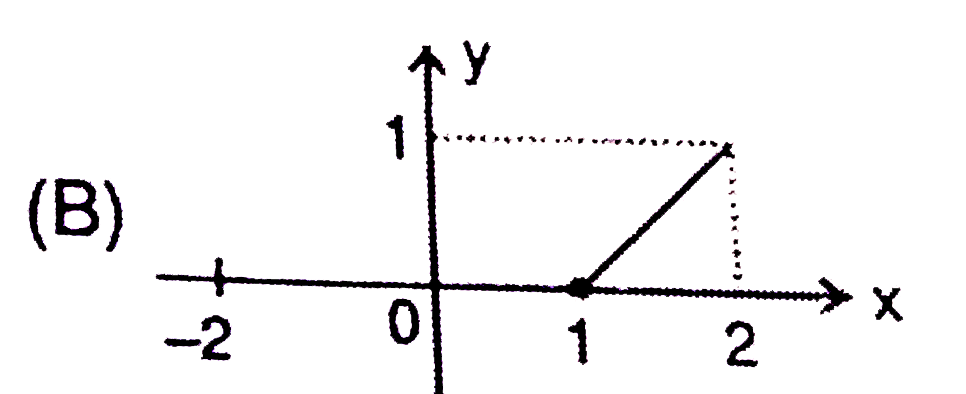 C
C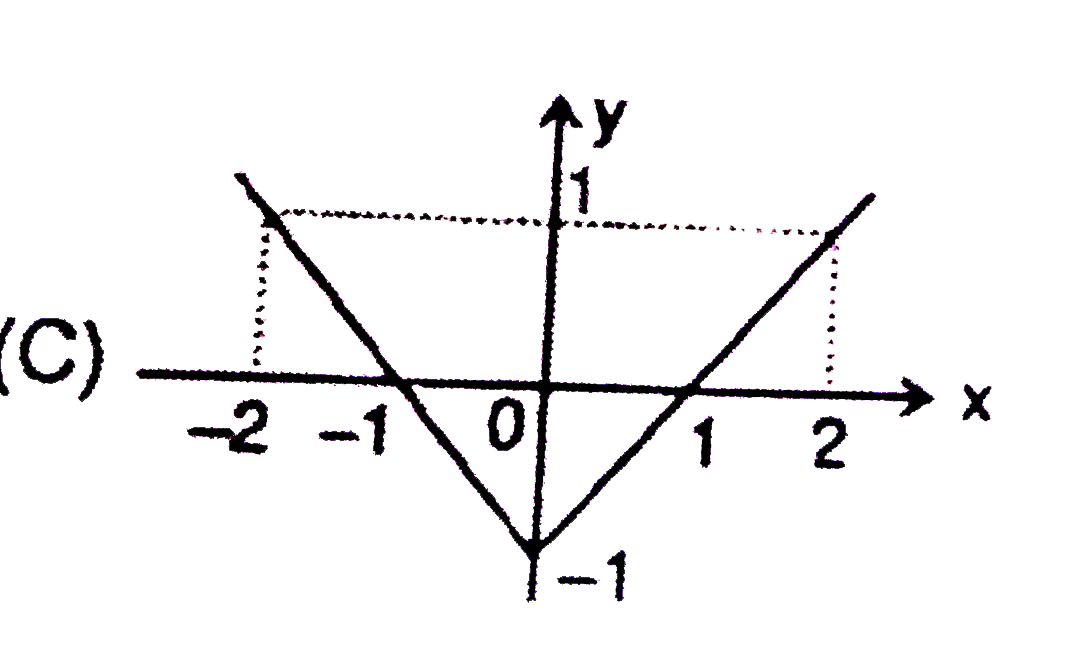 D
D
The graph of the function y=f(x) is symmetrical about the line x=2, then :
Af(x+2)=f(x-2)Bf(2+x)=f(2-x)Cf(x)=f(-x)Dnone of these
If the graph of the function y=f(x) is as shown : the graph of y=12(|f(x)|−f(x)) is
View SolutionIf the graph of the function y = f(x) is symmetrical about the line x = 2, then
View SolutionDraw the graph of the function f(x)=x−∣∣x−x2∣∣,−1≤x≤1 and find the points of non-differentiability.
View SolutionConstruct the graph of the function y=f(x−1)+f(x+1) where f(x)=1−|x| where |x|≤1, f(x)=0 where |x|>1
View SolutionNumber of critical point of the function f(X) =x+√|x| is _______.
View SolutionConsider the function f(x)=(.x+1C2x−8)(.2x−8Cx+1) Statement-1: Domain of f(x) is singleton. Statement 2: Range of f(x) is singleton.
View Solution
