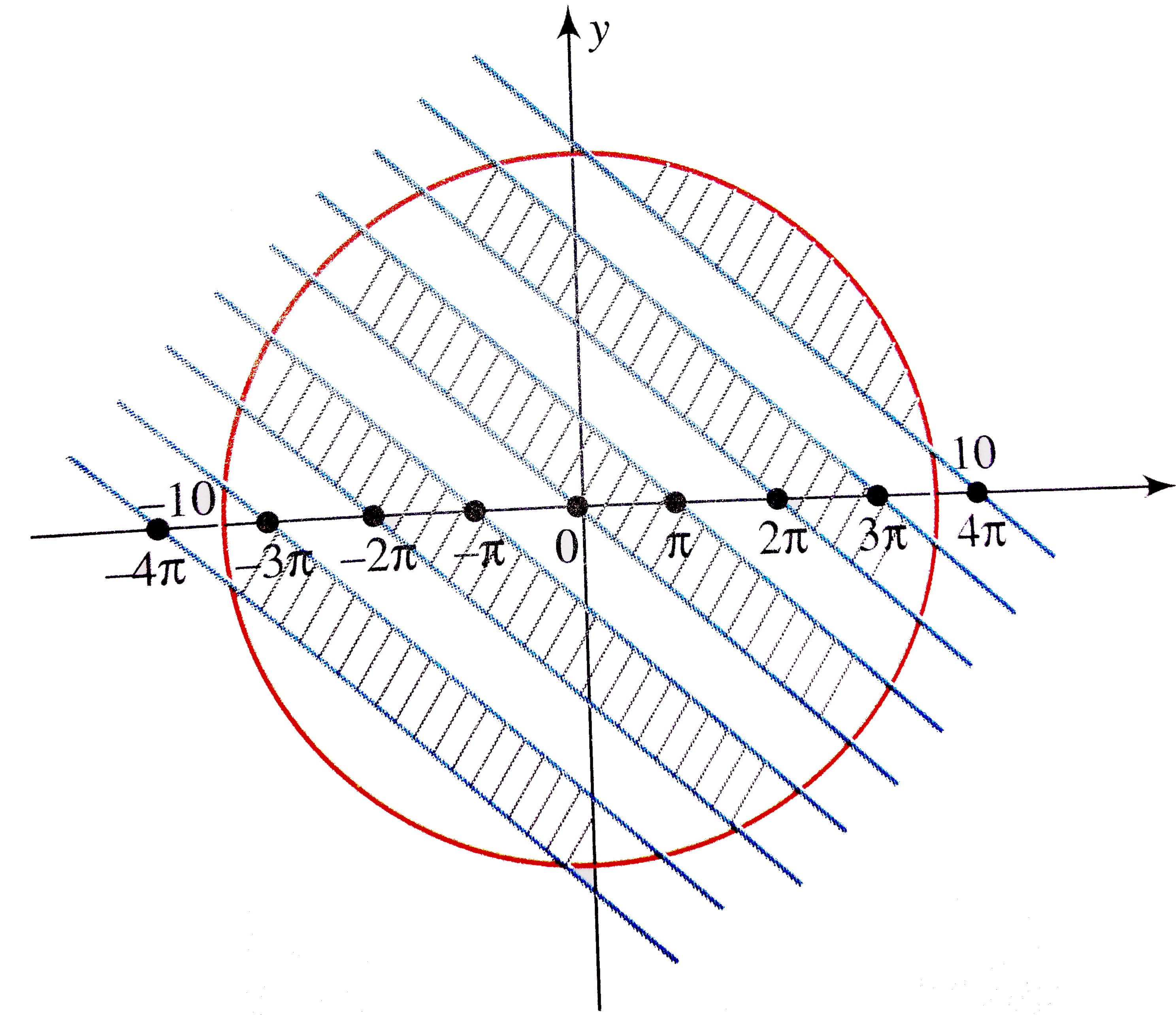
Consider the regions A={(x,y)∣x2+y2≤100}andB={(x,y)∣sin(x+y)>0} in the plane. Then the area of the region A∩B isSolution in Bengali
x2+y2≤100
So the points lie inside the circle or on the circle.
sin(x+y)>0
x+y∈(0,π)∪(2π,3π)…
x+y=c is the equation of the line
Required area= shaded region = 12π(10)2=50π
Topper's Solved these Questions
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - EXERCISES
34 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises
17 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercises
22 Videos
Similar Questions
Draw the graph of y=1(1/x).
View SolutionDraw the graph of y=(x−1)(x2−x+1).
View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
View SolutionFind the area of the region {(x,y) :x2+y2≤4, x+y ≥2}
View SolutionFind the area of the region {(x,y):x^(2)<=y<=x}
View SolutionConsider the region formed by the lines x=0,y=0,x=2,y=2. If the area enclosed by the curves y=exandy=1nx, within this region, is being removed, then find the area of the remaining region.
View SolutionSketch the region on x-y plane satisfying : (a) [y]=sin x(b)y={cos x}(-pi<=x<=pi)
View SolutionFind the area of the region {(x,y):x2+y2≤1≤x+y}
View SolutionConsider the regions A={(x,y)∣x2+y2≤100} and ={(x,y)∣sin(x+y)>0} in the plane. Then the area of the region A∩B is -
View SolutionConsider the regions A={(x,y)∣x2+y2≤100}andB={(x,y)∣sin(x+y)>0} in the plane. Then find the area of the region A∪B.
View SolutionConsider the regions A={(x,y)∣x2+y2≤100}andB=|xy|sin(x+y)>0} in the plane. Then the area of the region A∩B is
View SolutionThe area of the region bounded by the curves x=y2−2 and x=y is
View SolutionThe area of the region bounded by the curves x=y2−2 and x=y is
View SolutionIf the regions A and B are given by A={(x,y)/:y>x}B={(x,y):y<2−x2} then the area of A∩B is
View SolutionThe area of the region bounded by y=x2 and y=−x2+2 is
View SolutionThe area of the region bounded by y=x−[x],y=[x] in [0, 2] is
View Solution
