ΔDEF is an equilateral triangle. Seg DP⊥ side EF, and E-P-F. Prove that: DP2=3EP2

Step by step video & image solution for DeltaDEF is an equilateral triangle. Seg DPbot" side "EF, and E-P-F. Prove that: DP^(2)=3EP^(2) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PYTHAGORAS THEOREM BOOK - TARGET PUBLICATIONCHAPTER - PYTHAGORAS THEOREM EXERCISE - Aditional Problems for Practice(Based on the Practice Set 2.2)
5 Videos
- PYTHAGORAS THEOREM BOOK - TARGET PUBLICATIONCHAPTER - PYTHAGORAS THEOREM EXERCISE - Chapter Assessment
16 Videos
- PYTHAGORAS THEOREM BOOK - TARGET PUBLICATIONCHAPTER - PYTHAGORAS THEOREM EXERCISE - Multiple Choice Questions
18 Videos
- PROBABILITYBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITYEXERCISE - Try This
1 Videos
- QUADRATIC EQUATIONSBOOK - TARGET PUBLICATIONCHAPTER - QUADRATIC EQUATIONSEXERCISE - Additional problems for Practice : Based on Practice set 2.6
30 Videos
Similar Questions
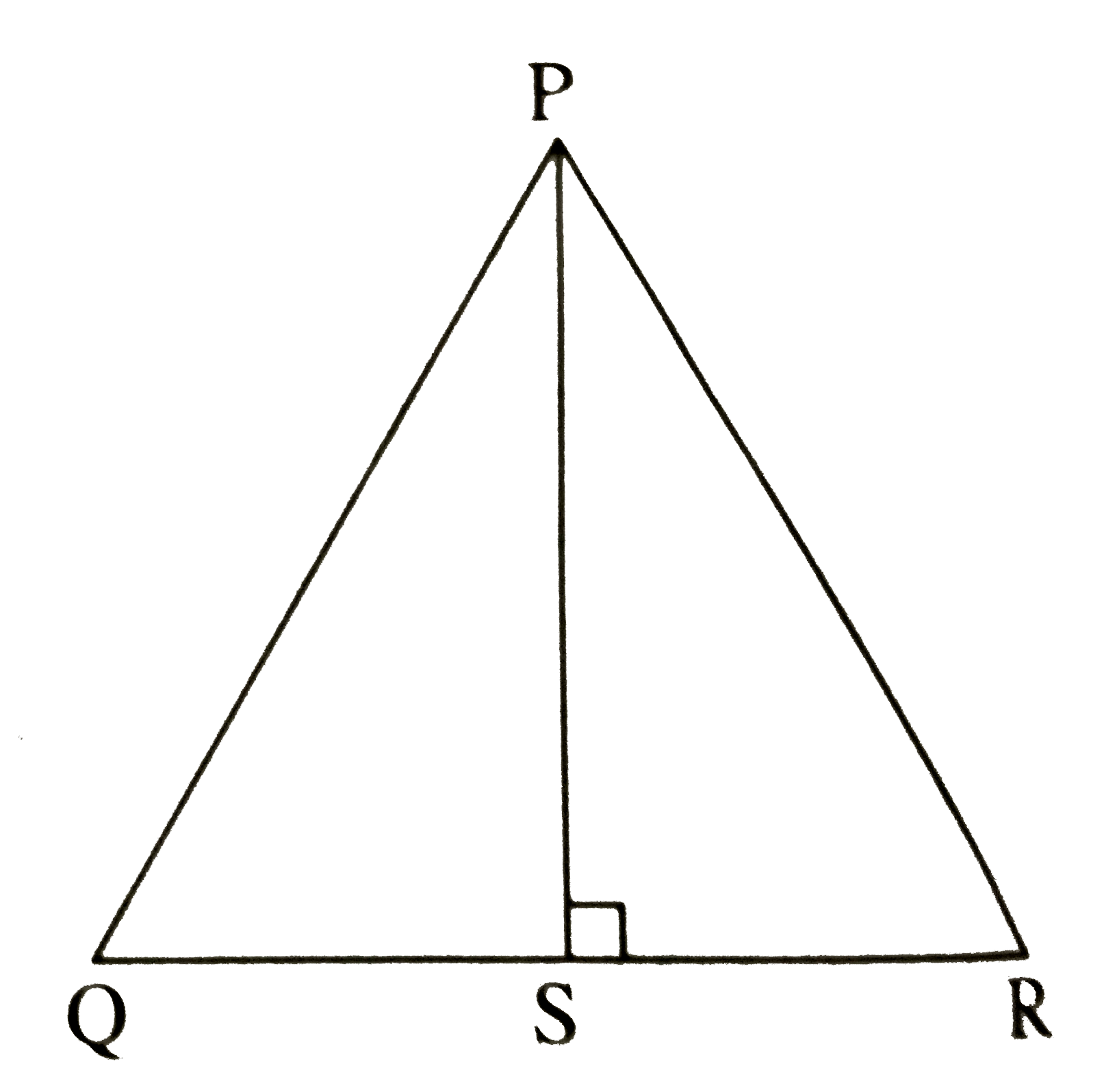
ΔPQR is an equilateral triangle. Seg PS⊥ side QR such that Q−S−R. Prove PS2=3QS2 by completing the following activity.
In ΔPQS,
∠PSQ=□……(Given)
∠Q=□………….(Angle of an equilateral triangle)
∴∠QPS=30∘.................(Remaining angle of ΔPQS)
∴ΔPQS is a □ triangle
PS=□PQ.............(Side opposite to 60∘)..............(1)
and QS=□PQ..........(Side opposite to 30∘)
PQ=2QS .....(2)
Substituting value of PQ from (2) in (1)
PS=√32×2QS
∴PS=□QS
∴PS2=3QS2......(Square both the sides)
View SolutionIn an equilateral triangle ABC the side BC is trisected at D . Prove that 9 AD2=7 AB2
View Solution
Knowledge Check
What is the area of an equilateral triangle with side 2cm?
A4√3cm2B3√3cm2C6cm2D√3cm2The area of an equilateral triangle with side 2√3 cm is
A5.196 cm2B0.866 cm2C3.496 cm2D1.732 cm2
Find the height of an equilateral triangle having side 2a
View SolutionIn the adjoining figure, ΔPQR is an equilateral triangle. Point S is on seg QR. such that QS=13QR. Prove that : 9PS2=7PQ2.
View SolutionIn the figure ΔPQR is an equilateral triangle. Point S is on seg QR such that
QS=13QR.
Prove that : 9PS2=7PQ2

View SolutionΔABC is an equilateral triangle. Point D is on seg BC such that BD=15AC. Prove : that 25AD2=21AB2
View SolutionProve that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
Use the above theorem, in the following.
If ABC is an equilateral triangle with AD⊥BC, then prove that AD2=3DC2.
View SolutionIn an equilateral triangle with side a, prove that area =√34a2
View Solution
