Find the area of the triangle formed by the tangents from the point (4, 3) to the circle x2+y2=9 and the line joining their points of contact.
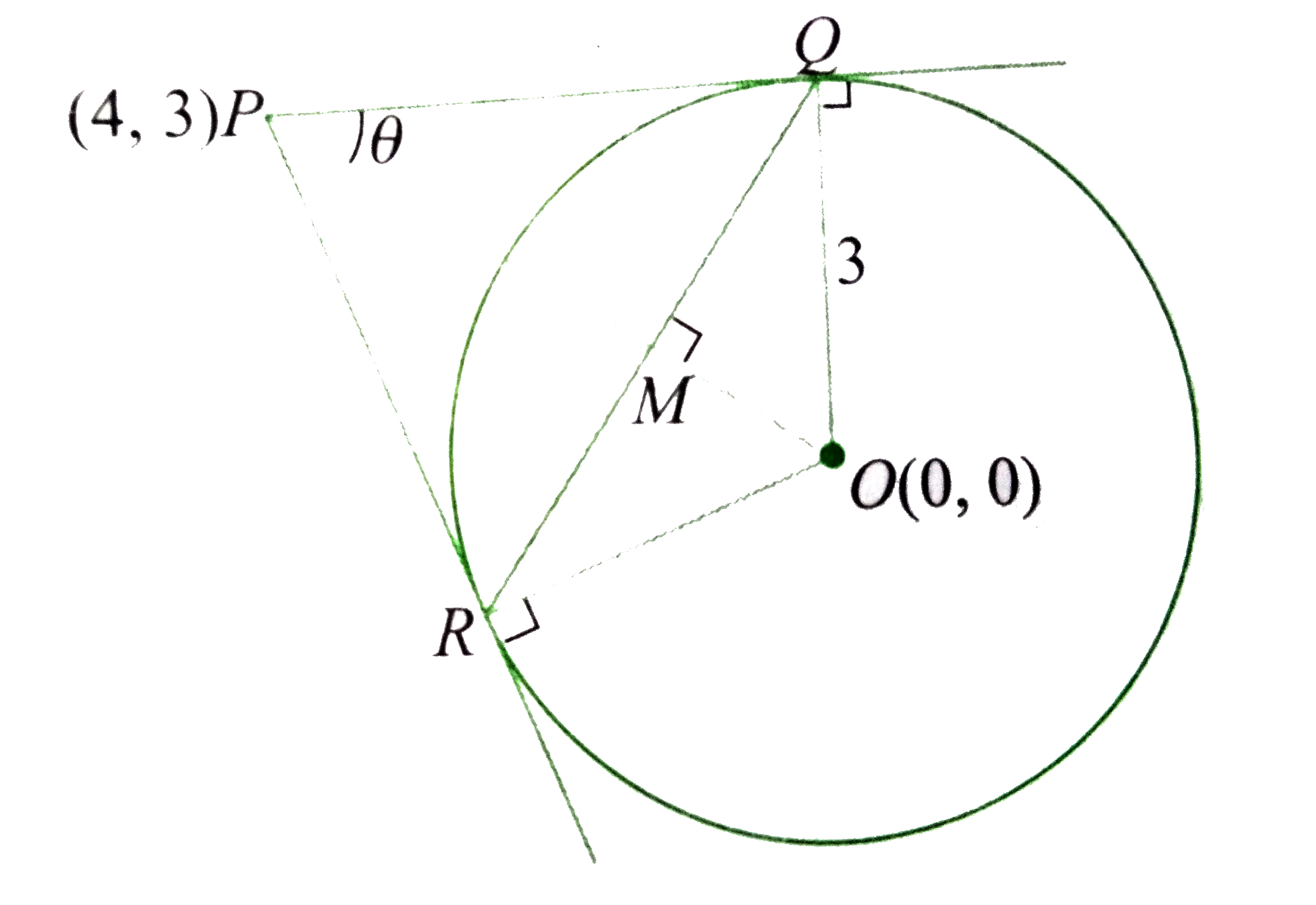
In the figure, OP=5 .
∴PQ=4.
In triangel OQP, tanθ=34
∴Area of triangle PQR=12PQ×PRsin2θ
=12×4×4×2tanθ1+tan2θ
=82(32)1+(34)2=19225 sq. unit
Topper's Solved these Questions
Similar Questions
Find the area of the triangle formed by the tangents drawn from the point (4,6) to the circle x2+y2=25 and their chord of contact.
Answer: 405√352 sq. units
View SolutionThe area of the triangle formed by the tangent at the point (a,b) to the circle x2+y2=r2 and the co-ordinate axes is:
View Solution
Knowledge Check
The area of the triangle formed by the tangents from the point (4,3) to the circle x2+y2=9 and the line joining their points of contact in sq. units is
A13225B16225C19225DnoneThe area of the triangle formed by the tangent at the point (a, b) to the circle x2+y2=r2 and the coordinate axes, is
Ar42abBr42|ab|Cr4abDr4|ab|The area of the quadrilateral formed by the tangents from the point (4,5) to the circle x2+y2−4x−2y−11=0 with the pair of radii through the points of contact of the tangents is:
A4 sq. unitsB8 sq.unitsC6 sq. unitsDnone
Find the area of the triangle formed by the lines y=x,y=2x,y=3x+4
View SolutionThe area of the triangle formed by the tangent drawn at the point (-12, 5) on the circle x2+y2=169 with the coordinate axes is:
View SolutionThe area of the △ formed by the tangent at (3,4) to the circle x2+y2=25 and the co-ordinate axes is ……………
View SolutionThe area of triangle formed by the tangents from the point (3, 2) to the hyperbola x2−9y2=9 and the chord of contact w.r.t. the point (3, 2) is_____________
View SolutionThe point of contact of a tangent from the point (1,2) to the circle x2+y2=1 has the coordinates :
View Solution