Find the equation of the circle which touches both the axes and the line x=c.
The correct Answer is:(x−1)2+(y±1)2=1
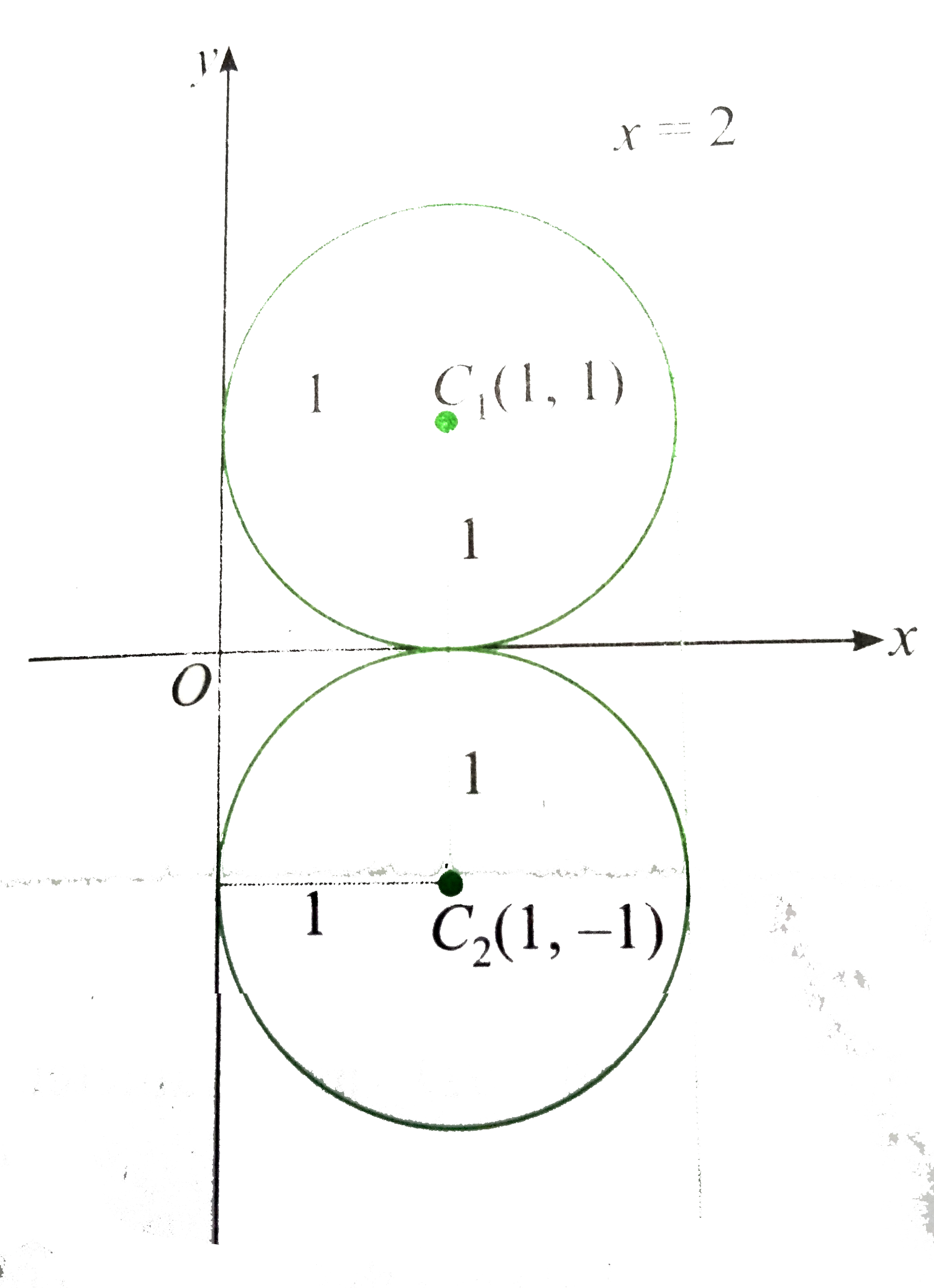
Clearly, radius of the circle is '1' and centre can be (1,±1)
Therefore, equation of circle will be (x−1)2+(y±1)2=1.
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.4
4 Videos
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.5
5 Videos
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.2
2 Videos
- BINOMIAL THEOREMBOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix
4 Videos
- CIRCLESBOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
Similar Questions
Knowledge Check
The equation of a circle which touches both the coordinate axes and the line x = 3 is
A4x2+4y2−12x±12y+9=0B4x2+4y2+12x±12y+9=0C4x2+4y2±12x−12y+9=0D4x2+4y2±12x+12y+9=0
Find the equation of the circle which touches both the axes and the straight line 4x+3y=6 in the first quadrant and lies below it.
View SolutionThe equation of the circle which touches both the axes and the line 4x+3y=6 in first quadrant and lies below it
View SolutionFind the equation of a circle which touches the coordinate axes and the line x+y=1 and has its centre situated remote from the origin with respect to the given line.
View Solution(i) Find the equation of a circle which touches both the axes and whose centre is (2,2).
(ii) Find the equation of a circle, touching the lines x=0, y=0 and x=6.
View SolutionFind the equation of a circle which touches both the axes and whose radius is 2 units
View SolutionEquation of circles which touch both the axes and also the line x=k(k>0) is
View SolutionFind the equation of the circle which touches the coordinate axes and whose centre lies on the line x−2y=3.
View Solution
