Find the equation of the circle which touches both the axes and the straight line 4x+3y=6 in the first quadrant and lies below it.
Video Solution
Text Solution
Since the circle touches both the aces, its equation takes the form.
(x−a)2+(y−a)2=a2
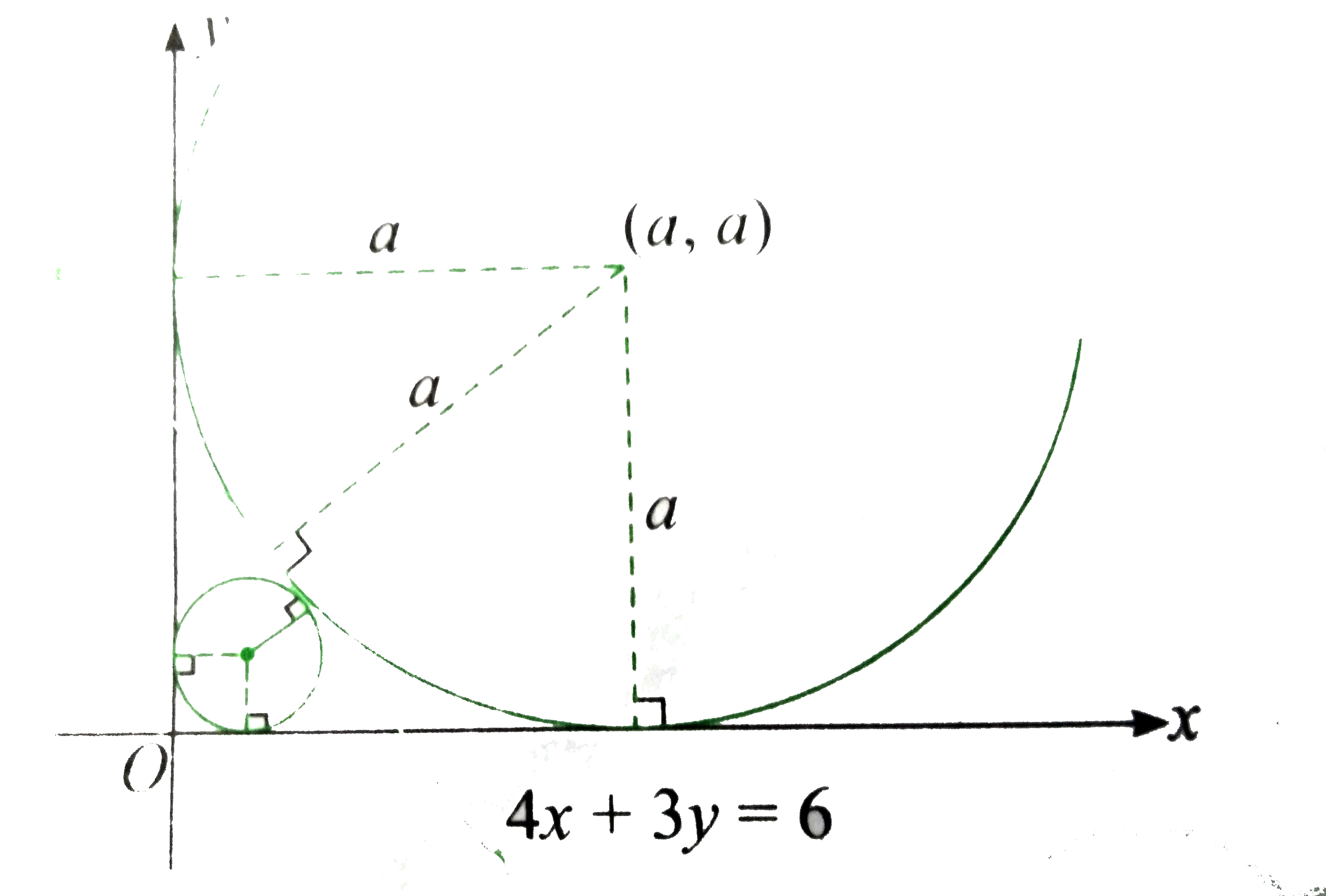
Also, circle touches the line 4x+3y−6=0
So, the distance of centre of the circle from the line is equatl to its radius.
∴|4a+3a−6|√16+9=a
⇒7a−6=±5a
⇒a=3 or 1/2
Therefore, possible equatiosn of circle are
(x−1/2)2+(y−1/2)2=1/4
and (x−3)2+(y−3)2=9
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The equations of the circles which touch both the axes and the line x = a are
Ax2+y2±ax±ay+a24=0Bx2+y2+ax±ay+a24=0Cx2+y2−ax±ay+a24=0Dnone of theseThe equation of the circles which touch both the axes and the line 4x+3y=12 and have centres in the first quadrant, are
Ax2+y2−x−y+1=0Bx2+y2−2x−2y+1=0Cx2+y2−12x−12y+36=0Dx2+y2−6x−6y+36=0Find the equation of a circle which touches both the axes and the line 3 4 8 0 x y - + = and lies in the third quadrant.
Ax2+y2+4x+4y−4=0Bx2+y2−4x−4y+4=0Cx2+y2+4x+4y+4=0DNone of the above
Find the equation of the circle which touches both the axes and the line 3x−4y+8=0 and lies in the third quadrant.
View SolutionEquation of circles which touch both the axes and also the line x=k(k>0) is
View SolutionFind the equation of the circle which touches the coordinate axes and whose centre lies on the line x−2y=3.
View SolutionWhat is the equation to circle which touchs both the axes and has centre on the line x+y=4 ?
View SolutionWhat is the equation to circle which touchs both the axes and has centre on the line x+y=4 ?
View Solution
