Find the mean wage from the following data: Wage (in Rs.: 800820 860900920 9801000 No. of workers:7 14 19 25 20 10 5 By short cut method
To find the mean wage from the given data using the shortcut method, we can follow these steps:
Step 1: Organize the Data
We will create a table to organize the wages (xi) and the number of workers (fi).
| Wage (xi) | Number of Workers (fi) |
|-----------|------------------------|
| 800 | 7 |
| 820 | 14 |
| 860 | 19 |
| 900 | 25 |
| 920 | 20 |
| 980 | 10 |
| 1000 | 5 |
Step 2: Assume a Mean (a)
We will assume a mean (a) from the data. Here, we can choose 900 as the assumed mean.
Step 3: Calculate Deviations (di)
We calculate the deviations (di) from the assumed mean (a = 900):
- For 800: di = 800 - 900 = -100
- For 820: di = 820 - 900 = -80
- For 860: di = 860 - 900 = -40
- For 900: di = 900 - 900 = 0
- For 920: di = 920 - 900 = 20
- For 980: di = 980 - 900 = 80
- For 1000: di = 1000 - 900 = 100
Step 4: Calculate fi * di
Next, we calculate the product of the number of workers (fi) and the deviations (di):
| Wage (xi) | Number of Workers (fi) | Deviations (di) | fi * di |
|-----------|------------------------|------------------|-----------|
| 800 | 7 | -100 | -700 |
| 820 | 14 | -80 | -1120 |
| 860 | 19 | -40 | -760 |
| 900 | 25 | 0 | 0 |
| 920 | 20 | 20 | 400 |
| 980 | 10 | 80 | 800 |
| 1000 | 5 | 100 | 500 |
Step 5: Sum of fi and Sum of fi * di
Now, we calculate the sum of fi and the sum of fi * di:
- Sum of fi (n) = 7 + 14 + 19 + 25 + 20 + 10 + 5 = 100
- Sum of fi * di = -700 - 1120 - 760 + 0 + 400 + 800 + 500 = -880
Step 6: Calculate the Mean Wage (x̄)
Now we can use the formula for the mean wage:
¯x=a+∑fi⋅din
Substituting the values:
¯x=900+−880100
¯x=900−8.8=891.2
Final Answer
The mean wage is Rs. 891.2.
---
We will create a table to organize the wages (xi) and the number of workers (fi).
|-----------|------------------------|
| 800 | 7 |
| 820 | 14 |
| 860 | 19 |
| 900 | 25 |
| 920 | 20 |
| 980 | 10 |
| 1000 | 5 |
We will assume a mean (a) from the data. Here, we can choose 900 as the assumed mean.
We calculate the deviations (di) from the assumed mean (a = 900):
- For 820: di = 820 - 900 = -80
- For 860: di = 860 - 900 = -40
- For 900: di = 900 - 900 = 0
- For 920: di = 920 - 900 = 20
- For 980: di = 980 - 900 = 80
- For 1000: di = 1000 - 900 = 100
Next, we calculate the product of the number of workers (fi) and the deviations (di):
|-----------|------------------------|------------------|-----------|
| 800 | 7 | -100 | -700 |
| 820 | 14 | -80 | -1120 |
| 860 | 19 | -40 | -760 |
| 900 | 25 | 0 | 0 |
| 920 | 20 | 20 | 400 |
| 980 | 10 | 80 | 800 |
| 1000 | 5 | 100 | 500 |
Now, we calculate the sum of fi and the sum of fi * di:
- Sum of fi * di = -700 - 1120 - 760 + 0 + 400 + 800 + 500 = -880
Now we can use the formula for the mean wage:
The mean wage is Rs. 891.2.
Topper's Solved these Questions
Similar Questions
Find the mean wage from the data given below: Wage (in Rs) 800 820 860 900 920 980 1000 No. of workers: 7 14 19 25 20 10 5
View SolutionFind the mean from the following table using short cut method :
 View Solution
View SolutionFind the mean from the following table using short cut method :
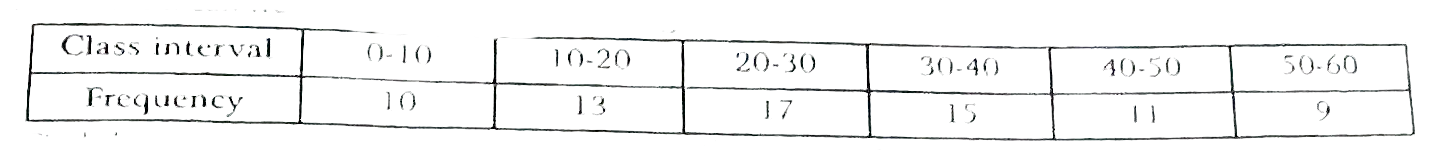 View Solution
View SolutionFind the mean from the following table using short cut method. :
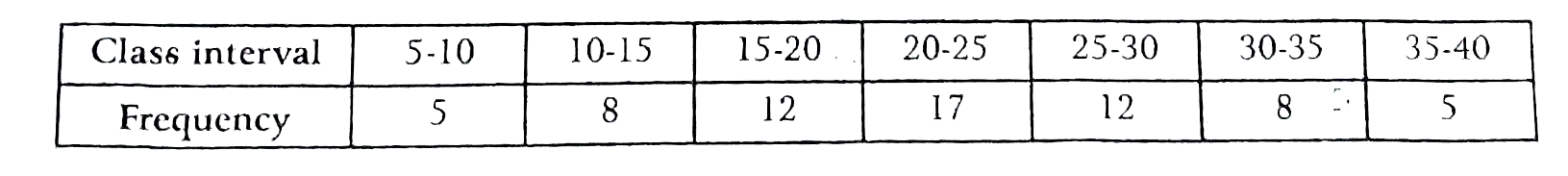 View Solution
View SolutionFind the mean, median and mode of the following data:
19, 7, 7, 25, 7
View SolutionFind mean, median and mode of the following data : 10, 12, 14, 16, 14
View SolutionCalculate the mean deviation from the median of the following data: Wages per week (in Rs.), 10-20, 20-30, 30-40, 40-50, 50-60, 60-70, 70-80 No. of workers, 4, 6, 10, 20, 10, 6, 4
View SolutionCalculate the median deviation from the median of the following data: Wages per week (in Rs.), 10-20, 20-30, 30-40, 40-50, 50-60, 60-70, 70-80 No. of workers, 4, 6, 10, 20, 10, 6, 4
View SolutionCalculate the mean and standard deviation for the following data: Wages upto (into Rs.) 15 30 45 60 75 90 105 120 No. of workers 12 30 65 107 157 202 222 230
View SolutionFind the mean deviation from the median of the following data: 15 21 27 30 3 5 6 7
View Solution

