Find the missing frequencies in the following frequency distribution if it is known that the mean of the distribution is 50. f=17,f1=32,f2=19 Total 120
To find the missing frequencies f1 and f2 in the given frequency distribution, we can follow these steps:
Step 1: Set up the equation for total frequency
We know that the total frequency is 120. The frequencies given are:
- f=17
- f1=?
- f2=?
- 32
- 19
We can write the equation for total frequency:
17+f1+32+f2+19=120
Step 2: Simplify the equation
Now, we simplify the equation:
17+32+19=68
So, we have:
f1+f2+68=120
Subtracting 68 from both sides gives:
f1+f2=120−68=52
This is our first equation (Equation 1).
Step 3: Set up the equation for the mean
We know that the mean of the distribution is 50. The formula for the mean is:
Mean=ΣfixiΣfi
Here, Σfi=120, and we need to calculate Σfixi.
Step 4: Calculate Σfixi
We will calculate Σfixi using the given values:
- For x=10, frequency f=17: 17×10=170
- For x=30, frequency f1: 30×f1
- For x=50, frequency f=32: 32×50=1600
- For x=70, frequency f2: 70×f2
- For x=90, frequency f=19: 19×90=1710
Putting this together:
Σfixi=170+30f1+1600+70f2+1710
So,
Σfixi=3480+30f1+70f2
Step 5: Set up the equation for the mean
Since the mean is given as 50, we can set up the equation:
50=3480+30f1+70f2120
Multiplying both sides by 120 gives:
6000=3480+30f1+70f2
Subtracting 3480 from both sides gives:
30f1+70f2=6000−3480=2520
This is our second equation (Equation 2).
Step 6: Solve the system of equations
We now have two equations:
1. f1+f2=52 (Equation 1)
2. 30f1+70f2=2520 (Equation 2)
From Equation 1, we can express f2 in terms of f1:
f2=52−f1
Step 7: Substitute f2 into Equation 2
Substituting f2 into Equation 2:
30f1+70(52−f1)=2520
Expanding this gives:
30f1+3640−70f1=2520
Combining like terms:
−40f1+3640=2520
Subtracting 3640 from both sides:
−40f1=2520−3640=−1120
Dividing by -40 gives:
f1=112040=28
Step 8: Find f2
Now substituting f1 back into Equation 1 to find f2:
f2=52−28=24
Final Answer
Thus, the missing frequencies are:
- f1=28
- f2=24
We know that the total frequency is 120. The frequencies given are:
- f=17
- f1=?
- f2=?
- 32
- 19
17+f1+32+f2+19=120
Now, we simplify the equation:
17+32+19=68
So, we have:
f1+f2+68=120
Subtracting 68 from both sides gives:
f1+f2=120−68=52
This is our first equation (Equation 1).
We know that the mean of the distribution is 50. The formula for the mean is:
Mean=ΣfixiΣfi
Here, Σfi=120, and we need to calculate Σfixi.
We will calculate Σfixi using the given values:
- For x=10, frequency f=17: 17×10=170
- For x=30, frequency f1: 30×f1
- For x=50, frequency f=32: 32×50=1600
- For x=70, frequency f2: 70×f2
- For x=90, frequency f=19: 19×90=1710
Σfixi=170+30f1+1600+70f2+1710
So,
Σfixi=3480+30f1+70f2
Since the mean is given as 50, we can set up the equation:
50=3480+30f1+70f2120
Multiplying both sides by 120 gives:
6000=3480+30f1+70f2
Subtracting 3480 from both sides gives:
30f1+70f2=6000−3480=2520
This is our second equation (Equation 2).
We now have two equations:
1. f1+f2=52 (Equation 1)
2. 30f1+70f2=2520 (Equation 2)
f2=52−f1
Substituting f2 into Equation 2:
30f1+70(52−f1)=2520
Expanding this gives:
30f1+3640−70f1=2520
Combining like terms:
−40f1+3640=2520
Subtracting 3640 from both sides:
−40f1=2520−3640=−1120
Dividing by -40 gives:
f1=112040=28
Now substituting f1 back into Equation 1 to find f2:
f2=52−28=24
Thus, the missing frequencies are:
- f1=28
- f2=24
Topper's Solved these Questions
Similar Questions
Find the frequencies in the following frequency distribution if it is known that the mean of the distribution is 1.46.
View SolutionFind the missing frequencies in the following frequency distribution if it is known that the mean of the distribution is 50. x : 10 30 50 70 90 f : 17 f1 32 f2 19 Total 120.
View Solution
Knowledge Check
Find the missing frequency in the following distribution, if it is known that the mean of the distribution is 50.16
 Af1= 27Bf1= 23Cf1 = 34Df1 = 30
Af1= 27Bf1= 23Cf1 = 34Df1 = 30
Find the missing frequencies in the following frequency distribution whose mean is 34.
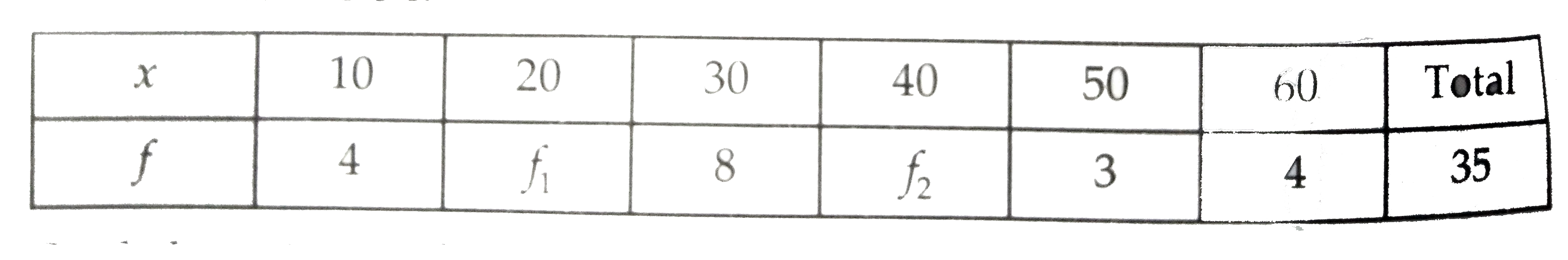
View SolutionFind the missing frequencies in the following frequency distribution if it is known that the mean of the distribution is 1.46. N⊙ofant(x) 0 1 2 3 4 5 TotalFrequency(f): 46 ? ? 25 10 5 200
View SolutionConsider the following frequency distribution
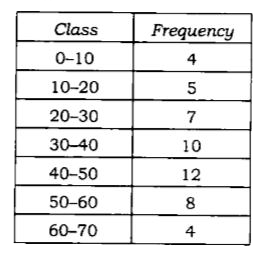
What is the mean of the distribution?
View SolutionThe mean of the following frequency distribution is 50 :
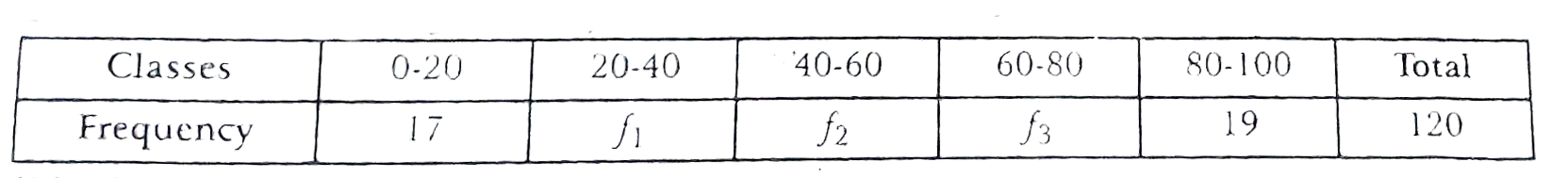
View SolutionFind the mean of the followings frequency distribution :
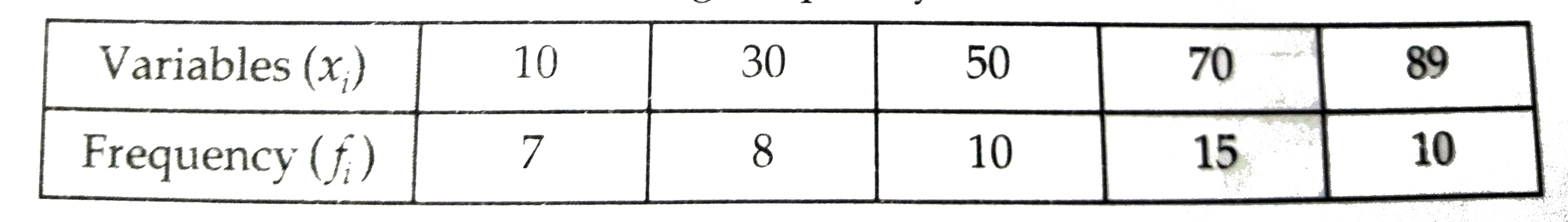
View SolutionFind the mean for the following frequency distribution :
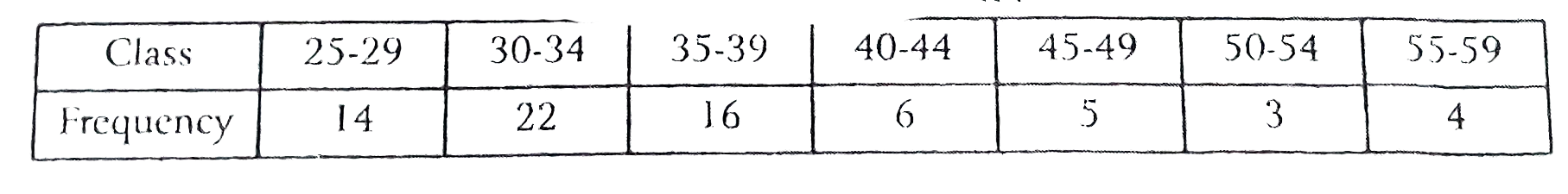
View SolutionFind the mean and variance for the following frequency distributions in
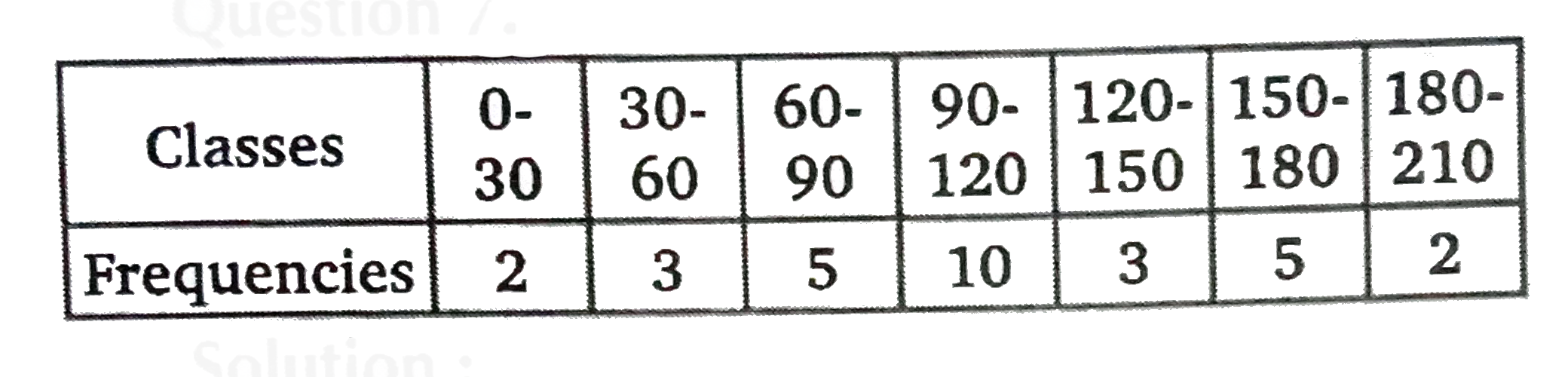
View Solution