Find the set of real value(s) of $a$ for which the equation $| 2 x + 3 | + 2 x - 3 ∣ = a x + 6$ has more than two solutions.

Video Solution

Text Solution

Verified by Experts

We have $y = | 2 x + 3 | + | 2 x - 3 |$
$= {\begin{matrix} 4 x, & x \geq \frac{3}{2} \\ 6, & - \frac{3}{2} < x < \frac{3}{2} \\ - 4 x, & x \leq - \frac{3}{2} \end{matrix}$
The graph of the function is as shown in the following figure.

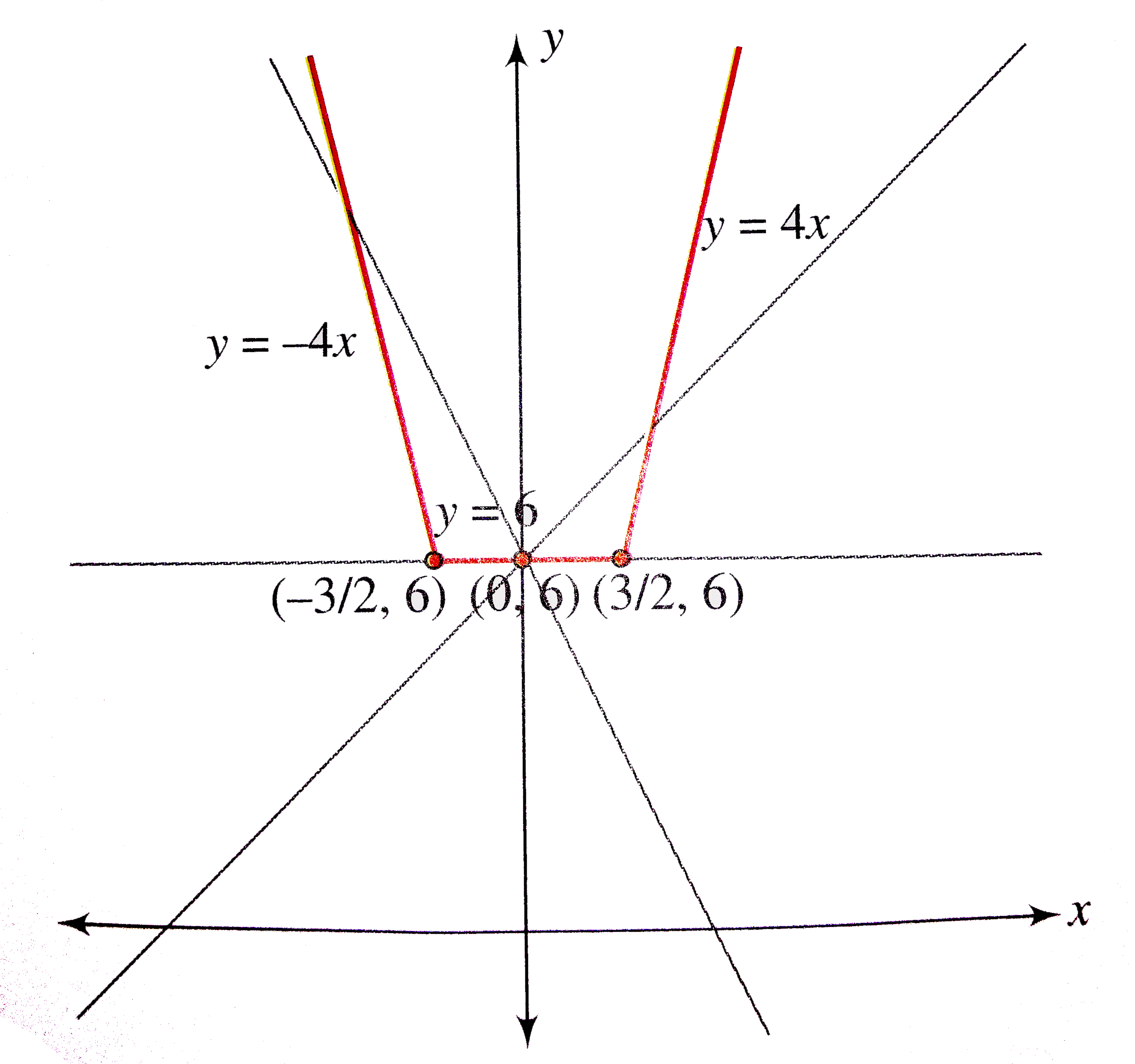
$y = a x + 6$ is a line passing through $(0, 6)$ .
From the graph, it is obvious that if $a = 0$ , we have infinite solutions in $[- \frac{3}{2}, \frac{3}{2}]$ .
If $0 < a < 4 or - 4 < a < 0$ , two solutions.
If $a = 4 or - 4$ , we have $x = 0$ as the only solution.

Updated on:21/07/2023