Find the standard deviation for the following data: (i) x: , 3, 8, 13, 18, 23 f: , 7, 10, 15, 10, 6
To find the standard deviation for the given data, we will follow these steps:
Step 1: Prepare the Data Table
We will create a table to organize the data and calculate the necessary components for the standard deviation.
| x | f | f⋅x | x−¯x | (x−¯x)2 | f⋅(x−¯x)2 |
|---------|---------|------------------|--------------------|------------------------|-------------------------------|
| 3 | 7 | 21 | | | |
| 8 | 10 | 80 | | | |
| 13 | 15 | 195 | | | |
| 18 | 10 | 180 | | | |
| 23 | 6 | 138 | | | |
| Total | 50 | 660 | | | |
Step 2: Calculate ¯x (Mean)
The mean ¯x is calculated using the formula:
¯x=Σ(f⋅x)Σf
Substituting the values:
¯x=66050=13.2
Step 3: Calculate x−¯x
Now, we will calculate x−¯x for each x:
- For x=3: 3−13.2=−10.2
- For x=8: 8−13.2=−5.2
- For x=13: 13−13.2=−0.2
- For x=18: 18−13.2=4.8
- For x=23: 23−13.2=9.8
Step 4: Calculate (x−¯x)2
Next, we will square the results from the previous step:
- For −10.2: (−10.2)2=104.04
- For −5.2: (−5.2)2=27.04
- For −0.2: (−0.2)2=0.04
- For 4.8: (4.8)2=23.04
- For 9.8: (9.8)2=96.04
Step 5: Calculate f⋅(x−¯x)2
Now, we will multiply f with (x−¯x)2:
| x | f | (x−¯x)2 | f⋅(x−¯x)2 |
|---------|---------|------------------------|-------------------------------|
| 3 | 7 | 104.04 | 7⋅104.04=728.28 |
| 8 | 10 | 27.04 | 10⋅27.04=270.4 |
| 13 | 15 | 0.04 | 15⋅0.04=0.6 |
| 18 | 10 | 23.04 | 10⋅23.04=230.4 |
| 23 | 6 | 96.04 | 6⋅96.04=576.24 |
| Total | 50 | | 1998.88 |
Step 6: Calculate Variance
The variance σ2 is given by the formula:
σ2=Σ(f⋅(x−¯x)2)Σf
Substituting the values:
σ2=1998.8850=39.9776
Step 7: Calculate Standard Deviation
Finally, the standard deviation σ is the square root of the variance:
σ=√39.9776≈6.32
Final Answer
The standard deviation for the given data is approximately 6.32.
We will create a table to organize the data and calculate the necessary components for the standard deviation.
|---------|---------|------------------|--------------------|------------------------|-------------------------------|
| 3 | 7 | 21 | | | |
| 8 | 10 | 80 | | | |
| 13 | 15 | 195 | | | |
| 18 | 10 | 180 | | | |
| 23 | 6 | 138 | | | |
| Total | 50 | 660 | | | |
The mean ¯x is calculated using the formula:
Now, we will calculate x−¯x for each x:
- For x=8: 8−13.2=−5.2
- For x=13: 13−13.2=−0.2
- For x=18: 18−13.2=4.8
- For x=23: 23−13.2=9.8
Next, we will square the results from the previous step:
- For −5.2: (−5.2)2=27.04
- For −0.2: (−0.2)2=0.04
- For 4.8: (4.8)2=23.04
- For 9.8: (9.8)2=96.04
Now, we will multiply f with (x−¯x)2:
|---------|---------|------------------------|-------------------------------|
| 3 | 7 | 104.04 | 7⋅104.04=728.28 |
| 8 | 10 | 27.04 | 10⋅27.04=270.4 |
| 13 | 15 | 0.04 | 15⋅0.04=0.6 |
| 18 | 10 | 23.04 | 10⋅23.04=230.4 |
| 23 | 6 | 96.04 | 6⋅96.04=576.24 |
| Total | 50 | | 1998.88 |
The variance σ2 is given by the formula:
Finally, the standard deviation σ is the square root of the variance:
The standard deviation for the given data is approximately 6.32.
Topper's Solved these Questions
Similar Questions
Find the standard deviation from the following data:
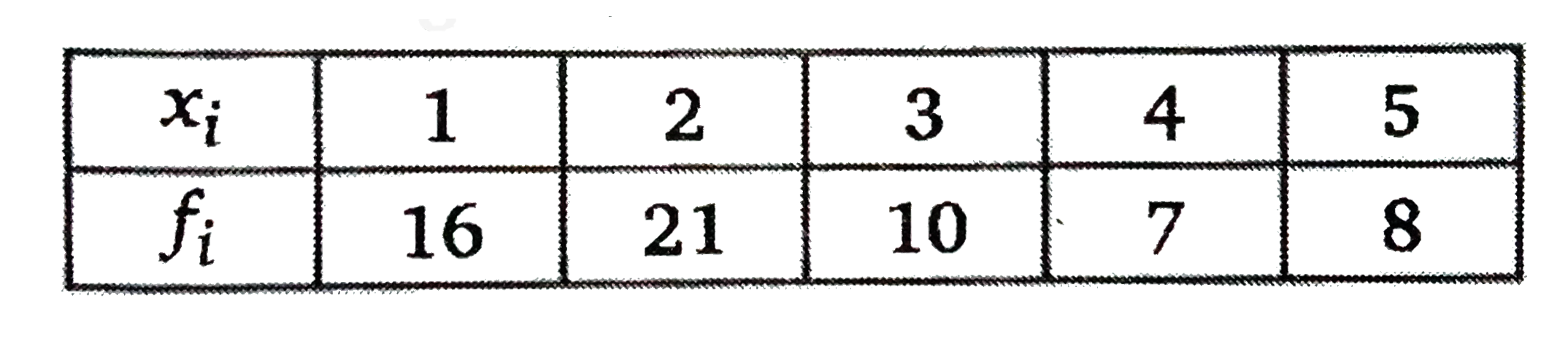 View Solution
View SolutionFind the standard deviation from the following datas:
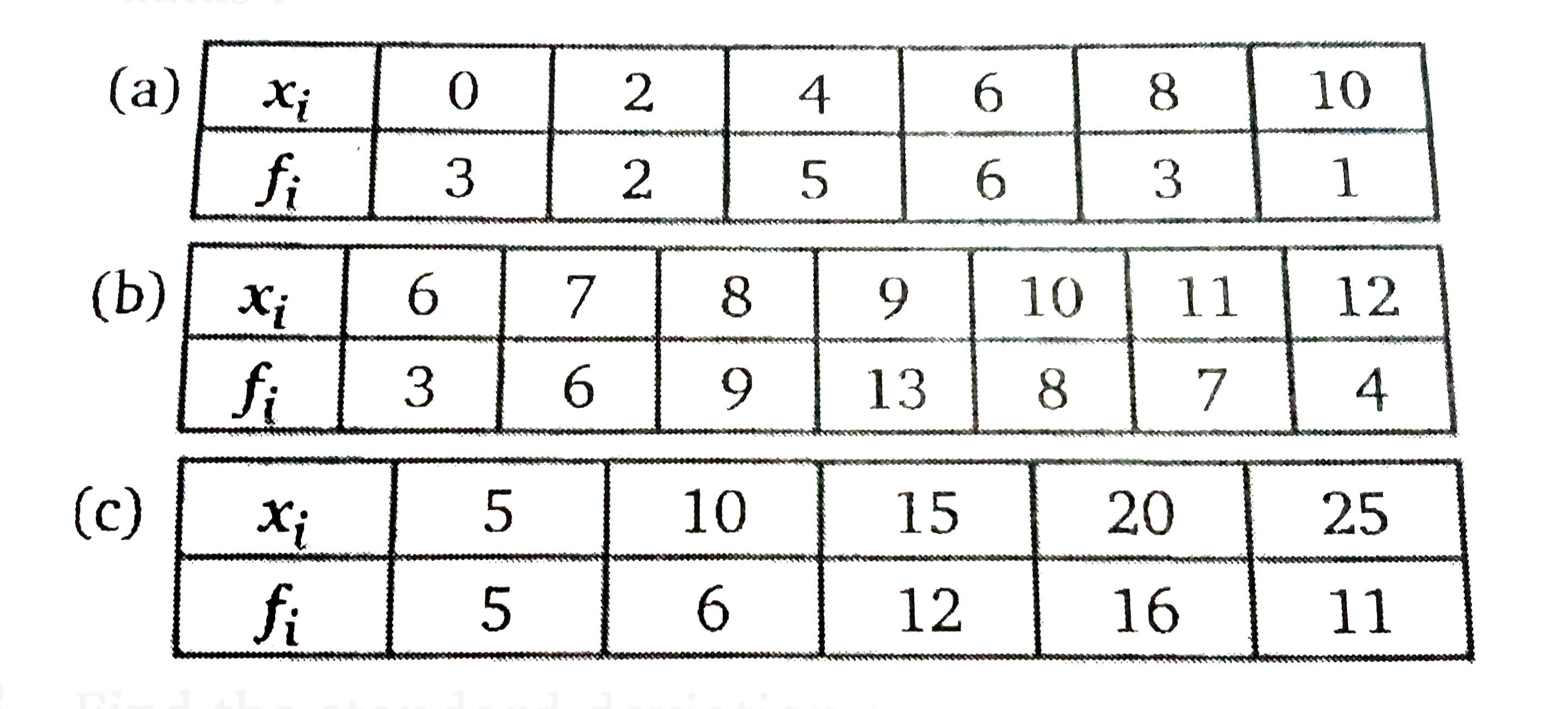 View Solution
View SolutionFind the mean and standard deviation from the following data :
 View Solution
View SolutionFind the standard deviation for the following data :5,9,8,12,6,10,6,8
View SolutionFind the variance and standard deviation of the following data:
 View Solution
View SolutionFind the variance and standard deviation from the following data:
 View Solution
View SolutionFind the variance and standard deviation for the following data: 4,6,10,12,14,18,20
View SolutionFind the variance and standard deviation of the following data 5,12,3,18,6,8,2,10
View SolutionFind the mean variance and standard deviation for the following data: 2,4,5,6,8,17
View SolutionFind the mean, variance and standard deviation for the following data: 6,7,10,12,13,4,8,12
View Solution

