Find the values of α for which the point (α−1,α+1) lies in the larger segment of the circle x2+y2−x−y−6=0 made by the chord whose equation is x+y−2=0
The given circle
S(x,y)≡x2+y2−x−y−6=0 (1) has center at C≡(1/2,1/2),
According to the required conditions, the given point P(α−1,α+1) must lie inside the given circle, i.e.,
S(α−1,α+1)<0
or (α−1)2+(α+1)2−(α−11)−(α+1)−6<0
or α2−α−2<0
i.e., (α−2)(α+1)<0
or −1<α<2 (2)
Also, P and C must lie on the same side of the line
L(x,y)≡x+y−2=0 (3)
i.e., L(1/2,1/2) and L(α−1,α+1) must have the same sign.
Now, since
L(12,12)=12+12−2<0
we have L(α−1,α+1)=(α−1)+(α+1)−2<0
i.e., α<1 (4)
Inequalities (2) and (4) together give the possible values of α as −1<α<1
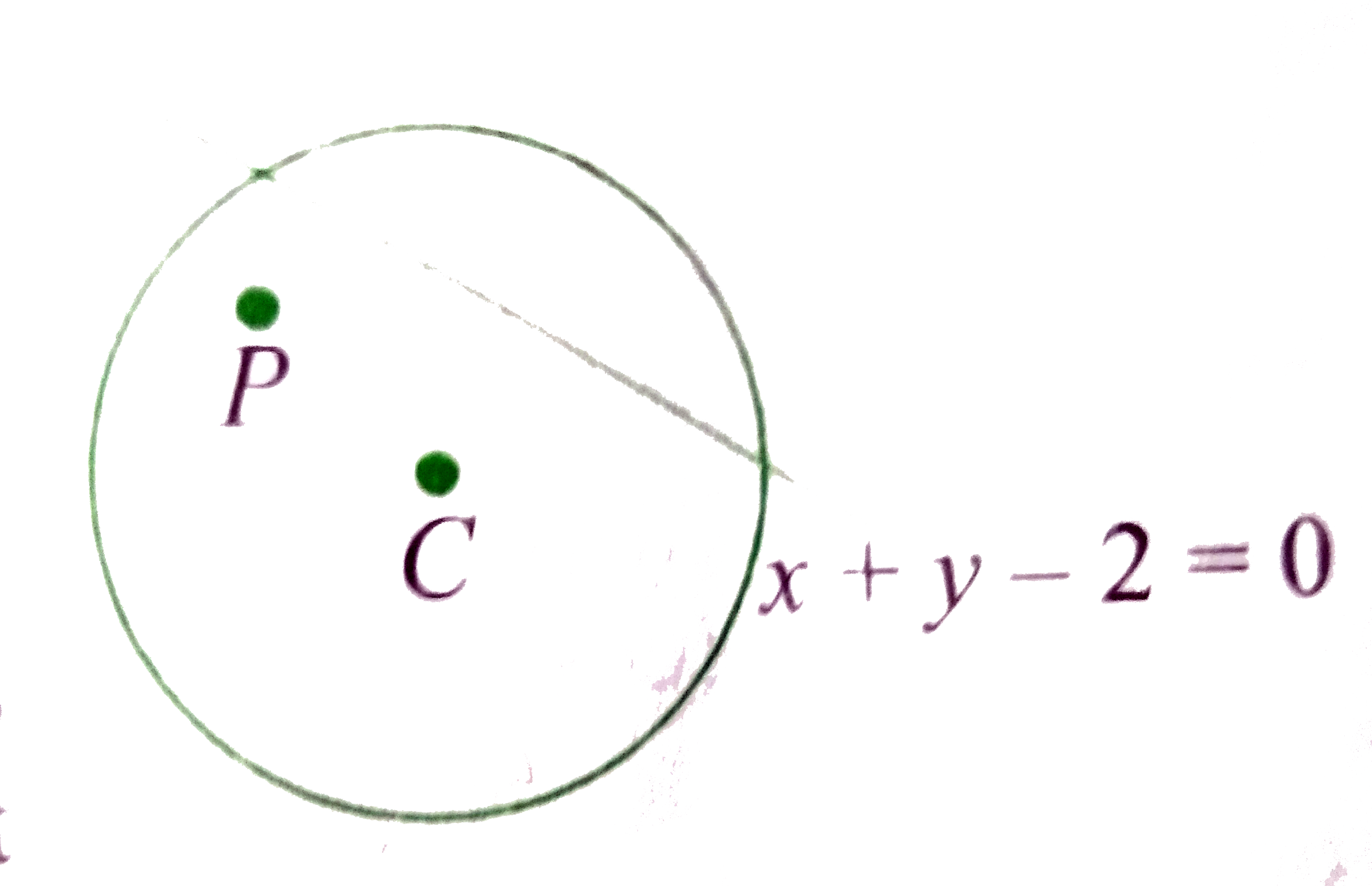
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The range of 'α' for which the point (α,α) lies in minor arc bounded by the curves x2+y2=1 and x+y=1 is
A12<α1√2B−1√2<α<1√2Cα>12D0<α<12 - Question 2 - Select One
Set of value of α for which the point (α,1) lies inside the circle x2+y2−4=0 and parabola y2=4x is
A|α|<√3B|α|<2C14<α<√3Dnone of these
The set of values of a for which the point (2a,a+1) is an interior point of the larger segment of the circle x2+y2−2x−2y−8=0 made by the chord x−y+1=0, is
View SolutionThe value of α for which the point (α,α+2) is an interior point of smaller segment of the curve x2+y2−42=0 made by the chord of the curve whose equation is 3x+4y+12=0 is:
View SolutionDetermine all the values of α for which the point (α,α2) lies inside the triangle formed by the lines. 2x+3y−1=0 x+2y−3=0 5x−6y−1=0
View SolutionFind the values of α so that the point P(α2,α) lies inside or on the triangle formed by the lines x−5y+6=0,x−3+2=0andx−2y−3=0.
View SolutionFind the values of a for which the point (a,a),a>0, lies outside the circle x2+y2−2x+6y−6=0
View SolutionFind the interval of α for which (α,α2,α)and(3,2,1) lies on same side of x+y−4z+2=0.
View Solution

