For the circle x2+y2=r2, find the value of r for which the area enclosed by the tangents drawn from the point P(6,8) to the circle and the chord of contact and the chord of contact is maximum.
The given circle is x2+y2=r2. From point (6,8), tangents are drawn to this circle.
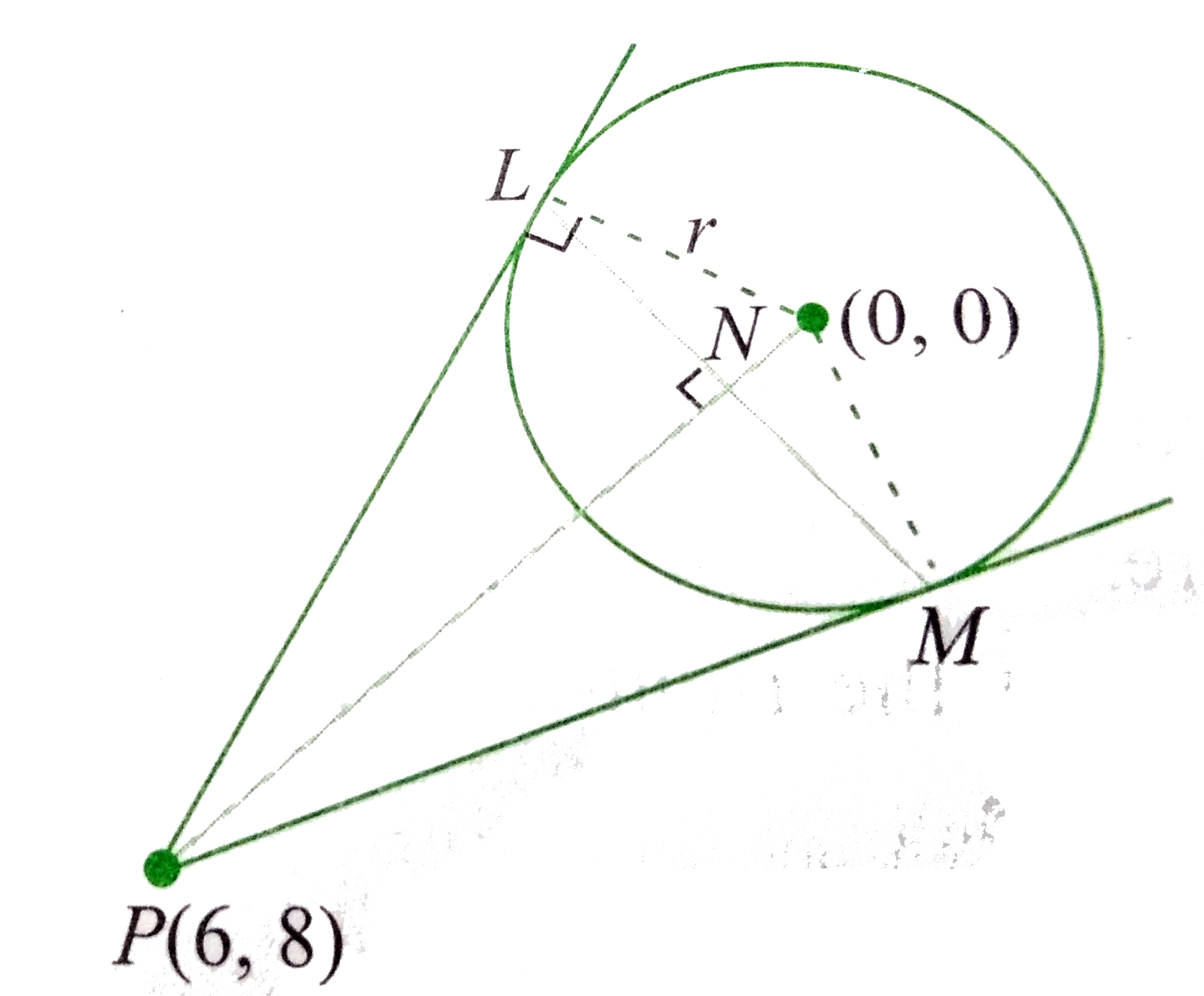
Then, the length of tangent is
PL=√62+82−r2=√100−r2
Also, the equation of chord of contact LM is 6x+8y−r2=0.
PN= Length of ⊥ from P to LM
=36+64−r2√36+64=100−r210
Now, in right - angles ΔPLN
LN2=PL2−PN2
=(100−r2)−(100−r2)2100=(100−r2)r2100
or LN=r√100−r210
∴LM=r√100−r25 (∵LM=2LN)
∴ Area of ΔPLM=Δ=12×LM×PN
=12×r√100−r25×100−r210
=1100[r(100−r2)3/2]
For the maximum value of Δ, we should have
dΔdr=0
or 1100[(100−r2)3/2+r32(100−r2)1/2(−2r)]=0
or (100−r2)1/2[100−r2−3r2]=0
i.e., r=10 or r=5
But r=10 gives the length of tangent PL. Therefore, r cancel (=)10.
Hence, r=5.
Topper's Solved these Questions
Similar Questions
Find the area of the triangle formed by the tangents drawn from the point (4,6) to the circle x2+y2=25 and their chord of contact.
Answer: 405√352 sq. units
View SolutionTangents are drawn from the point (-1, 2) to the parabola y2=4x The area of the triangle for tangents and their chord of contact is
View Solution
Knowledge Check
Statement-1: The line x+9y−12=0 is the chord of contact of tangents drawn from a point P to the circle 2x2+2y2−3x+5y−7=0.
Statement-2: The line segment joining the points of contacts of the tangents drawn from an external point P to a circle is the chord of contact of tangents drawn from P with respect to the given circleAStatement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1.BStatement-1 is True, Statement-2 is not a correct explanation for Statement-1.CStatement-1 is True, Statement-2 is False.DStatement-1 is False, Statement-2 is True.Pair of tangents are drawn from origin to the circle x2+y2–8x–4y+16=0 then square of length of chord of contact is
A645B245C85D813
The length of the chord of contact of the tangents drawn from the point (−2,3) to the circle x2+y2−4x−6y+12=0 is:
View SolutionTangents are drawn to the parabola y2=4x from the point (1,3). The length of chord of contact is
View SolutionAngle between tangents drawn from a point P to circle x2+y2−48−8y+8=0 is 60∘ then length of chord of contact of P is
View SolutionTangents are drawn from external poinl P(6,8) to the circle x2+y2=r2 find the radius r of the circle such that area of triangle formed by the tangents and chord of contact is maximum is (A) 25 (B) 15 (C) 5 (D) none
View SolutionFind the equation to the chord of contact of the tangents drawn from an external point (−3,2) to the circle x2+y2+2x−3=0.
View SolutionConsider a circle x2+y2=4 and a point P(4,2). θ denotes the angle enclosed by the tangents from P on the circle and A, B are the points of contact of the tangents from P on the circle. The value of θ lies in the interval The intercept made by a tangent on the x-axis is
View Solution
