Four equal circles are described about the four corners of a square so that each touches two of the others as shown in Fig. 15.38. Find the area of the shaded region, each side of the square measuring 14 cm. (FIGURE)
To solve the problem of finding the area of the shaded region when four equal circles are inscribed in the corners of a square, follow these steps:
Step 1: Understand the Dimensions
The side length of the square is given as 14 cm.
Step 2: Calculate the Radius of Each Circle
Since each circle is inscribed in the corners of the square, the radius r of each circle is half of the side length of the square. Therefore,
r=142=7 cm
Step 3: Calculate the Area of the Square
The area A of the square can be calculated using the formula:
A=side2=14×14=196 cm2
Step 4: Calculate the Area of One Circle
The area of a full circle is given by the formula:
Area of Circle=πr2
However, since we are dealing with quadrants (one-fourth of a circle) at each corner, we need to find the area of one quadrant:
Area of Quadrant=14πr2=14×π×(7)2
Using π≈227:
Area of Quadrant=14×227×49=22×4928=107828≈38.5 cm2
Step 5: Calculate the Total Area of the Four Quadrants
Since there are four quadrants (one at each corner of the square):
Total Area of Four Quadrants=4×38.5≈154 cm2
Step 6: Calculate the Area of the Shaded Region
The area of the shaded region can be found by subtracting the total area of the four quadrants from the area of the square:
Area of Shaded Region=Area of Square−Total Area of Four Quadrants
Area of Shaded Region=196−154=42 cm2
Final Answer
The area of the shaded region is 42 cm2.
---
The side length of the square is given as 14 cm.
Since each circle is inscribed in the corners of the square, the radius r of each circle is half of the side length of the square. Therefore,
r=142=7 cm
The area A of the square can be calculated using the formula:
A=side2=14×14=196 cm2
The area of a full circle is given by the formula:
Area of Circle=πr2
However, since we are dealing with quadrants (one-fourth of a circle) at each corner, we need to find the area of one quadrant:
Area of Quadrant=14πr2=14×π×(7)2
Using π≈227:
Area of Quadrant=14×227×49=22×4928=107828≈38.5 cm2
Since there are four quadrants (one at each corner of the square):
Total Area of Four Quadrants=4×38.5≈154 cm2
The area of the shaded region can be found by subtracting the total area of the four quadrants from the area of the square:
Area of Shaded Region=Area of Square−Total Area of Four Quadrants
Area of Shaded Region=196−154=42 cm2
The area of the shaded region is 42 cm2.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Four equal charges Q are placed at the four corners of a square of each side is 'a'. Work done in removing a charge −Q from its centre to infinity is
AzeroB√2q2πε0aC√2qπε0aDq2πε0a - Question 2 - Select One
A and B are centers of two circles that touch each other externally, as shown in the figure.What is the area of the circle whose diameter is AB?
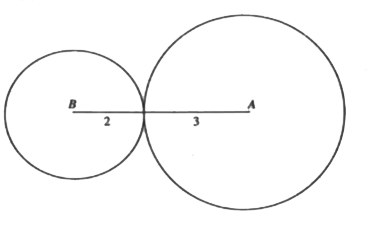 A4πB25π/4C9πD16π
A4πB25π/4C9πD16π
In Figure, PQRS is a square of side 4 cm. Find the area of the shaded region.
View SolutionFour equal circles, each of radius 5 cm, touch each other as shown in Fig.20. Find the area included between them. (Takeπ=3.14).
View SolutionFour equal circles, each of radius 5cm, touch each other as shown in Figure. Find the area included between them.
View SolutionIn figure, a square of diagonal 8 cm is inscribed in a circle. Find the area of the shaded region.
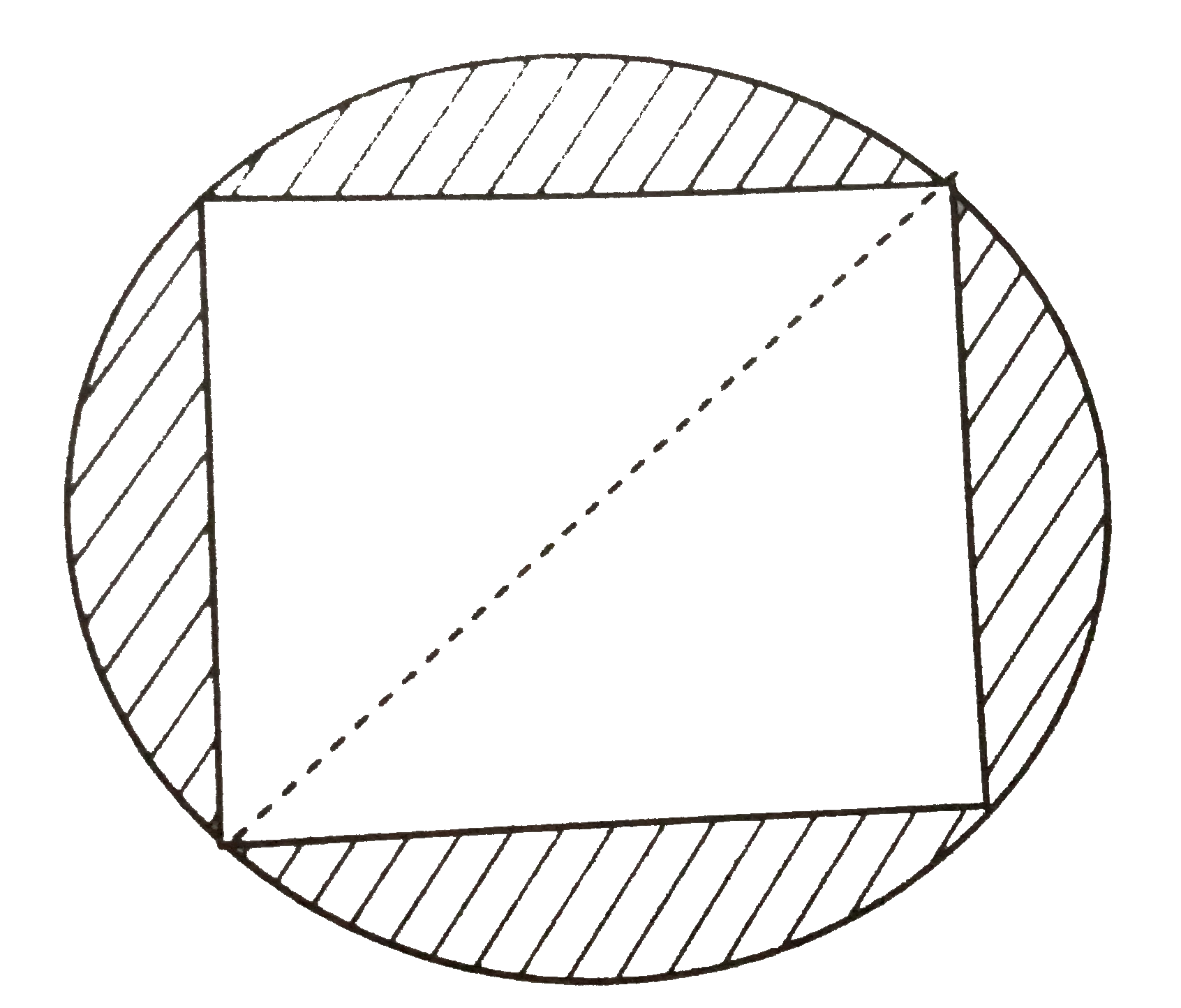 View Solution
View SolutionFour equal charges are kept fixed on the vertices of a square of side 'a'. A charge q1 is kept at the centre of the square as shown in the figure . Then
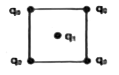 View Solution
View SolutionIn figure arcs have been drawn with radii 14 cm each and with centres P, Q and R. Find the area of the shaded region .
 View Solution
View Solution

