Doubtnut Promotions Sticky

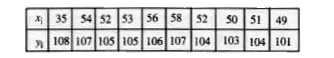
From the prices of shares X and Y below, find out which is more stable in value X 35 54 52 53 56 58 52 50 51 49 . Y 108 107 105 105 106 107 104 103 104 101

Video Solution
Doubtnut Promotions Banner
Text Solution
Generated By DoubtnutGPT

To determine which share, X or Y, is more stable in value, we will calculate the mean and standard deviation for both sets of share prices. The share with the lower standard deviation is considered more stable.

Step 1: Calculate the Mean of Share X

The prices of share X are:
35,54,52,53,56,58,52,50,51,49

- Total of prices (X):
35+54+52+53+56+58+52+50+51+49=500

- Number of observations (n):
n=10

- Mean (X̄):
Mean=Totaln=50010=50

Step 2: Calculate the Variance of Share X

To calculate the variance, we need to find the squared differences from the mean.

- Squared differences:
(35−51)2=256(54−51)2=9(52−51)2=1(53−51)2=4(56−51)2=25(58−51)2=49(52−51)2=1(50−51)2=1(51−51)2=0(49−51)2=4

- Total of squared differences:
256+9+1+4+25+49+1+1+0+4=350

- Variance (σ²):
Variance=Total of squared differencesn=35010=35

Step 3: Calculate the Standard Deviation of Share X

- Standard Deviation (σ):
Standard Deviation=√Variance=√35≈5.92

Step 4: Calculate the Mean of Share Y

The prices of share Y are:
108,107,105,105,106,107,104,103,104,101

- Total of prices (Y):
108+107+105+105+106+107+104+103+104+101=1050

- Mean (Ȳ):
Mean=105010=105

Step 5: Calculate the Variance of Share Y

- Squared differences:
(108−105)2=9(107−105)2=4(105−105)2=0(105−105)2=0(106−105)2=1(107−105)2=4(104−105)2=1(103−105)2=4(104−105)2=1(101−105)2=16

- Total of squared differences:
9+4+0+0+1+4+1+4+1+16=40

- Variance (σ²):
Variance=4010=4

Step 6: Calculate the Standard Deviation of Share Y

- Standard Deviation (σ):
Standard Deviation=√4=2

Conclusion

- Standard Deviation of Share X: ≈5.92
- Standard Deviation of Share Y: 2

Since the standard deviation of share Y is less than that of share X, share Y is more stable in value.

---

|