Calculate the mean deviation from the median of the following frequency distribution:5 7 9 11 13 15 17 2 4 6 8 10 12 8
To calculate the mean deviation from the median of the given frequency distribution, we will follow these steps:
Step 1: Organize the Data
We have the following data points:
- 5, 7, 9, 11, 13, 15, 17, 2, 4, 6, 8, 10, 12, 8
Step 2: Create a Frequency Distribution Table
We will create a frequency distribution table for the data:
| Value (Xi) | Frequency (Fi) |
|------------|----------------|
| 2 | 1 |
| 4 | 1 |
| 5 | 1 |
| 6 | 1 |
| 7 | 1 |
| 8 | 2 |
| 9 | 1 |
| 10 | 1 |
| 11 | 1 |
| 12 | 1 |
| 13 | 1 |
| 15 | 1 |
| 17 | 1 |
Step 3: Calculate Cumulative Frequency
Now, we will calculate the cumulative frequency:
| Value (Xi) | Frequency (Fi) | Cumulative Frequency |
|------------|----------------|----------------------|
| 2 | 1 | 1 |
| 4 | 1 | 2 |
| 5 | 1 | 3 |
| 6 | 1 | 4 |
| 7 | 1 | 5 |
| 8 | 2 | 7 |
| 9 | 1 | 8 |
| 10 | 1 | 9 |
| 11 | 1 | 10 |
| 12 | 1 | 11 |
| 13 | 1 | 12 |
| 15 | 1 | 13 |
| 17 | 1 | 14 |
Step 4: Find the Median
To find the median, we need to find n/2:
- Total frequency n=14
- n/2=14/2=7
The median lies in the cumulative frequency where it exceeds n/2. The cumulative frequency just greater than 7 is 8, which corresponds to the value 9.
Thus, the median a=9.
Step 5: Calculate Mean Deviation from the Median
The formula for mean deviation from the median is:
Mean Deviation=1n∑|Xi−a|⋅Fi
Where |Xi−a| is the absolute deviation from the median.
Now we will calculate |Xi−9| for each value and multiply by the frequency:
| Value (Xi) | Frequency (Fi) | |Xi - 9| | Fi * |Xi - 9| |
|------------|----------------|---------|-----------------|
| 2 | 1 | 7 | 7 |
| 4 | 1 | 5 | 5 |
| 5 | 1 | 4 | 4 |
| 6 | 1 | 3 | 3 |
| 7 | 1 | 2 | 2 |
| 8 | 2 | 1 | 2 |
| 9 | 1 | 0 | 0 |
| 10 | 1 | 1 | 1 |
| 11 | 1 | 2 | 2 |
| 12 | 1 | 3 | 3 |
| 13 | 1 | 4 | 4 |
| 15 | 1 | 6 | 6 |
| 17 | 1 | 8 | 8 |
Now we sum the last column:
∑Fi⋅|Xi−9|=7+5+4+3+2+2+0+1+2+3+4+6+8=43
Step 6: Calculate Mean Deviation
Now we can calculate the mean deviation:
Mean Deviation=1n∑Fi⋅|Xi−9|=4314≈3.07
Final Result
Thus, the mean deviation from the median is approximately 3.07.
---
We have the following data points:
- 5, 7, 9, 11, 13, 15, 17, 2, 4, 6, 8, 10, 12, 8
We will create a frequency distribution table for the data:
|------------|----------------|
| 2 | 1 |
| 4 | 1 |
| 5 | 1 |
| 6 | 1 |
| 7 | 1 |
| 8 | 2 |
| 9 | 1 |
| 10 | 1 |
| 11 | 1 |
| 12 | 1 |
| 13 | 1 |
| 15 | 1 |
| 17 | 1 |
Now, we will calculate the cumulative frequency:
|------------|----------------|----------------------|
| 2 | 1 | 1 |
| 4 | 1 | 2 |
| 5 | 1 | 3 |
| 6 | 1 | 4 |
| 7 | 1 | 5 |
| 8 | 2 | 7 |
| 9 | 1 | 8 |
| 10 | 1 | 9 |
| 11 | 1 | 10 |
| 12 | 1 | 11 |
| 13 | 1 | 12 |
| 15 | 1 | 13 |
| 17 | 1 | 14 |
To find the median, we need to find n/2:
- Total frequency n=14
- n/2=14/2=7
The formula for mean deviation from the median is:
Mean Deviation=1n∑|Xi−a|⋅Fi
Where |Xi−a| is the absolute deviation from the median.
|------------|----------------|---------|-----------------|
| 2 | 1 | 7 | 7 |
| 4 | 1 | 5 | 5 |
| 5 | 1 | 4 | 4 |
| 6 | 1 | 3 | 3 |
| 7 | 1 | 2 | 2 |
| 8 | 2 | 1 | 2 |
| 9 | 1 | 0 | 0 |
| 10 | 1 | 1 | 1 |
| 11 | 1 | 2 | 2 |
| 12 | 1 | 3 | 3 |
| 13 | 1 | 4 | 4 |
| 15 | 1 | 6 | 6 |
| 17 | 1 | 8 | 8 |
∑Fi⋅|Xi−9|=7+5+4+3+2+2+0+1+2+3+4+6+8=43
Now we can calculate the mean deviation:
Mean Deviation=1n∑Fi⋅|Xi−9|=4314≈3.07
Thus, the mean deviation from the median is approximately 3.07.
Topper's Solved these Questions
Similar Questions
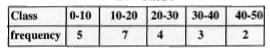
Find the mean deviation from the median for following frequency distribution :
View SolutionCalculate the mean deviation about the median of the following frequency distribution: 5 7 9 11 13 15 17 Having Frequency 2 4 6 8 10 12 8
View SolutionCalculate mean deviation from the median for the following distribution: xi: 10 15 20 25 30 35 40 45 fi: 7 3 8 5 6 8 4 9
View SolutionObtain the median for the following frequency distribution: x : 1 2 3 4 5 6 7 8 9 f : 8 10 11 16 20 25 15 9 6
View SolutionCalculate mean deviation from the median for the following distribution: xi: , 10, 15, 20, 25, 30, 35, 40, 45 fi: , 7, 3, 8, 5, 6, 8, 4, 9
View SolutionFind the mean deviation about the median of the following frequency distribution: Class:, 0-6, 6-12, 12-18, 18-24, 24-30 Frequency:, 8, 10, 12, 9, 5
View SolutionFind the mean deviation about the median of the following frequency distribution: Class:, 0-6, 6-12, 12-18, 18-24, 24-30 Frequency:, 8, 10, 12, 9, 5
View SolutionFind the mean of the following distribution: 4 6 9 10 15 5 10 10 7 8
View SolutionCalculate the mean deviation about the mean for the following frequency distribution Class interval 0−4,4−8,8−12,12−16,16−20 Frequency 4,6,8,5,2
View SolutionFind the mean of the following distribution: x: 4 6 9 10 15 f: 5 10 10 7 8
View Solution
